Proof of the First Fundamental Theorem of Calculus using the Second Fundamental Theorem of Calculus

This video could also be titled "Proof of the Second Fundamental Theorem of Calculus using the First Fundamental Theorem of Calculus". The reason is that many textbooks and online resources swap the names of these theorems, based on which can be used to prove the other. This actually makes good sense.
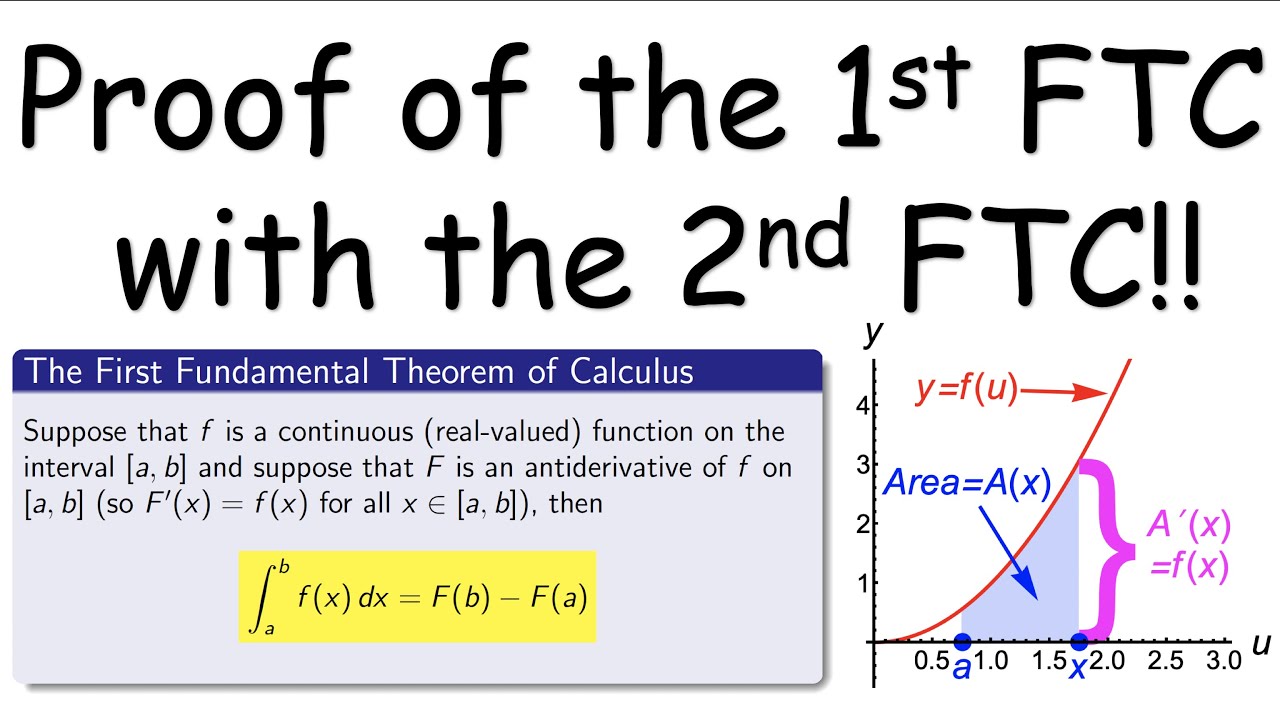
The First Fundamental Theorem of Calculus (1st FTC) can be proved using the Second Fundamental Theorem of Calculus (2nd FTC). The proof is short, though you need to understand the statements and meanings of each theorem. The First Fundamental Theorem tells you how to evaluate definite integrals using antiderivatives. The Second Fundamental Theorem tell you how to differentiate integrals where the upper limit of integration is the variable.

Proof: Assume f(x) is continuous on [a,b] and assume that F(x) is an antiderivative of f(x) on [a,b] (so F'(x) = f(x) for all x in [a,b]). Let A(x) be equal to the definite integral of f(u) as u varies from u = a to u = x. Then the Second Fundamental Theorem of Calculus implies that A'(x) = f(x) = F'(x). Therefore, there is a constant C so that A(x) = F(x) + C for all x in [a,b]. But this implies that the integral of f(x) as x varies from x = a to x = b equals A(b) - 0 = A(b) - A(a) = (F(b)+C)-(F(a)+C)=F(b)-F(a). Q.E.D.

AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.

[billkinneymath]

You should be aware that many textbooks and other resources switch the names of these two theorems around. This actually makes good sense, based on which can be used to prove the other.