filmov
tv
f implies continuous

Показать описание
Every function is continuous! Don't believe it? Then watch this video!
f implies continuous
Continuous implies Bounded
differentiable implies continuous
Proof: Differentiability implies continuity | Derivative rules | AP Calculus AB | Khan Academy
Proof that Differentiable Implies Continuous
Riemann Integration 5: Continuous Implies Riemann Integrable Proof
Differentiable Implies Continuous
3.5 Differentiable implies continuous
Differentiable then Continuous Proof
How to determine if a function is continuous and differentiable
Continuity and Differentiability
Sequential Criteria of continuous function | L2 | TYBSc Maths | Continuous Functions @ranjankhatu
Multivariable Calculus | Differentiable implies continuous.
Proof that a continuous function on a closed interval is uniformly continuous
Differentiable implies continuous
fg is continuous
f+g is continuous
Complex Analysis | Unit 1 | Lecture 38 | Every Differentiable Function is Continuous
Derivatives: Intro - 10. Differentiable Implies Continuous
Riemann Integration | Lecture 21 | Every Continuous Function is Riemann Integrable
3.9 Continuous but not differentiable functions
A Function from X to Y is Continuous iff for every open set V in Y f–1(V) is open in X
is the derivative continuous?
Uniform Convergence of Continuous Functions is Continuous (Proof)
Комментарии
 0:11:40
0:11:40
 0:08:11
0:08:11
 0:05:50
0:05:50
 0:11:39
0:11:39
 0:03:25
0:03:25
 0:12:38
0:12:38
 0:05:40
0:05:40
 0:03:56
0:03:56
 0:03:51
0:03:51
 0:02:21
0:02:21
 0:32:48
0:32:48
 0:10:04
0:10:04
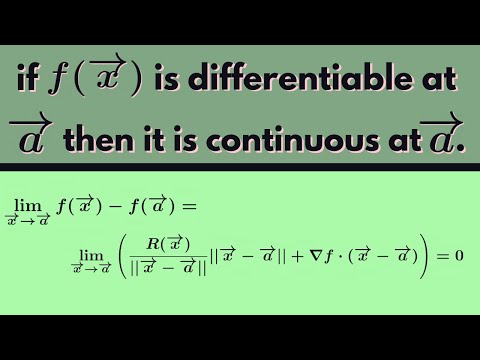 0:14:04
0:14:04
 0:12:00
0:12:00
 0:11:24
0:11:24
 0:11:33
0:11:33
 0:14:13
0:14:13
 0:03:48
0:03:48
 0:08:48
0:08:48
 0:14:38
0:14:38
 0:06:34
0:06:34
 0:12:58
0:12:58
 0:16:00
0:16:00
 0:05:56
0:05:56