fg is continuous

Показать описание

Here I show that the product of two continuous functions is continuous. I do it both by using epsilon-delta and the sequence definition of continuity. Interestingly, the proof is similar to the proof of the product rule for derivatives. Enjoy!

Dr Peyam

Рекомендации по теме

Комментарии

Thanks must go to Dr .Peyam for the eloquent proof in his great video ❤

wtt

Amazing proof! Great job, from a mathematics major in Canada.

Jekku

The video sound is pretty good, beyond my imagination

trangngoc

Is this a real analysis lesson, due to involving the sequence definition of continuity?

mike_the_tutor

Hi dr. Peyam! Thanks for the class!
A doubt:
if I had chosen the ε of the δ3 as ε/(2*|g(xo)|), could I separate this exercise it into two cases, one where g is different from 0 and another where g is equal to 0 ?
Thanks for your attention!

mariosergiomadurosantana

Do f and g have to have the same domain for this proof to apply?

Shaan_Suri

Dr sir, please suggest a textbook to read along with 😢

meshachistifanus

and the proof I spent two hours trying to figure out is done in 11 minutes

jewelleharper

If f(x):R to R is continuous, and
g, h:R to R, g(x)=f(x+t) and h(x)=f(tx)
[ t€R+ ]. Then, are g(x) and h(x) continuous or uniformly continuous or discontinuous?

SR-kdwi

Tbh I dislike that during proofs people "choose ε=1". Literary ε is a small number "given by the enemy", as my teacher would say...

skipii

fg is continuous

fg is continuous

f/g is continuous

Continuity 2 || Algebra of Continuous function || Real analysis || maths fun || Diyash kumar 🔥

prove that f(x) and g(x) are continuous then f g (x) is also Continuous||

THEOREM 21.5 | F AND G IS CONTINUOUS THEN F+G,F-G,F.G,F/G IS CONTINUOUS

If `f.g` is continuous at `x = 0` , then f and g are separately continuous at `x = 0`.

If f and g are both continuous at x = a, then f-g is | 12 | CONTINUITY | MATHS | TARGET PUBLICA...

continuous running FG film - Philip Wandor

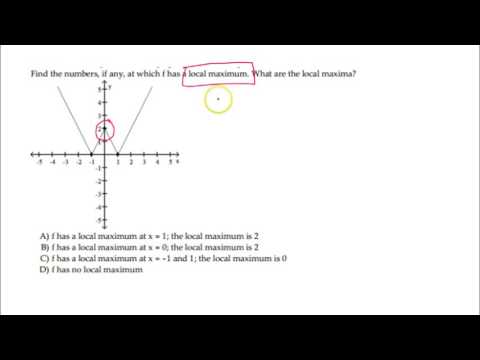
Max and Min FG

fg is uniformly continuous on s if s is compact | mth621 vu

Vinnie Patteri Continuous FG Center

Vinnie Patteri Continuous FG Hashes

Continuous fg from 33-50

Continuous fg film

FGIX (Continuous DJ Mix)

If F and g are measurable functions then F+g and F-g are measurable, Mathematics/M.SC/PG/semester-2

İspat f ve g sürekli fonksiyonlarsa f-g nin sürekli oldugunun ispatı

Epsilon Delta Continuity (Example 4): x^3

Lec-24 V f g is less or equal AV f +BV g

Taylor Sheets FG Practice (Continuous)

Matt Mengel UCLA k/p continuous fg film

Continuous function

FG Commits Double Offense with Nnamdi Kanu’s Continuous Detention - Ejimakor

Jamie Sutcliffe - Continuous FG kicks ....Practice Film