3-Body Problem Lagrangian Equations and Effective Potential Energy | Topic 4

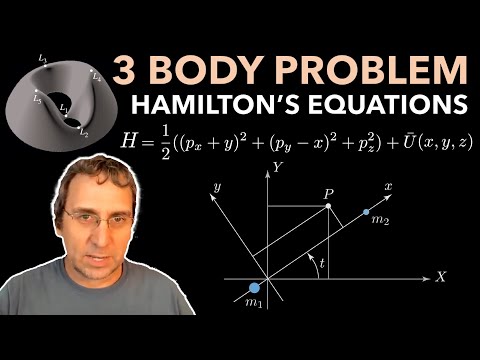
Using the Lagrangian formulation, the equations of motion are derived for a spacecraft in the Circular Restricted Three Body Problem (CR3BP) viewed in the rotating frame. This approach is more elegant and requires fewer steps and less algebra. One can simply write the total kinetic energy minus the total potential energy, L = K - U, using rotating frame coordinates. Lagrange's equations (also known as the Euler-Lagrange equations) then give the correct equations of motion.

❌CORRECTION at 9:43 - term at the end should be +Ū (NOT -2Ū)

The effective potential energy (also called the augmented potential) is a way to include both the effects of gravity and the centrifugal force of the rotating frame. The critical points of the effective potential energy function are the Lagrange points of balance (or equilibrium).

The restricted 3-body problem describes the motion of a body moving in the gravitational field of two primaries that are orbiting in a circle about their common center of mass, with trajectories such as Lagrange points, halo orbits, Lyapunov planar orbits, quasi-periodic orbits, quasi-halos, low-energy trajectories, etc.
• The two primaries could be the Earth and Moon, the Sun and Earth, the Sun and Jupiter, etc.
• The equations of motion of derived in the inertial frame centered at the primaries' center of mass.
• The equations have been non-dimensionalized
• The mass parameter μ is the only factor determining the type of motion possible for the spacecraft. It is analogous to the Reynold's number Re in fluid mechanics, as it determines the onset of new types of behavior.

▶️ Next:3-Body Problem Hamilton's Equations -&- Why View Motion in a Rotating Frame?

▶️ Previous: 3-Body Problem Equations Derived, Part 2: Rotating Frame

▶️ In Case you Missed It: 3-Body Problem Introduction

▶️ Related: Lagrange's Equations Introduction | Generalized Coordinates, Constraints, Degrees of Freedom

► FREE Book on the Patched 3-Body Method:
Dynamical Systems, the Three-Body Problem and Space Mission Design. Koon, Lo, Marsden, Ross (2011)
We are Chapter 2.

► PDF Lecture Notes (Lecture 2 for this video)

► Dr. Shane Ross is an Aerospace Engineering Professor at Virginia Tech. He has a Ph.D. from Caltech (California Institute of Technology) and worked at NASA/JPL and Boeing.

► Other Related Videos
▶️ Applications to Dynamical Astronomy

► Related Courses and Series Playlists by Dr. Ross

📚3-Body Problem Orbital Dynamics Course

📚Space Manifolds

📚Space Vehicle Dynamics

📚Lagrangian and 3D Rigid Body Dynamics

📚Nonlinear Dynamics and Chaos

📚Hamiltonian Dynamics

📚Center Manifolds, Normal Forms, and Bifurcations

#orbitalmechanics #LagrangePoint #rotatingframe #threebodyproblem #CR3BP #space #3body #3bodyproblem #mathematics #SpaceManifolds #JamesWebb #NonlinearDynamics #gravity #SpaceTravel #SpaceManifold #DynamicalSystems #JamesWebbSpaceTelescope #space #solarSystem #NASA #dynamics #celestial #SpaceSuperhighway #InterplanetarySuperhighway #spaceHighway #spaceHighway #gravitational #mathematics #dynamicalAstronomy #astronomy #wormhole #physics #chaos #unstable #PeriodicOrbits #SaddlePoint #CriticalPoint #Halo #HaloOrbit #LibrationPoint #LagrangianPoint #LowEnergy #VirginiaTech #Caltech #JPL #LyapunovOrbit #CelestialMechanics #HamiltonianDynamics #planets #moons #multibody #GatewayStation #LunarGateway #L1gateway #ArchesOfChaos #cislunar #cislunarspace #orbitalDynamics #orbitalMechanics #celestialChaos #Chaotician #Boeing #JetPropulsionLab #Centaurs #Asteroids #Comets #TrojanAsteroid #Jupiter #JupiterFamily #JupiterFamilyComets #Hildas #quasiHildas #KuiperBelt

[mohammadmundiwala]

i feel very lucky to have found your content. great lectures

[stefanonovelli]

Thank you so much, prof Ross.It was exciting and so clear.And now the lagrangian approach: another adventure. By.

[roros]

1:14 -(1-mu)mu/2 thank you for this explanation, I couldn't find it anywhere else, thanks again