The Two Body Problem (Newton, Kepler) | Fundamentals of Orbital Mechanics 1

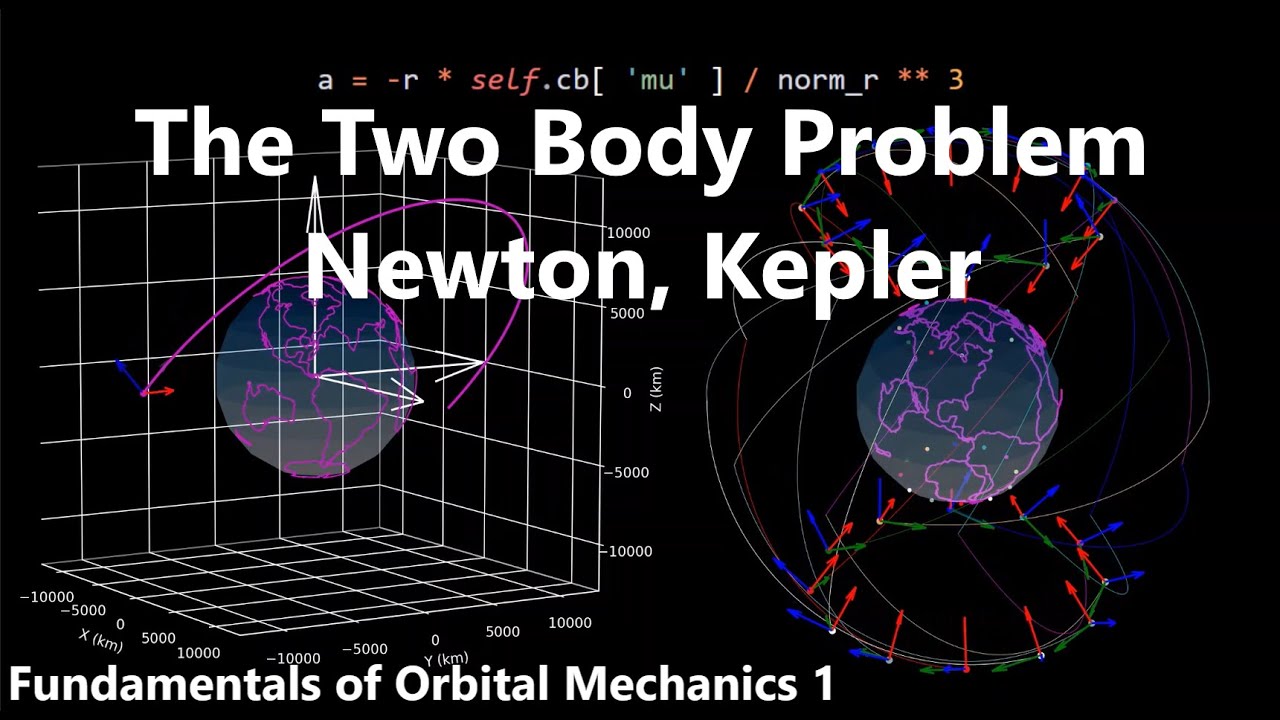
This video covers the two body assumptions, Newton's universal law of gravitation, Newton's 1st law, and Kepler's first law, to explain the two body problem of orbital motion.

Instagram and TikTok: @spaceengineeringpodcast

The two body problem assumes:
1. All that exists in the universe is one large body (like the Earth) and one small body (like a spacecraft)
2. The large body is spherical and its mass is evenly distributed, which means that its gravitational pull can be modeled as coming from its geometric center
3. The small body does not influence the large body gravitationally
4. We use an inertial frame centered at the geometric center of the large body
Using these 4 assumptions, we arrive at Newton's Universal Law of Gravitation, which states that the force due to gravity on the small body is equal to the mass of the large body times the mass of the small body times the gravitational constant in our universe divided by the distance between them squared

However, we are interested in the acceleration of the small body (not the gravitational force), because we use acceleration to simulate the motion of orbiting bodies. We can solve for the acceleration using Newton's first law (F=ma). This acceleration equation is a differential equation, because we want to calculate the position of the small body over time, and the acceleration is the second derivative of position.

In order to create 3D simulations of orbits, we need to extend that equation into 3D using vectors. So we turn the radial distance into a vector, which is the vector pointing from the center of the large body to the small body. We also need to add a negative sign, because the acceleration due to gravity is pointing in the exact opposite direction from the position of the small body. And this 3D equation is exactly how we plug it into software to create these simulations.

Links to the Space Engineering Podcast (YouTube, Spotify, Google Podcasts, SimpleCast):

Link to Mecánica Orbital con Python (videos en Español):

Link to old video on the Two Body Problem and Ordinary Differential Equation (ODE) Solvers:

#twobodyproblem #orbitalmechanics #astrodynamics

[omarazami]

You deserve some sort of is incredibly non-trivial and insightful and i think more people should rise to the level of beyond pop science. I really have a lot of respect for you.

[jimmycricket]

One of the best physics teachers I ever encountered was a geographer who told me he hated physics and had been drafted in to cover for the physics lecturer who was sick that day. He must have been a whizz at geography.

[pancakesnchill]

This has been super helpful, I'm currently learning orbital mechanics and python without any college courses through an internship and this has helped break it down so well for me. I was able to compare it directly to my notes and follow along and then play around with my own notebooks in python to see how it worked. Thank you for all the helpful info!

[CentauriBros]

Aaaagh. Thank you!! As a 8th grader who wants to be an aerospace engineer, astrophysicist and astronaut this was sooo helpful!!

[ashokdarbhe]

thank you for the detailed explanation

[DavidRabanus]

I think that the 3D display of earth with the sat's orbit (see 1:18) is not quite right. I think that the center of the earth should be in one of the ellipse's foci and not at the center of the ellipse.

[SSran-ivlu]

Judging by the Constellation gif, I think your code is pretty well optimized. As soon as I have more than 5 orbits, it starts to lag, especially when I change the view angle in Matplotlib 3D.
Does yours glitch/lag when you use the mouse to change the view angle?
Also, great video. I much more intuitively understand what is going on in the differential equation function now. A great addition to the series!

[astronomyflare]

Beautiful explanation. Please make some videos on celestial mechanics.

[christopherhorton]

Is the code going to be uploaded on GitHub? Would love to also see the adcs portion!

[comicrelief]

Do you have a video on the Principle of Least Action?

[MattyAtoms]

Does any body truly have a perfectly evenly distributed mass?

[videomaniaXX]

Nice video as always. Yours videos have been really helpful. I have a question not related to the topic of this specific video but I don't know where to ask. I have used matplotlib animation and FuncAnimation to generate gifs. At the moment I am stuck with the quivers. I used set_segments but it does not seem to work. However the same principle works just fine for the trajectories. Any advice on this, please. Thanks

[ankitaaarya]

Holy shiyy this is some advance level stuff i stumbled upon, alien technology, ufo antigravity vibes.

[thebiber]

What you call Newton's first Law is actually Newton's second law according to his Principia. It is conventional to teach it this way.

[aitorramirez]

Great video! I am interested on knowing how you implemented the Earth coastlines. Did you use shape files or is it some python library? Thank you :)

[nicholaskryger-nelson]

F=ma is Newton's second law (certainly his most memorable but it is his second)