filmov
tv
Banach Fixed Point Theorem

Показать описание
In this video, I prove the celebrated Banach fixed point theorem, which says that in a complete metric space, a contraction must have a fixed point. The proof is quite elegant and illustrates the beauty of analysis. This theorem is used for example to show that ODE have unique solutions under certain conditions. Enjoy!
Banach Fixed Point Theorem
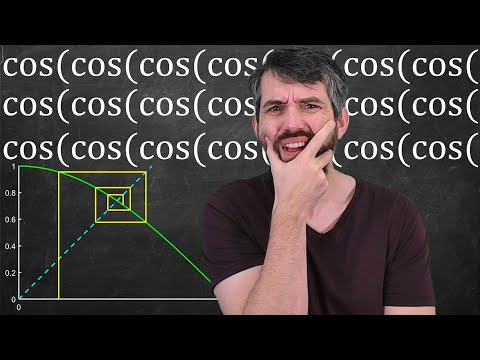
What is cos( cos( cos( cos( cos( cos( cos( cos( cos( cos( cos( cos(…?? // Banach Fixed Point Theorem...
Banach Fixed Point Theorem
16.1 The Banach fixed point theorem
Fixed Points
Banach Fixed Point Theorem
Fixed Point Iteration: Examples, Analysis, and the Banach Fixed Point Theorem
Proving Banach Fixed Point Theorem (Contraction Principle) in 3 Minutes
Banach fixed-point theorem MADNESS
11. Banach's Fixed Point Theorem || Contraction Mapping || Fixed Point || in hindi | Ravina Tut...
Contraction Mapping Theorem & Finding Fixed Points of Functions
Introduction to Banach Fixed Point Theorem
Math | Metric Spaces | Banach Fixed Point Theorem | Lect.15 | Dr. S.S.Bellale | DSCL
The Banach contraction mapping theorem - Oxford Mathematics 1st Year Student Lecture
Banach fixed point theorem in hindi // fixed point Theorem //
Prove Banach's Fixed Point Theorem in 4 min 30 sec
Banach fixed point theorem in English (part-4)
Example of Banach fixed point theorem
Banach's fixed point theorem||Complete Proof In Hindi ||
Banach fixed-point theorem
What is a fixed point?
Fixed Point Iteration System of Equations with Banach
Banach fixed point -2
13.1. The Banach fixed point theorem
Комментарии
 0:11:00
0:11:00
 0:09:11
0:09:11
 0:20:50
0:20:50
 0:13:59
0:13:59
 0:16:26
0:16:26
 0:25:52
0:25:52
 0:06:32
0:06:32
 0:03:00
0:03:00
 0:02:00
0:02:00
 0:37:05
0:37:05
 0:17:05
0:17:05
 0:04:04
0:04:04
 0:56:02
0:56:02
 0:55:17
0:55:17
 0:17:34
0:17:34
 0:04:32
0:04:32
 0:11:15
0:11:15
 0:04:12
0:04:12
 0:14:33
0:14:33
 0:08:46
0:08:46
 0:05:16
0:05:16
 0:11:10
0:11:10
 0:07:19
0:07:19
 0:44:05
0:44:05