Marcus Michelen (UIC): Roots of random polynomials near the unit circle

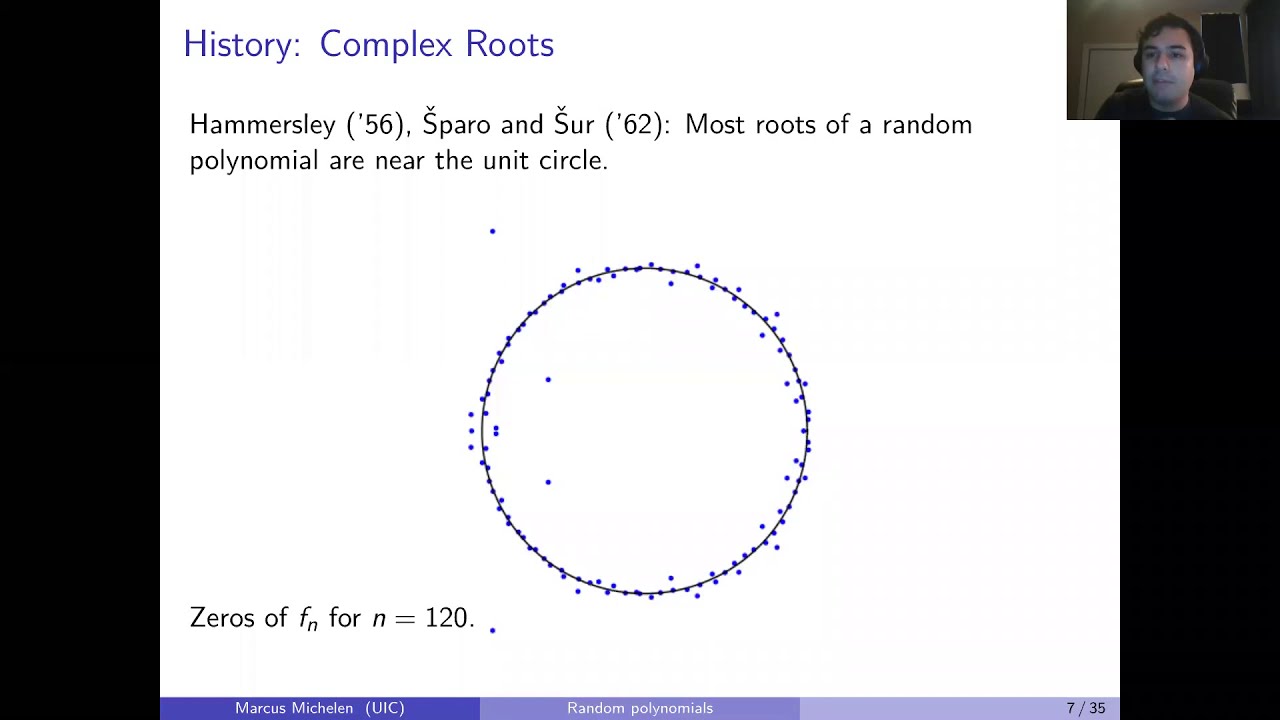
Abstract: It is a well-known (but perhaps surprising) fact that a polynomial with independent random coefficients has most of its roots very close to the unit circle. Using a probabilistic perspective, we understand the behavior of roots of random polynomials exceptionally close to the unit circle and prove several limit theorems; these results resolve several conjectures of Shepp and Vanderbei. We will also discuss how our techniques provide a heuristic, probabilistic explanation for why random polynomials tend to have most roots near the unit circle. Based on joint work with Julian Sahasrabudhe.