Sam Chow (Warwick): Galois groups of random polynomials

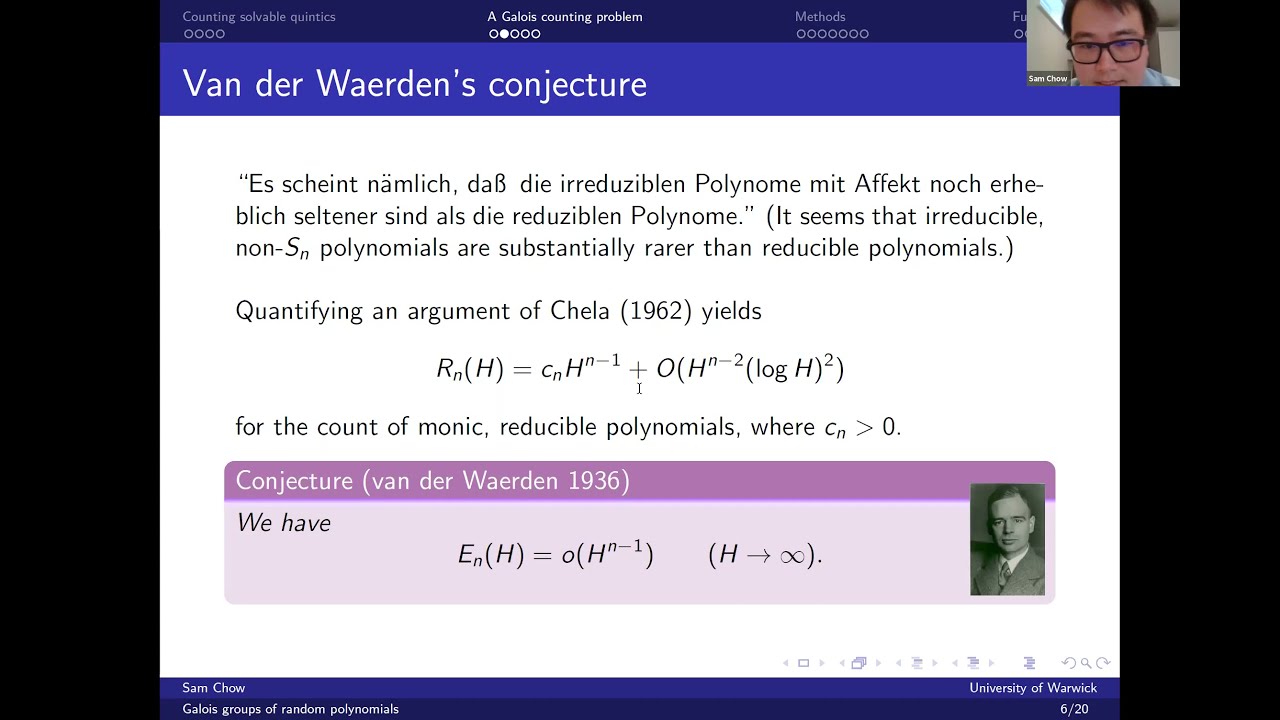
How often is a quintic polynomial solvable by radicals? We establish that the number of such polynomials, monic and irreducible with integer coefficients in [-H, H], is O(H^3.91). More generally, we show that if $n \ge 3$ and $n \neq 7, 8, 10$ then there are O(H^(n-1.017) monic, irreducible polynomials of degree n with integer coefficients in [-H, H] and Galois group not containing A_n. Save for the alternating group and degrees 7, 8, 10, this establishes a 1936 conjecture of van der Waerden, that irreducible non-S_n polynomials are substantially rarer than reducible polynomials.