Direct Math Proof: If n is odd then 3n + 7 is even

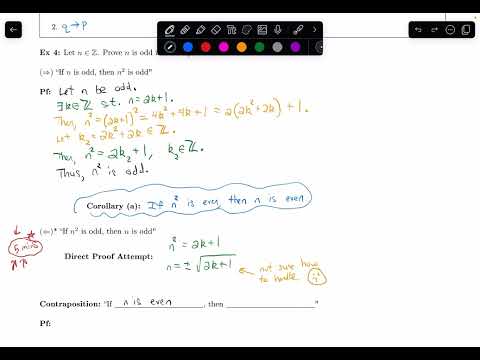
In this video I prove that if n is an odd integer then 3n + 7 is an even integer. This is a good proof for learning proof structure.

(the above links are my affiliate links)

If you enjoyed this video please consider liking, sharing, and subscribing.

There are several ways that you can help support my channel:)

************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course

Advanced Calculus Course

Calculus 1 Course

Calculus 2 Course

Calculus 3 Course

Calculus 1 Lectures with Assignments and a Final Exam

Calculus Integration Insanity

Differential Equations Course

Differential Equations Lectures Course (Includes Assignments + Final Exam)

College Algebra Course

How to Write Proofs with Sets Course

How to Write Proofs with Functions Course

Trigonometry 1 Course

Trigonometry 2 Course

Statistics with StatCrunch Course

Math Graduate Programs, Applying, Advice, Motivation

Daily Devotionals for Motivation with The Math Sorcerer

Thank you:)

[idkjustleavemebeplease]

I genuinely feel like if I had a hand book that explained notation and the proper rules, I'd be able to fully calculate anything.

[Acid]

when you add odd number, it flips result:
n is odd
n+n is even
n+n+n is odd
n+n+n+7 is even

[Dottie]

i wonder how knowing things like this would change life ..
(had to drop out of school at age 14.. no clue what maths is all about - other then simple 1+1=2 and some light fractions im pretty much lost .. but i wonder if i had been able to go to school and actually study and know things lke this if it would have changed my life, .. okay tis sounds crazy .. but i think all knowledge we have shapes who/how we are, so it changes our perspectives on things .. okay im babbling now .. time for a movie and pringles!
youtube send me on a rabbithole w vids that make me go "hmm "

(ps u got a nice voice sir)

[joeytaft]

When you write the recall statements why do say some integers instead of all integers?

[thetheoreticalnerd]

Math sorcerer is there any way I could send you the download for the Calc 2 exam? When I put it in a YouTube comment it gets deleted

[gilbert]

I have never taken a math foundations class so this may be completely wrong. But here is what I got and I'm curious to see how close it was (haven't watched the video yet).

n odd
m=n-1
m even
n=m+1

substitute for n:
3n + 7 = 3(m+1) + 7
distribute:
=3m + 3 + 7
add 3 and 7:
=3m + 10
take out a 2:
=2(1.5m +

1.5m = 1m + 0.5m
but m is even, so by definition, 0.5m is an integer p
--> 1.5m = 1m + 0.5m = m + p

(1) becomes:
2(m+p+5)
m, p, and 5 are all integers, so m+p+5 is also an integer q
so we end up with:
2*q where q is an integer
2*integer = even number
QED?

Criticize please

[readyfireaim]

Humanity has ten numbers (0, 1, 2, 3, ...9)

Newton:
"0 is contingent" 🚫
and
"1-9 are necessary" 🚫
(this is the basis of Newton Calculus/Physics/Logic).

Leibniz:
"0 is necessary" ✅
and
"1-9 are contingent" ✅
(this is the basis of Leibniz Calculus/Physics/Logic).

Is zero the most important number?

Zero is the most important number in mathematics. Zero functions as a placeholder. Imagine a number, e.g., 5 and put as many zeroes behind it as you can think of. Zero drastically changes the value of the number from a mere 5 to 50, 500, 5000, 50000 and beyond.

Which is the greatest whole number?

There is no 'largest' whole number. Every whole number has an immediate predecessor, except 0. A decimal number or a fraction that falls between two whole numbers is not a whole number.

Why is it impossible to divide by zero?

The short answer is that 0 has no multiplicative inverse, and any attempt to define a real number as the multiplicative inverse of 0 would result in the contradiction 0 = 1.

Is 0 a rational number?

Yes, 0 is a rational number. Since we know, a rational number can be expressed as p/q, where p and q are integers and q is not equal to zero. Thus, we can express 0 as p/q, where p is equal to zero and q is an integer.

Is 0 A whole number?

The whole numbers are the numbers 0, 1, 2, 3, 4, and so on (the natural numbers and zero). Negative numbers are not considered "whole numbers." All natural numbers are whole numbers, but not all whole numbers are natural numbers since zero is a whole number but not a natural number.

Why is 0 a good number?

Zero helps us understand that we can use math to think about things that have no counterpart in a physical lived experience; imaginary numbers don't exist but are crucial to understanding electrical systems.
Zero also helps us understand its antithesis, infinity, in all of its extreme weirdness. 🔘 ♾ ☯️

Who is pushing Newtonian Calculus/Physics/Logic? The man was a moron (and a fraud who used political power to "win").

Our fundamentals are off because our fundamentals are directly "idiot" Issac Newton's fundamentals.

Our universe doesn't match Newton's illogical nonsense.

Theory of Everything is Cosmogony, Cosmology and Quantum and
Newton is buttcheeks at all three.

Nothing lines up.

Hard swap to Gottfried Leibniz.

Also, Aether > Gravity.
(Miller crushed Gravity so hard they had to wait more than a decade after his death to start weasling Newtonian logic a "win" again by burying Miller’s Aether theory).

Also, Tesla > Edison.
(3D height, 6D depth,
9D absorption i.e. contingent universe)

fundamental = rock
particular = sand

[rishikkeshari]

If n is odd then 3n is odd. And 3n +7 is sum of two odd numbers therefore it's even.

[jkid]

I don't know what level this is intended for, but might as well mention, you have made implicit use of the distributive property, and more deviously, that arbitrary sums and products of integers remain integers (given integer k, 3k+5 is an integer). But again, I don't have the full context, and maybe you have already proven some things about the integers as a field, but even if you did, you certainly didn't invoke it here in this short. Usually an important point of these low-level proofs is to really scrape the bottom, to see, like, "oh wow I actually can't get there without assuming I'm in a field, I wonder if this only holds in fields", etc. Just assuming intuitive properties of arithmetic is like, if we were allowed to do this, I would assume the whole statement to be proven :P

[kannix]

i mean odd times odd plus odd is even duh, why not just prove something more general?

[wanzaly]

❤Only for fans over 18 year⤵️ Alles sehr schön. Aber zuerst zusammen die Nummern 10 und 1. Eine Babymomm.beauty Brünette und eine andere Blondine. Es wäre unfair, wennu ich 4 wählen würde

[richard_darwin]

if n is odd, 3n will always be odd (proof: basic intuition ie. 2n+n = even + odd = odd) => 3n+7 = odd + odd = even always. it's pretty fucking basic why even make a video about it lmao