filmov
tv
Sum of Integers Squared: Math Direct Proof Example
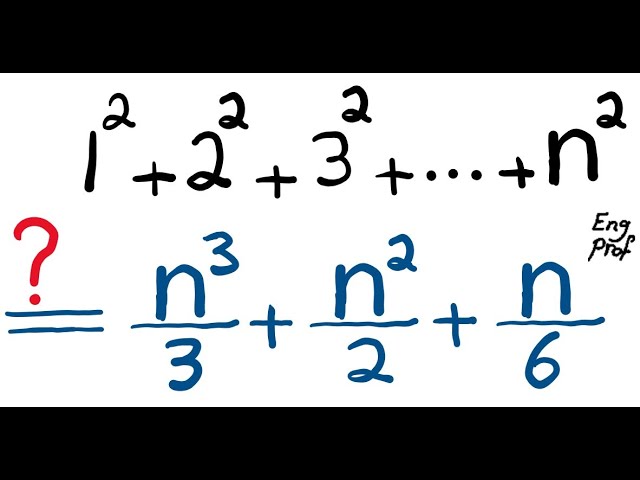
Показать описание
A Direct Proof Example is presented in this video to prove the closed form formula for the sum of the squares of positive integers from 1 to n.
In logic and mathematics, a direct proof is a method of proving statements of the form p ⇒ q ”if p then q” that takes an original hypothesis or assumption p, which we assume to be true, and use it to show directly that the desired conclusion q is true.
In logic and mathematics, a direct proof is a method of proving statements of the form p ⇒ q ”if p then q” that takes an original hypothesis or assumption p, which we assume to be true, and use it to show directly that the desired conclusion q is true.
Numberphile v. Math: the truth about 1+2+3+...=-1/12
Math Antics - Adding & Subtracting Integers
What does it feel like to invent math?
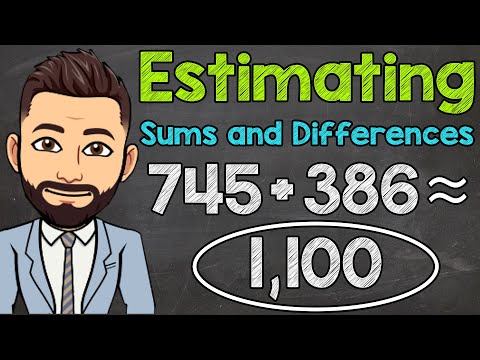
Estimating Whole Number Sums & Differences | Addition & Subtraction Estimation | Math with M...
How to Create a Magic Square With Negative Numbers : College Math
ROUNDING TO ESTIMATE THE SUM / 3rd Grade COMMON CORE MATH
What's an Integer? | Integers Explained | Math with Mr. J
What are Square Roots? | Math with Mr. J
Math 1 Week 5 Day 2 Quarter 2 Add 1 digit and 2 digit numbers multiples of 10 with sums up to 100 in
Learn to Square any 2 digit number I Math Tricks and Tips
Basic Math Addition For Kids | Noodle Kidz Pre-K and Kindergarten Educational Video
Estimating Sum For Kids (Math) | TutWay
Grade 2 Math: Addition Solution
Math Antics - Multi-Digit Addition
Adding Integers Using a Number Line | Math with Mr. J
Adding & Subtracting! | Mini Math Movies | Scratch Garden
Adding Number (Up to 20) - 1st Grade Math (1.OA.2)
Math Antics - Exponents and Square Roots
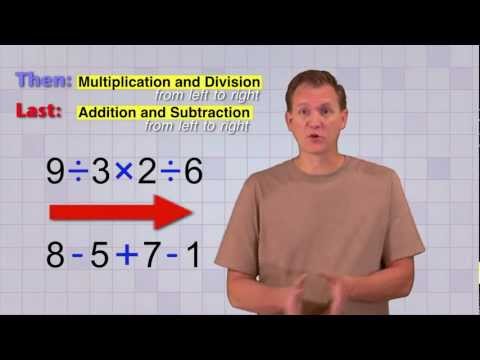
Math Antics - Order Of Operations
The Strange Branch of Math Where Infinity is Tiny
Learn Addition Using Number Line | Mathematics Book B | Periwinkle
Adding 2-Digit Numbers With Regrouping | Double-Digit Addition | Elementary Math with Mr. J
Square Roots and Cube Roots | Math with Mr. J
Grade 2 Math: Subtraction Rocks with Base Ten Blocks
Комментарии
 0:41:44
0:41:44
 0:11:23
0:11:23
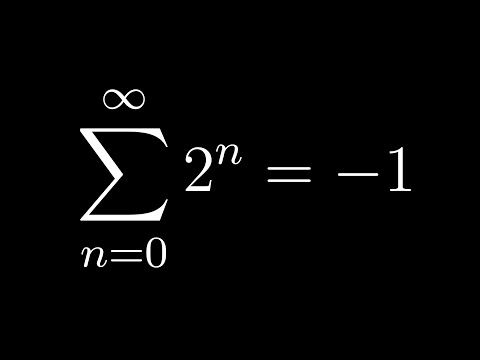 0:15:08
0:15:08
 0:07:38
0:07:38
 0:03:05
0:03:05
 0:08:22
0:08:22
 0:02:22
0:02:22
 0:04:00
0:04:00
 0:08:21
0:08:21
 0:07:10
0:07:10
 0:07:01
0:07:01
 0:06:44
0:06:44
 0:01:10
0:01:10
 0:06:29
0:06:29
 0:04:09
0:04:09
 0:07:27
0:07:27
 0:04:03
0:04:03
 0:11:09
0:11:09
 0:09:40
0:09:40
 0:33:06
0:33:06
 0:01:03
0:01:03
 0:07:59
0:07:59
 0:05:03
0:05:03
 0:02:27
0:02:27