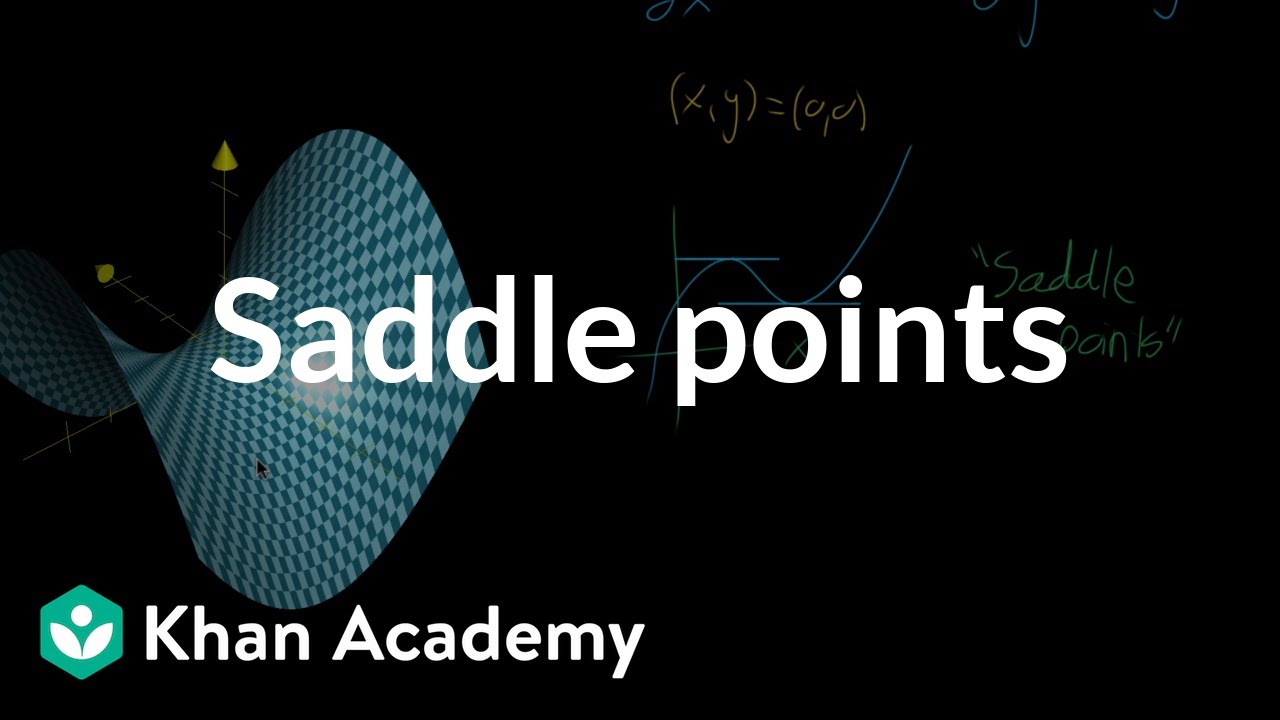
Saddle points

Just because the tangent plane to a multivariable function is flat, it doesn't mean that point is a local minimum or a local maximum. There is a third possibility, new to multivariable calculus, called a "saddle point".

[rachealmolzahn]

I think it would be better if it was called pringle...

[ricardovaras]

This is just gold for me, I promise that when I get a job and (some) money I'll give it all (well, maybe not all) to Khan Academy, it's not just that you're helping me on College, you're also motivating me to follow some sort of a dream that I have. I'm so thankful with these and other content creators who want to share it's knowledge to everyone who wants to learn.

(Also, sorry if I have errors in my grammar, non native speaker)

[zairaner]

The statement "If the derivative is zero in a point in singlevariable calculus, it is either a minimum or a maximum" is obviously false because it could happen that the second derivative is also zero -like the point 0 for x^3

[damian.gamlath]

I wish Grant could go through and refresh all of Khan's older videos - Grant's stuff is just simply brilliant.

[sankaracharyadutta]

Hey Grant !! Regarding your statement of Saddle points is a new concept in Multi -variable calc and the example about the single variable calculus ---
In single Variable Calculus there exists a point called the POINT OF INFLECTION where the tangent has zero slope but it is neither a maxima nor a minima.. INFLECTION points are similar to saddle points because in such points the neighborhoods have different tendencies just like the fact that the partial derivatives have different tendencies here. So please Refer.

But by the way you are doing a fantastic job by making the viewers really understand the topics through real 3d graphs... THANKS!! lots of love...

[zyzhang]

For one variable case it is actually possible for a tangent plane to be flat for neither maximum nor minimum (inflection) 4:36

[robertcharmers]

As ever, an adequately comprehensive and clear exposition of a relatively involved topic. Many Thanks.

[ozboomer_au]

As an engineer, I find this sort of mathematics makes me *grin* (and bends my brain)... particularly when you look in terms of data structures in (computer) programming. In 2D (a "table" array), the X-variable and the f() (or Z) can have 'obvious' maximum and minimum values... in 3D (a "cube" array of 3 items), both the X-variable AND the Y-variable can be a maximum AND/OR a minimum... but you only realize this because you can see what's going on from a 3D viewpoint. What happens when we start thinking in 4D and beyond!? Time to re-visit 'Flatland' by Edwin A Abbott...

[giantneuralnetwork]

Always heard of saddle points... A local min and max! It's like you're close to a local max and yet so far away. Darn saddle points...! These vids are great, thanks for making them.

[venjaminschuster]

Khan academy is my new Netflix! Love it! Thank you very very much!

[pg]

Solomon Khan I owe you my life
I kept trying to visualize and draw what a saddle point would look like today in class with no luck. The 3D graphics really help

[pavanpatel]

what is the software, you are using for the graph?

[wildertapiasaenz]

These graphics are awesome and help so much conceptually, thank you! 🙏

[tahoon]

That's a beautiful visualization, thanks :)

[Persian]

awesome what a beautiful graph It makes it easy to understand.

[iwtwb]

For everyone commenting about whether saddle points are new concepts or not- I see what you mean but I think he is talking about cases were where the second derivative is not zero. IE, the function he is displaying has a negative second derivative with respect to y, but a positive second derivative with respect to x. That is the new concept.

[clapathy]

such a clear description of the saddle point

[sindivcx]

Great explanation! May i ask you what program you are using?

[miguelmendoza]

What software do you use to do this???

[shreyasarkar]

How are plateaus treated in maxima and minima? When are they considered an extrema point?