What Is And How To Find, Calculate Mean Absolute Deviation - Mean Absolute Deviation Explained

Показать описание

In this video we discuss what is absolute deviation and explain how to calculate absolute deviation. We go through an example comparing the absolute deviations of 2 similar items.

Transcript/notes
What is absolute deviation? Absolute deviation, which is often referred to as the mean absolute deviation, is a statistic that tells us how much a data set varies around the mean. Technically it tells us the average distance each data point is from the mean.

As a quick example, if you are looking to purchase new tires for your vehicle, there are 2 different brands of tires that cost the same and they both have an average life of 22,000 miles. Which one should you purchase? Well, the absolute deviation can help. If brand A has an absolute deviation of 725 miles, and brand B has an absolute deviation of 980 miles, this means that the average life of brand A has less deviation from the mean of 22,000 miles, so based on this statistic, they would be a better purchase.

As an example, here are 2 data sets for the playtime in hours, per full charge for 2 models, model A and model B of earbuds. 6 different pairs of each were tested, and the average playtime for both pairs was 36 hours.
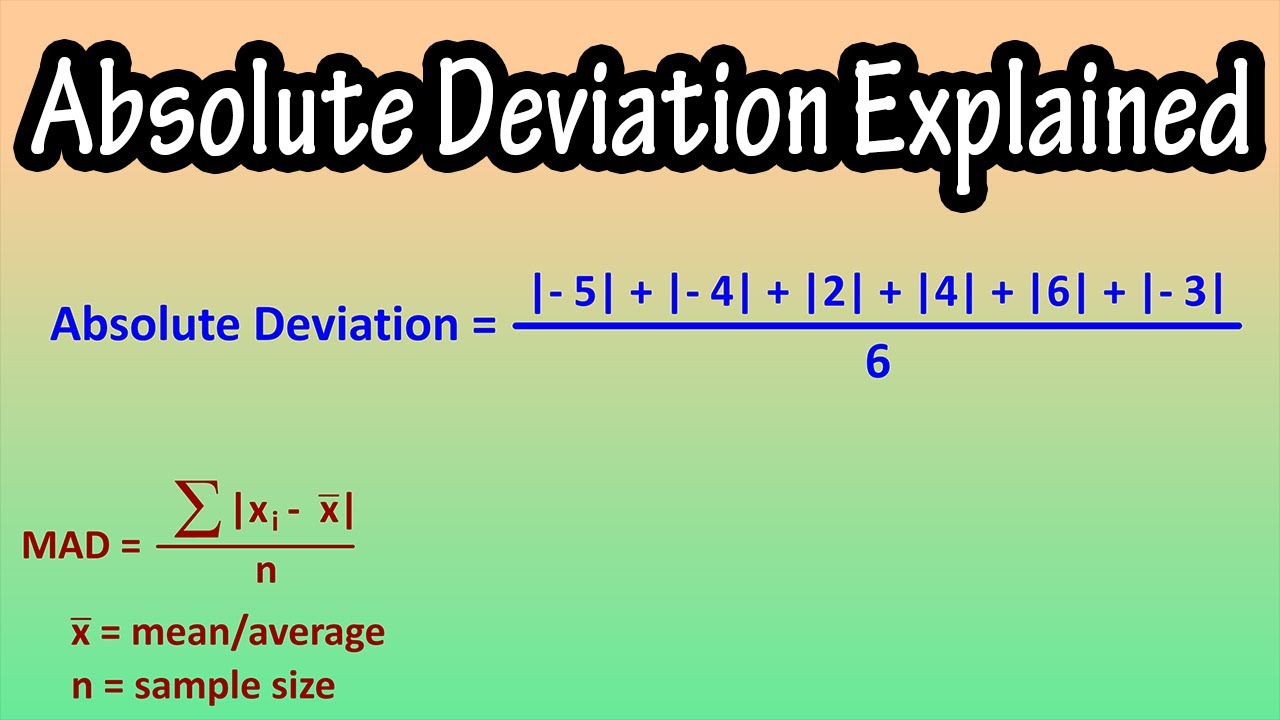
Next, we are going to calculate their absolute deviations and compare them. The way we do this is, we take the absolute value of each data point minus the mean, then we add them together, and then we divide by the total number of data points. So, for model A, we take the absolute value of pair 1 minus the average, plus the absolute value of pair 2 minus the average, plus the absolute value of pair 3 minus the average, and we do this for all 6 pairs, and then we divide that number by 6, the total number of pairs.

One note, for any math nerds like me, the formula for absolute deviation is the sum of the absolute value of x sub i minus x bar, over n, where x bar is the mean and n is the sample size.

I have done this on the screen, absolute value of 31 minus 36 for pair 1, plus, absolute value of 32 minus 36 for pair 2, and so on. Next, we get all positive values since we are taking the absolute value, and we end up getting 24 divided by 6, which equals 4. So, the absolute deviation for model A is 4 hours.

Here is the same process for model B, which gives us an absolute deviation of 4.67 hours rounded off.

With model A being 4 hours and model B being 4.67 hours, this tells us that the model A earbuds average playtime per charge are more consistent of having an average playtime of 36 hours. So, based on this statistic alone, they would be the better purchase.

This is an extremely small sample size, so you would also have to take that into consideration.

Here is a dot plot graph to show you this visually. Here is the average for both models, and you can see that model A is more consistent around the average and model B is much more spread out.

Timestamps
0:00 What is absolute deviation?
0:16 Quick example of absolute deviation
0:48 Detailed example of absolute deviation
1:07 How to calculate absolute deviation
1:37 Mathematical formula for absolute deviation
2:21 Comparing absolute deviations
2:43 Visual dot plot