Alexey Beshenov | Weil-étale cohomology of arithmetic schemes

Seminar on A1-topology, motives and K-theory, May 15, 2021
Alexey Beshenov (CIMAT)
Weil-étale cohomology of arithmetic schemes

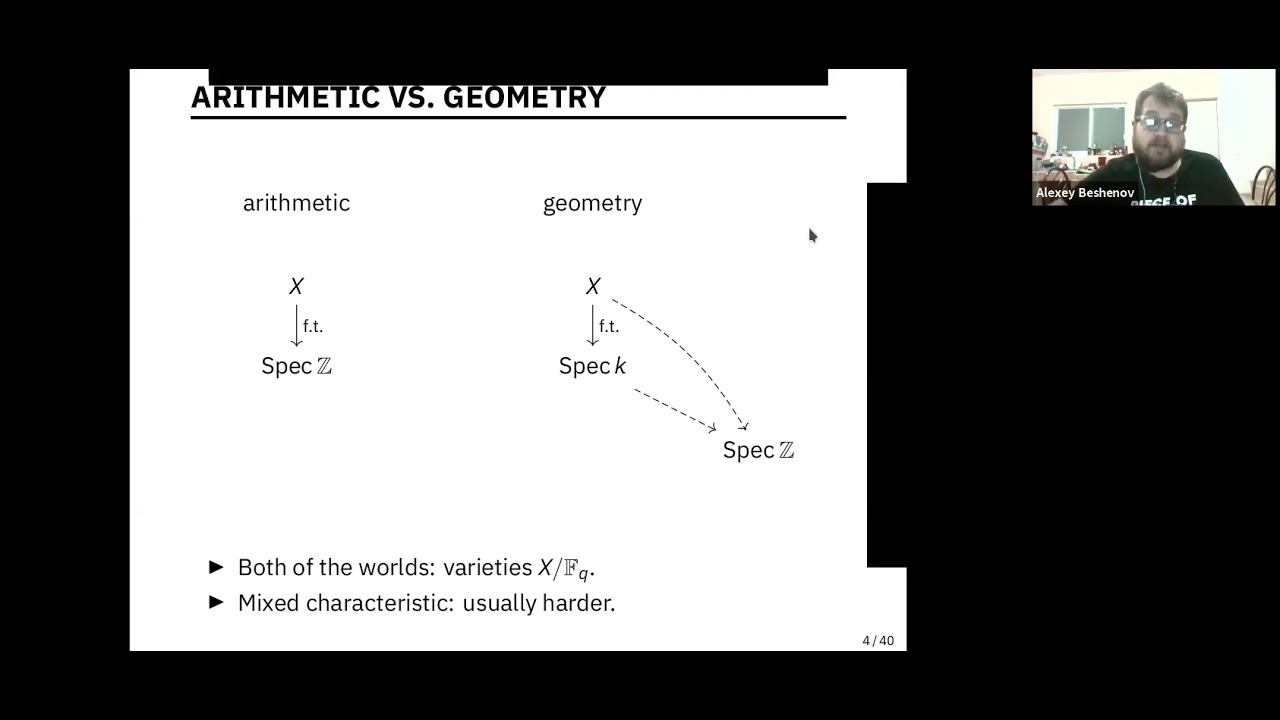
Let X be an arithmetic scheme, by which we will mean that it is separated and of finite type over Spec Z. The corresponding zeta function ζ(X,s) is defined via the usual Euler product over the closed points of X, and for an integer n, the special value ζ*(X,n) is the leading coefficient of the Taylor series of ζ(X,s) around s=n (assuming analytic continuation). Several conjectures, of varying generality, express ζ*(X,n) in terms of arithmetic invariants attached to X (such as algebraic K-theory, motivic cohomology, and regulators).

Lichtenbaum conjectured the existence of Weil-étale cohomology groups H^i_{W,c}(X,Z(n)) that encode the special value of ζ*(X,n). Thanks to the subsequent work of Geisser, Weil-étale cohomology is rather well-understood for varieties over finite fields X/Fq, and the case of mixed characteristic was later considered by Morin and Flach. The construction is based on étale motivic cohomology of X and arithmetic duality.

I will talk about the general context behind Lichtenbaum's Weil-étale program, known results, particular examples, my contributions, and some open problems.