filmov
tv
Ch7Pr26a: Kernel and Nullity of a 4x3 matrix

Показать описание
How to find the kernel and nullity of a 4x3 linear transformation. This is Chapter 7 Problem 26a of the MATH1231/1241 Algebra Notes, presented by Norman Wildberger of UNSW.
Ch7Pr26a: Kernel and Nullity of a 4x3 matrix
Ch7Pr26b: Kernel and Nullity of a 3x4 matrix
Image and Kernel
1.6 Ex2 Finding kernel and range
KERNEL and RANGE of a LINEAR TRANSFORMATION - LINEAR ALGEBRA
Determine the Null Space (Kernel) and Nullity of a Linear Transformation
The rank nullity relation and examples
Given a linear transformation, find the kernel and range
Sec 4.8 Example 1: Kernel, range, and rank-nullity for a linear transformation
The Kernel of a Linear Map
Kernel and Image of a Linear Transformation | Linear Algebra | Griti
Kernel and Image of a Linear Transformation Example 1 | Linear Algebra | Griti
Rank and Nullity of A Example 2
Determine a Basis for the Kernel of a Matrix Transformation (3 by 4)
Linear Algebra - Lecture 32 - Dimension, Rank, and Nullity
kernel and range of a linear transformation and thier dimensions
Example of Kernel and Range of Linear Transformation
Rank Nullity Theorem | Linear Algebra | Griti
RANK and NULLITY of a Matrix | FREE Linear Algebra Course
Sec 4.8 Example 2: Kernel, range, and rank-nullity
Tutorial Q39 -- rank and nullity relations of matrix
Week 9 - Rank-nullity theorem
Rank and nullity of linear map, Rank and Nullity Theorem
Linear Algebra 3.3. Nullity/Kernel of a Matrix A. Finding the basis.
Комментарии
 0:09:58
0:09:58
 0:04:55
0:04:55
 0:05:35
0:05:35
 0:02:45
0:02:45
 0:08:46
0:08:46
 0:57:49
0:57:49
 0:03:16
0:03:16
 0:08:25
0:08:25
 0:18:03
0:18:03
 0:02:47
0:02:47
 0:10:34
0:10:34
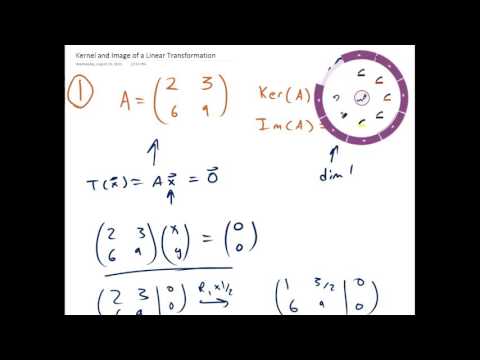 0:06:06
0:06:06
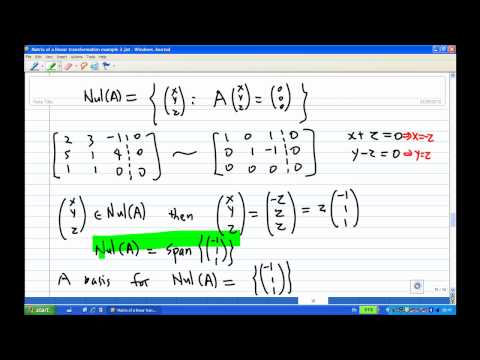 0:03:30
0:03:30
 0:04:16
0:04:16
 0:10:38
0:10:38
 0:00:08
0:00:08
 0:06:31
0:06:31
 0:06:46
0:06:46
 0:10:46
0:10:46
 0:17:32
0:17:32
 0:10:29
0:10:29
 0:01:57
0:01:57
 0:07:42
0:07:42
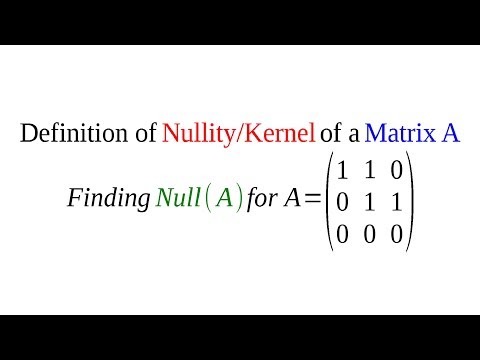 0:09:29
0:09:29