❖ Mixing Problems and Separable Differential Equations ❖

In this video, we explore mixing problems and how to solve them using first-order separable differential equations. Mixing problems are common in chemistry and other real-world scenarios where substances are added to and removed from a tank or container at certain rates.

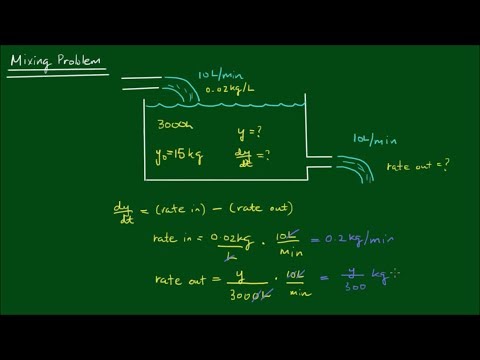
We'll walk through a problem where a salt solution is added to a tank, thoroughly mixed, and drains out at the same rate. Using the concept of rate in minus rate out, we'll set up and solve a differential equation to determine how much salt is in the tank after 't' minutes. Along the way, we’ll cover:

Setting up the differential equation.
Solving for the amount of substance in the tank as a function of time.
Applying initial conditions to find particular solutions.
Interpreting real-world results from the mathematical model.
By the end of this video, you'll understand how to approach and solve mixing problems using differential equations, a powerful technique for modeling dynamic systems.

What You Will Learn:

How to set up a differential equation for mixing problems.
How to solve first-order separable differential equations.
How to apply initial conditions to find particular solutions.
Real-world applications of mixing problems in mathematics.
If you find this video helpful, please consider liking, sharing, and subscribing! Also, let your friends, teachers, or parents know about this channel if you think they would benefit from these lessons.

Support me on Patreon if you'd like to see more content:

Hashtags
#MixingProblems #DifferentialEquations #FirstOrderEquations #MathApplications #SeparableEquations #RateInRateOut #MathHelp #PatrickJMT

[Jyuni]

You are the main reason why i passed calculus 1, 2 and 3. Whenever i have a classmate that is struggling, i'd always tell them to watch your videos. Thank you so much, patrick!

[Macoranino]

10 years down the line and, your material is still relevant !

Great work, Patrick.

[rustyshivers]

I love that the main ad you have is "That Tutor Guy". I haven't even followed the link! For YEARS I have had great success with your videos. Nothing against the other guy. No One on the internet explains with the same clarity and consistency as you.
Thank you!

[neda]

May I say that you are AMAZING, omg finally I found someone that talks in my language. Professors usually skip a lot of steps assuming we know them!!! but your videos makes a lot of sense, that a lot, SUBSCRIBED 

[DangerDonkey]

I've always wanted to know why calculus textbooks always had this big hard-on for big tanks of brine. My textbook literally has a problem exactly like this... with brine. Anyways, you quite literally are the best math teacher on YouTube in my opinion. You're the reason I get by in engineering. If I could, I would donate. Keep being awesome.

[Dan-ykkd]

you and the khan academy guy should team up and be the kings of online education

[mikehenry]

I've been struggling with the modelling of equations exactly like the one you explained in your video for quite a while. All thanks to your amazing teaching ability, I can now do mixing problems flawlessly. Thanks Patrick!

[JoshStoller]

Thank you so much for filling in the gaps left by my Professor.

[jiadongyu]

Nearly 10 years on, this video is still really helpful. Thanks bro!

[MrKittenslayer]

I wish I had known about your tutorials sooner! So much easier to understand than my professor! Thanks a ton!

[williambarnett]

I just came across your channel and this section of differential equations has never been easy for me to understand until I watched this. THANK YOU

[nkad]

Your videos from 2010 are getting me grades in 2018. Can't thank you enough.

[patrickjmt]

@ermitz90 cause i am lazy and it is easier to write A instead of A(t).

it is the same reason people write: y = x - 3 instead of y(x) = x - 3

[blok]

this was extremely helpful! We learned this in Diff. Eq today and i left class thinking it was so tough. You made it so much simpler. I swear im spending the rest of the semester studying your videos! Thanks!!

[RGDynamics]

This made a hell of a lot more sense than my textbook, thanks for the crystal clear explanations.

[juniorjay]

Everybody kick in a few bucks to his Patreon, he deserves it more than most of our math professors!

[nicholasmartin]

Your explanation is very good. I'm only a sophomore in high school, and I understood you pretty well. Great video.

[OnTheBrink]

my professor completely blew through this stuff and my textbook is basically useless. Thanks for explaining it so clearly! you're a lifesaver =)

[williamvasiladiotis]

I know it’s been 8 years but thank you so much for this video! Much clearer than my ODE class

[ryantanner]

this video is awesome still 13 years later thank you!