filmov
tv
A Cuter Way to Undo Square Roots
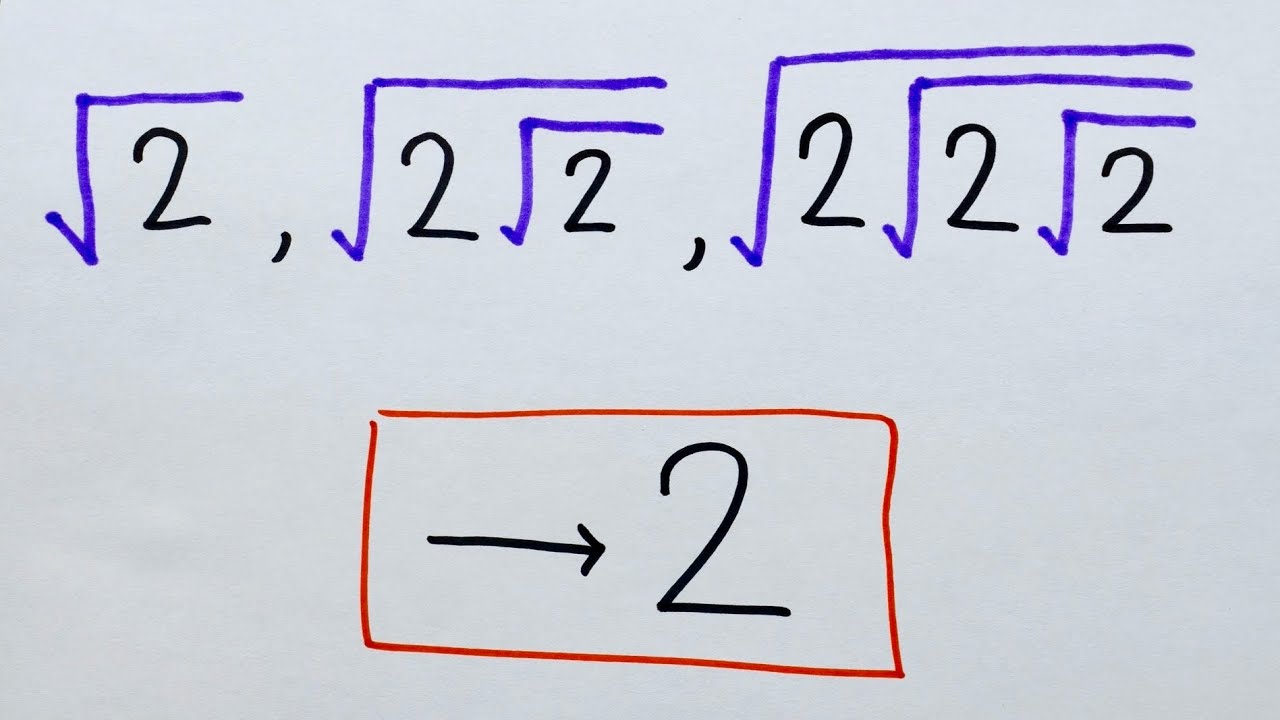
Показать описание
We prove an adorable sequence of nested square roots converges to 2 using the monotone convergence theorem and some basic algebra. We are looking specifically at a recursive sequence and will find proof by induction very useful. #calculus2 #mathematics
Join Wrath of Math to get exclusive videos, lecture notes, and more:
Textbooks I like!
★DONATE★
Outro music by Ben Watts and is available for channel members.
Follow Wrath of Math on...
Join Wrath of Math to get exclusive videos, lecture notes, and more:
Textbooks I like!
★DONATE★
Outro music by Ben Watts and is available for channel members.
Follow Wrath of Math on...
A Cuter Way to Undo Square Roots
A Better way to Undo Mistakes | #UnityTips
I want to remove the battery from this gadget. Chinchilla hamster. Cute pet debut plan. Raising
it's won't remove 😭 | my talking tom #mytalkingtom2 #talkingtom #cute #challenge #funny
A better way to remove Polish 💀
How To Gleek Easily!! 😱😮
Remove the stain | my talking tom #shorts #mytalkingtom2 #funny #cute #challenge
7 TIPS | How to Remove Box Braids without Breakage and Better Length Retention
Remove the first and last letter of your name #youtubeshorts #cute #funny #trend
Cutter undo... 😂🤣💢|fun tube YT| #comedy #shorts
How to remove catarrh from babies nose #catarrh #nosefrida #baby #snotsucker #nasalwash #shortsfeed
Better Than AirTags For Stolen E-Bikes and Vehicles | How to Remove Atuvos Speakers
HOW TO REMOVE OLD VINYL FLOOR 💪💪💸💸FASTER BETTER CHEAPER ,MULTI TOOL 🔥😱😱
Remove the first and last letter of your name...👹#cute #cat #shorts #fyp #trending #trendingshorts...
I haven’t found a better way to easily remove a protruding stripped screw. #shorts
How To Remove The Watermark On Cute Cut Tutorial!
Better way to remove a toilet. #shorts #youtubeshorts #diy
How To Remove Unwanted Grass Using A Sod Cutter ✂️✂️✂️
Rolling cutter process for cutting tires
A Better Way to Remove Background in Photoshop (Detailed Subject) #shorts #shortsvideo #short
Remove the first and last letter of ur name #dogshorts #cute #funny
A quick how to remove nail products #nails #cute #diy #nailvideos #removal #acrylicnails #gelnails
#Insulation #remove to one #Side #Cuter #Viral #Short#feedshorts Tech for YouTube 👀💛💙#Tech #Life #Pk...
A better way to remove anything from boiling oil or a pan #kichentools #machinery #facts #tools
Комментарии
 0:16:50
0:16:50
 0:00:37
0:00:37
 0:00:06
0:00:06
 0:00:08
0:00:08
 0:00:22
0:00:22
 0:00:24
0:00:24
 0:00:07
0:00:07
 0:05:01
0:05:01
 0:00:07
0:00:07
 0:00:14
0:00:14
 0:00:04
0:00:04
 0:10:39
0:10:39
 0:00:16
0:00:16
 0:00:07
0:00:07
 0:00:19
0:00:19
 0:02:02
0:02:02
 0:00:23
0:00:23
 0:00:37
0:00:37
 0:00:05
0:00:05
 0:01:00
0:01:00
 0:00:07
0:00:07
 0:00:45
0:00:45
 0:00:20
0:00:20
 0:00:39
0:00:39