filmov
tv
Vector Spaces 1: What is the dimension of R over Q?

Показать описание
This video asks -- and answers -- the question: What is the dimension of R over Q? We explore what it means that a vector space has a dimension, look at some examples, and state a theorem which will be proved in the next video.
Understanding Vector Spaces
Vectors | Chapter 1, Essence of linear algebra
Vector Spaces | Definition & Examples
VECTOR SPACES - LINEAR ALGEBRA
Abstract vector spaces | Chapter 16, Essence of linear algebra
Linear subspaces | Vectors and spaces | Linear Algebra | Khan Academy
What is a Vector Space? (Abstract Algebra)
4.1 Vector Spaces and Subspaces
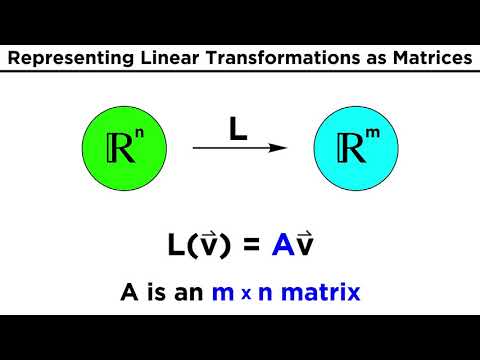
Matrices for General Linear Transformations | Linear Algebra
Oxford Linear Algebra: What is a Vector Space?
Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra
Vector space and subspace-Part one
Vector Space | Definition Of Vector Space | Examples Of Vector Space | Linear Algebra
Vector intro for linear algebra | Vectors and spaces | Linear Algebra | Khan Academy
Vector Spaces: Definitions and Axioms
Vector spaces | Lecture 16 | Matrix Algebra for Engineers
Vector Spaces 1: What is the dimension of R over Q?
Linear Algebra| Introduction of Vector space | Degree Mathematics in telugu
Introduction to Vector Spaces | Detailed Definition with Examples
Advanced Linear Algebra 1: Vector Spaces & Subspaces
Lecture - 1.1 Vector Spaces
Linear Transformations on Vector Spaces
How to Prove a Set is a Subspace of a Vector Space
Linear Algebra 4.1.2 Subspace of a Vector Space
Комментарии
 0:08:41
0:08:41
 0:09:52
0:09:52
 0:08:11
0:08:11
 0:13:03
0:13:03
 0:16:46
0:16:46
 0:23:29
0:23:29
 0:06:58
0:06:58
 1:14:57
1:14:57
 0:18:08
0:18:08
 0:29:53
0:29:53
 0:09:59
0:09:59
 0:12:05
0:12:05
 0:20:52
0:20:52
 0:05:49
0:05:49
 0:10:59
0:10:59
 0:07:23
0:07:23
 0:08:56
0:08:56
 0:29:33
0:29:33
 0:08:18
0:08:18
 0:41:25
0:41:25
 0:33:04
0:33:04
 0:09:11
0:09:11
 0:05:58
0:05:58
 0:17:58
0:17:58