filmov
tv
Solve the equation 2 sin^2 x + 3 sin x + 1 = 0
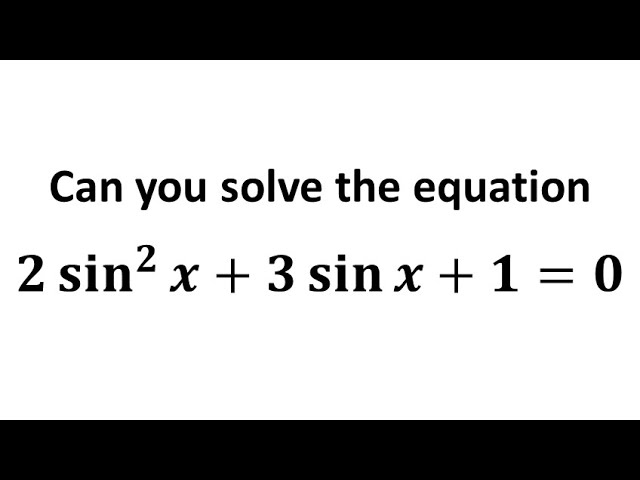
Показать описание
In this video, we will learn to solve the equation “2 sin^2 x + 3 sin x + 1 = 0”. The equation “2 sin^2 x + 3 sin x + 1 = 0” is the twenty sixth question of 2.3 section of the book “Trigonometry by Ron Larson”.
Following link of the video explains the proof of the identity sin(pi + x) = - sin(pi + x)
Following link of the video explains the proof of the identity sin(-x) = - sin x
Other topics of this video:
Solve 2 sin^2 x + 3 sin x + 1 = 0
Find values of x when 2 sin^2 x + 3 sin x + 1 = 0
How to solve 2 sin^2 x + 3 sin x + 1 = 0?
I, Ravi Ranjan Kumar Singh, have produced this video. All credits of this video belong to me.
Following link of the video explains the proof of the identity sin(pi + x) = - sin(pi + x)
Following link of the video explains the proof of the identity sin(-x) = - sin x
Other topics of this video:
Solve 2 sin^2 x + 3 sin x + 1 = 0
Find values of x when 2 sin^2 x + 3 sin x + 1 = 0
How to solve 2 sin^2 x + 3 sin x + 1 = 0?
I, Ravi Ranjan Kumar Singh, have produced this video. All credits of this video belong to me.
0:04:42
Solve Trig Equation by Factoring: 2sin^2(x)-sin(x)-1=0 (Radians)
0:04:04
Solve sin=2/5 Trigonometric equation
0:02:34
Solve the Trig equation 2 sin^2 x -5 sin x + 3 = 0 on the interval [0, 2pi)
0:03:15
Solve 2sin^2(x) + 3sin(x) + 1 = 0
0:12:56
Trigonometric Equation sin 2x = half in 0 to 2 pi domain MHF4U
0:03:43
PreCalculus - Trigonometry: Trig Identities (48 of 57) Solve 2sin^2(theta)-sin(theta)-1=0, theta=?
0:03:23
Solve 2sin^2x+cosx-1=0 (fast in 3.2 minutes, no details skipped)
0:05:05
Find All Solutions to the Equation 2sin(x) - 1 = 0
1:29:10
Trigonometry Question 5 - 7 Mathematics Paper 2 Grade 12 Pretrial 2023 Gauteng
0:02:30
Solve equation 2 cos x + sin 2x =0 over [0, 2pi). Double Angle Formula
0:01:22
2sin^2(x) - 1 = 0 Solve for interval 0 less theta less 2pi
0:08:59
Solve the equation 2sin 3x - sin x = 0
0:05:15
Trigonometric Equation Sin(2x+π/3)=½
0:01:34
How to prove that sin^2x - cos^2x = 1 - 2sin^2x
0:02:58
Solving trigonometric equations with multiple angles
0:02:58
Solve the Trig equation tan x = 2 sin x on the interval [0, 2pi)
0:01:52
Solve for all values of x when 2 sin x +1 =0 over [0, 360]
0:00:55
Trigonometric Equation: Solve sin (2x) = cos (x)
0:01:26
How to Find Sin 2 Theta : Grade School Math Questions
0:07:29
solving a trigonometric equation sin(2x)=cos(x) with the double angle identity, precalculus tutorial
0:01:27
If `2sin^2theta-cos^2theta=2,` find the value of `theta.`
0:03:59
Derivative of sin(x^2), sin^2(x), and sin(2x) with Chain Rule | Calculus 1 Exercises
0:07:45
2 Sin^2 x + √3 cos x +1=0 find minimum value of x
0:01:40
Find the general solutions of the following equation: `2\ sin\ theta+1=0`
Комментарии