solving a trigonometric equation sin(2x)=cos(x) with the double angle identity, precalculus tutorial

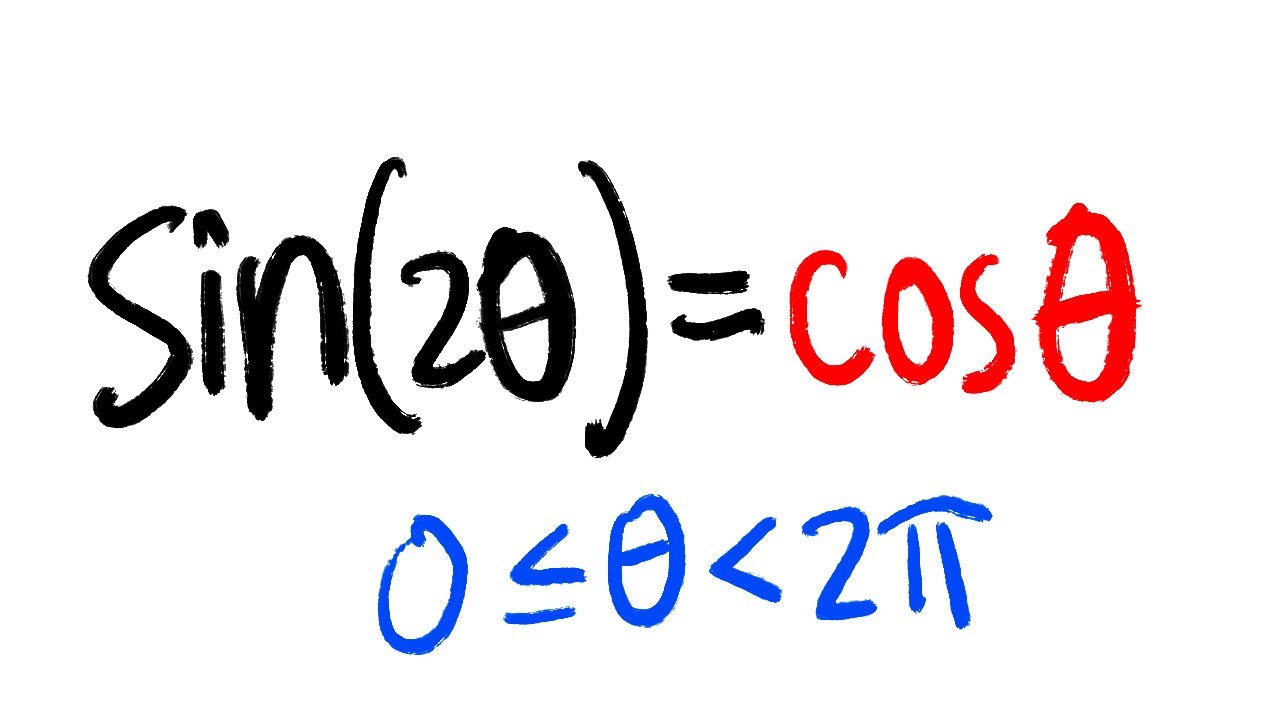
Показать описание

Learn how to solve this trigonometric equation sin(2theta)=cos(theta) for your trig or precalculus class. We will use the double angle formula for sine and turn this into a quadratic equation.
----------------------------------------
#blackpenredpen #math #trigonometry #apcalculus

blackpenredpen

Рекомендации по теме

Комментарии

It shall be (2n+1)pi/6 where n=0 to 5, so we have 6 solutions within the range.
Sin(2t)=cos(t)=sin(pi/2-t)
=> 2t=n*pi+pi/2-t
=>t=(2n+1)pi/6
Since 0<=t<=2pi, 0<=n<=5

jigshere

if we divide cos(o) on both sides
2sin(o) = 1
sin(o) = 1/2
o = pi/3 + 2pik or 2pi/3 + 2pik

nemanjalazarevic

Thanks for the explanation. It helped a lot.

harhar

At 0:54 you could also just divide by cos(theta) on both sides to get 2sin(theta)=1, because cos(theta) can't be 0 because theta must be less than 2(pi), as you said it was in the thumbnail.

frede

How do you know to avoid dividing both sides by cos(theta) since it will hide two possible solutions?

JefiKnight

or you could have used the firmulas...
cosx = 0
cosx = cos(π/2)
so x = 2κπ + π/2
οr x = 2κπ - π/2, κ is integer
and you want
0 <= x <= 2π
so u get
π/2, 3π/2

sinx = 0.5
sinx = sin(π/6)
x = 2κπ + π/6
or x = 2κπ + π/6
// π - π/6 = 5π/6

and u get π/6
and 5π/6

theopapa

Hey, what's with the blue pen! Just joking, love your videos.

vvop

Thank you so much I am slowly getting the hang of it have a test on it tomorrow

beast_gago

in degrees :
sin(2x) = cos(x)
sin(2x) = sin(90 - x)
2x = 90 - x
3x = 90
x = 30

answer tau/12

AlgyCuber

Why you wrote under root 3 and under root -3? In base

tayyabasadiq

Nice video bro!
Can you explain me why is pi/6?

imanolmanzanares

[ 2sinQcosQ=cosQ => 2sinQ= 1 => sinQ=1/2 ] ?

virunkhoem

Solving simple question in a complex way.
See my solution:
sin(2©)=cos©
sin(2©)=sin(90°-©)
2©=90°-©
3©=90°
©=30°
Other values of© are(180°-30°)=150°
(360°-30°)=330°
(360°-150°)=210°

Shreyas_Jaiswal

solving a trigonometric equation sin(2x)=cos(x) with the double angle identity, precalculus tutorial

Trigonometric Equation: Solve sin (2x) = cos (x)

solving a trigonometric equation sin(2x)=cos(x) with the double angle identity, precalculus tutorial

Solving Trig Equations sin(2x)=cos x

Solve the Trig equation sin(2x) = cos x on the interval [0, 2pi)

Solve the Trigonometric Equation sin(2x) = cos(x) After Applying a Trig Identity

sin2x=cosx, Solve a Nice Math Olympiad Trigonometry Equation

Find all values of x in the interval [0, 2π] that satisfy the equation: sin 2x=cosx | Plainmath

Solve: sin 2x - cos x = 0

Exact Solution of Trigonometric Equation Exam Problem

Trigonometric Equation Sin 2x Q7

How to Solve cos(x) + sin(2x) = 0 (Trigonometric Equations)

Solve Sin(2x) + Cos(x) = 0 in [0,2pi)

Solve 2sin^2x+cosx-1=0 (fast in 3.2 minutes, no details skipped)

Solve the Equation sin (2x) = cos(x) for Solutions from 0 to 2pi

Solve sin=2/5 Trigonometric equation

Solve Trigonometric Equation 3cosx sin^2 - cosx = 0

Learn to solve your trigonometric equation with sine squared

Grade 11 Maths Trig Equation - sin(2x)=cos(x+30) for xϵ[0,360]

Trigonometric Equation Sin(2x+π/3)=½

Trigonometric Functions || Solve the following trigonometric equation :Sin 2x + cos x= 0

General Solutions of Trigonometric Equation sin(x)-3sin(2x)+sin(3x) = cos(x)-3cos(2x)+cos(3x)

A-Level Maths: E7-27 [Trig Equations: Solve sin(2x) = 0.8 between 0 and 360 degrees]

Solve the Trig Equation sin(x/2) = cos(x/2) for the values of x

Solve Trig Equation Cos2x Equals Sinx Q4