Linear Correlation 21 Correlation between Age and Literacy

Показать описание

Statstics
MBA, MCA, CA, CPT, CS, CWA, CMA, FOUNDATION, CPA, CF, BBA, BCOM, MCOM, Grade-11, Grade-12, Class-11, Class-12, CAIIB, FIIIIAS, UPSC, RRB, Competitive Exams, Entrance Exams

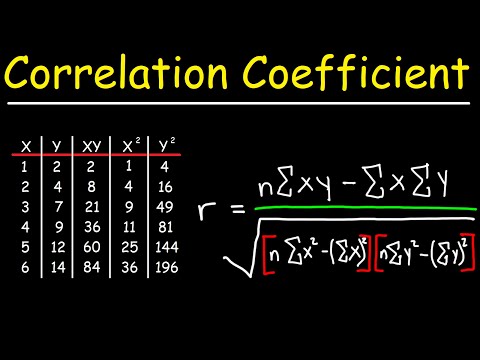
Linear Correlation - 21

Correlation between Age and Literacy:
In this case we requireto study the correlation between Age and Literacy. While age is naturally a quantitative measure, literacy is a qualitative measure. So, first of all we have to find some statistical/numerical value of literacy to make it a quantitative measure. Here, we have taken the percentages of the literate people to the total population as numerical value of literacy.

So, Literacy = (No. of literate people / Total Population) * 100

In case of age, we have taken the mid-value.

In this way, now we have two variables Age (say, x) and Literacy (say, y)

In this case also we have the benefit of change of origin and scale in case of age and of only change of origin in case of literacy.

A very important characteristic of the coefficient of correlation is "it is independent of change of origin and scale".

That means even if we change origin and/or scale of the original Variable(s) and create a new/revised variable, to ease the computation, there will be no change in the value of the coefficient of correlation.

Change of Origin:
If any constant value is added to or subtracted from the original values of any variable, to make the values reasonably large or small, then it is called 'Change of Origin'

e.g. u = (x - A) or u = (x + A), v = (y - B) or v = (y + B)

Change of Scale:
If we multiply or divide the original values of a variable by a constant value, to make the values reasonably large or small, it is called 'Change of Scale'.

e.g. u = (x * C) or u = (x/C), v = (y * B) or v = (y/B)

When we change origin and scale of a variable simultaneously, the resulting new/revised variable can be -

u = [(x - A)/C] or u = [(x + A)/C] or u = [(x - A) * C] or u = [(x + A) * C]

V= [(Y - B)/C] or v = [y + B)/C] or v = [(y - B) * C] or v = [(v + B) * C]

The problem discussed has variable 'price' in the form of class intervals. So, we have to take the mid-values of the class intervals as the various values of the variable 'price'. While in case of the values of another variable 'demand' no such change is needed.

Important suggestions:
When we go for change of origin by subtracting a constant value (which is known as assumed mean):

1) It is advisable to take the arithmetic mean of the variable itself as its assumed mean that will give the summation of the revised variable, after change of origin, exactly zero to substitute in the formula and thus will make the calculation very easy.

2) If the arithmetic mean of a variable is a fractional value, then it is advisable to take the integer value immediately preceding the arithmetic mean as the assumed mean. It will give a smallest possible positive total of the new variable after changing the origin to substitute in the formula. As a result (a) the calculations would become utmost easy and there will be no or possibly minimum number of negative values substituted in the formula which will save you from making the possible mistakes.

If we take a value greater than arithmetic as the assumed mean to subtract for changing the origin, it always gives a negative total of the new variable after changing origin.

Always remember:

(1) The summation/total of the deviations taken from the arithmetic mean is always zero.

(2) The summation/total of the deviations taken from the assumed mean less than the arithmetic mean is always positive.

(3) The summation/total of the deviations taken from the assumed mean greater than arithmetic mean is always negative.

So, it is advisable to take the assumed mean either equal to the arithmetic mean of the variable or less than the arithmetic mean.

In case of change of scale -
(1) If the original values of the variable are large enough in tens or hundreds or thousands, divide them by 10 or 100 or 1000 accordingly. That will reduce them considerably/reasonably without affecting the value of the coefficient of correlation.

(2) If the original values of the variable are small enough as fractional values with one decimal value or two decimal value or three decimal value, then multiply them by 10 or 100 or 1000 accordingly. That will increase them considerably/reasonably without affecting the value of the coefficient of correlation.