filmov
tv
believe in the math, not wolframalpha
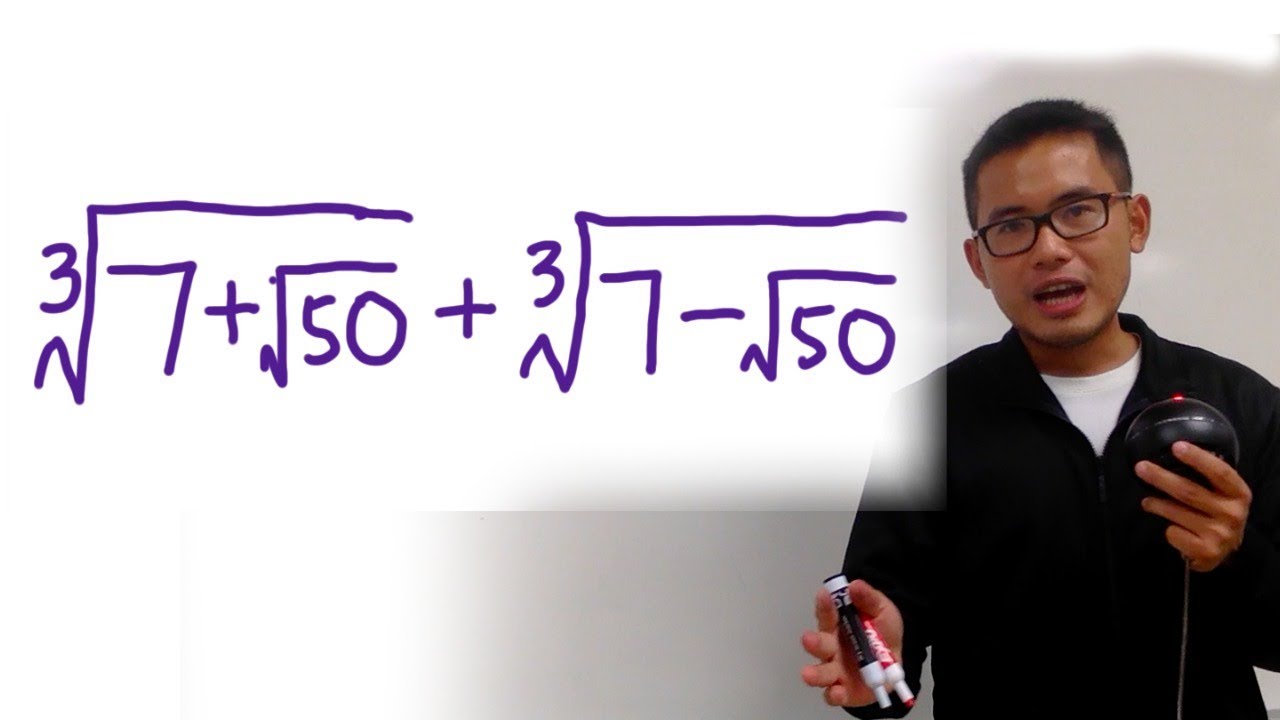
Показать описание
Believe in the math, not WolframAlpha. We will simplify this nested radical expression. This expression is usually from the cubic formula. Surprisingly, this expression gives us a whole number! Enjoy!
For more calculus tutorials, check out my new channel @just calculus
For more calculus tutorials, check out my new channel @just calculus
believe in the math, not wolframalpha
Believe In Reason, Not Math
Proving God exists using Math
Stop Trying to Understand Math, Do THIS Instead
The Simplest Math Problem No One Can Solve - Collatz Conjecture
Insane Math Facts That You Won’t Believe are True
Anyone Can Be a Math Person Once They Know the Best Learning Techniques | Po-Shen Loh | Big Think
Stand and Deliver (1988) - Tough Guys Don't Do Math Scene (2/9) | Movieclips
I Just Can't Believe what the Math is Telling Me
Can Math Prove God's Existence?
Why Do Some People Learn Math So Fast
Math isn't hard, it's a language | Randy Palisoc | TEDxManhattanBeach
Human Calculator Solves World’s Longest Math Problem #shorts
Is math discovered or invented? - Jeff Dekofsky
The 7 Levels of Math
Mathematician Jordan Ellenberg breaks down Math films & TV shows
When Asians Are Bad At Math
Why Math is Important
The math study tip they are NOT telling you - Ivy League math major
A Genius Mathematician Suggested This Simple Idea — And It Could Transform The Way You Do Math
A slacker was 20 minutes late and received two math problems… His solutions shocked his professor.
Why Everyone is a Math Person — Including You | Connie Vaughn | TEDxDayton
Cool Math Trick With Rectangle And Triangle #Shorts
Are you just bad at math? #shorts
Комментарии
 0:14:50
0:14:50
 0:03:13
0:03:13
 0:05:23
0:05:23
 0:05:21
0:05:21
 0:22:09
0:22:09
 0:17:19
0:17:19
 0:03:53
0:03:53
 0:02:19
0:02:19
 0:06:25
0:06:25
 0:04:33
0:04:33
 0:04:14
0:04:14
 0:08:55
0:08:55
 0:00:34
0:00:34
 0:05:11
0:05:11
 0:08:44
0:08:44
 0:15:13
0:15:13
 0:01:00
0:01:00
 0:06:02
0:06:02
 0:08:15
0:08:15
 0:08:49
0:08:49
 0:07:13
0:07:13
 0:10:22
0:10:22
 0:00:30
0:00:30
 0:00:34
0:00:34