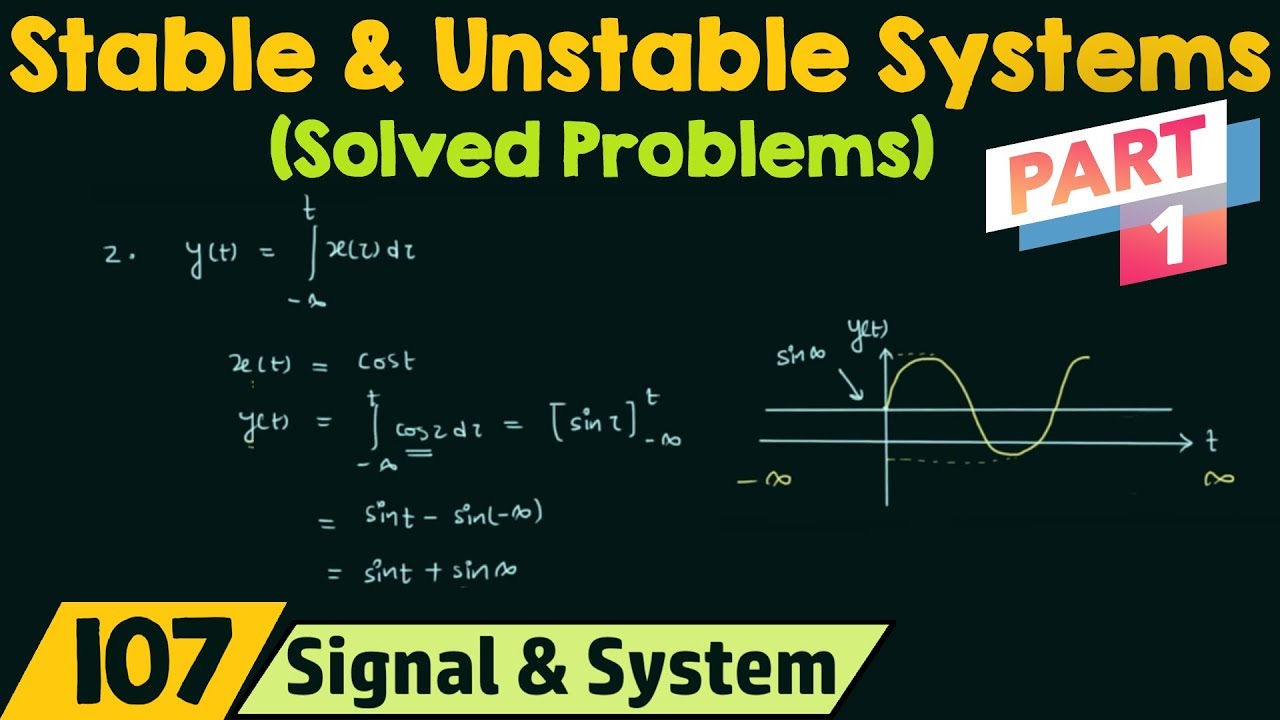
Stable and Unstable Systems (Solved Problems) | Part 1

Signal and System: Solved Questions on Stable and Unstable Systems
Topics Discussed:
1. Examples of stable and unstable systems.
2. Homework problem on stable and unstable systems.

Music:
Axol x Alex Skrindo - You [NCS Release]

#SignalAndSystemByNeso #Signal&System #StableSystem #UnstableSystem #BIBO

[RickJankowski]

Posted by Rajeshwara Raju

unstable
if u take x(t) as u(t) in this case it is stable only but it has to satisfy for all the bounded inputs
even if atleast for one signal it is unbounded means it is unstable system
let us take x(t) as cost
then it will become cos square of t

INTEGRATION { cos square of t }= (1+cos2t)/2

here we get integration of 1/2 which is equal to { 1/2 * t } i.e., ramp with slope 0.5
as we alredy know ramp is not a stable signal it is unbounded

so the system is unstable

[jigneshvala]

System is always stable for finite input, But if we take input is cos(t) we get integration 1/2*(t) + something, & 1/2*(t) is a ramp signal, & ramp signal is a unbounded signal, so for a any one bounded input the output is reaches to infinite of amplitude so the system considering as a unstable system.

[halitcukur]

Sir, you have great capability of teaching. Thank you sir.

[thotavenkateswararao]

please reveal the correct answer for HW problems

[altuber_athlete]

These examples only show if the system is stable *for a particular input.* But the definition of a BIBO-stable system requires that the system be stable for *any* input.

[saikiransiripuram]

Isnt there a common procedure for this kind of questions?do we need to check for that many number of inputs??

[ontimegrad]

Thank you so much for the clear explanation!! But still wondering whether there are some general methods instead of trying functions according to the experience and intuition.

[codeminatiinterviewcode]

as we know that cost is finite(bounded) signal -1<=cost<=1 so, the either stable or unstable this will depend on x(t). so if x(t)= u(t). then ramp signal will be its integral output . which is not bounded and IT IS a UNSTABLE SYSTEM for me.

[senmonkashonen]

For the home work, i used integration by part. at the end i had:
Y(t)=sin(minus inifinty)*x(minus infinite)
So if we assume that x(t) is a bounded input, the output automatically is gonna be bounded. (Because the sin(minus infinity) becomes an amplitude scaling)

[bharatbardiya]

UNSTABLE
if x(t) = cost
y(t) = [1/2t +sin2t/4] with limits
and after limits y(t) = infinite

[maheryagub]

Integral of Cos[Tau] does not converge on {-Infinity, t}. Hence unstable

[premkumarbalu]

If input is u(t) then intergal of u(t) is ramp signal which is unbounded.. Hence it is unstable..

[kuldipgohil]

All the concepts are very excellent and straight forward.
Very easy to learn here.🎶👌🎇

[prachis]

I have a doubt
In complex analysis, we studied that sine function is an unbounded function when we use sin(z).
So in the first example, should we consider the function to be stable or unstable ? And if stable how should we know where to consider x(t) as real function and where as complex function.

[ahmedmohamed-tcou]

In the integration example why you didn't put x(t) = u(t) and by the way it will show an unbounded output

[KRKUMARRANJAN]

Put x(t) = Cos t and Solve With Integration Range.
Ans Will be Infinity ♾️. Hence Unstable

[bhashkarkiaawaz]

Sir how to check linearity in split function ??

[Db-leko]

HW ANSWER
STABLE if you do integration by parts.
You should get u(t).sint + cost as a result which is bounded

[saadjamil]

Stable system: when you put u(t) then from -infinity to 0 value will zero and from 0 to t will be some finite value

[glitchboi]

in sins case limit is -1 to 1 meanwhile cos has no limit so technically unstable since unbounded right?