filmov
tv
Memory Lane: Vertical and Horizontal, Parallel and Perpendicular

Показать описание
In this video, we look at the equations of vertical and horizontal lines, as well as how to identify when two lines are parallel or perpendicular.
Memory Lane: Vertical and Horizontal, Parallel and Perpendicular
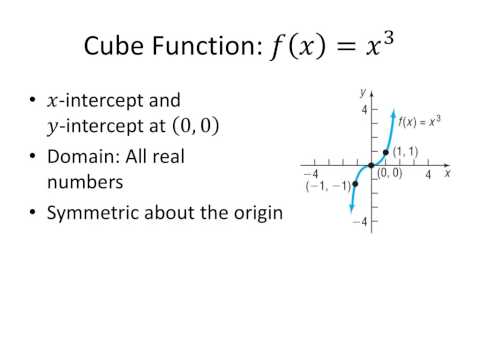
Memory Lane: Library of Functions
Vertical and Horizontal Lines - Visualizing Algebra
Memory Lane: The Idea of Slope
3.4 Parallel and Perpendicular for Horizontal and Vertical Lines
Vertical and Horizontal Lines
Vertical Memory Fast Lane Slideshow #Shorts
100 Photo - Logo Reveal After Effects Templates
Photo Slideshow – Free Download After Effects Template
Writing Equations of Parallel Lines Horizontal and Vertical
Graphing Vertical and Horizontal Lines
Graphing Vertical and Horizontal Lines
Write equation of perpendicular line through point (horizontal and vertical lines)
Parallel, Perpendicular, Horizontal, Vertical Lines
Vertical and horizonal lines parallel or perpendicular (7 of 7)
Elite Lifter Pranks Commercial Gym 👹
Horizontal and Vertical Parallel and Perpendicular Lines
Senior QUIZ: Remember these vintage objects? PART 3 🧠 Test your memory! 👑
Horizontal Vertical Parallel and Perpendicular Lines
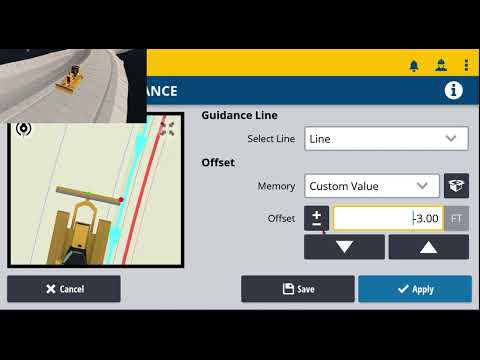
Trimble Earthworks Horizontal Guidance
Title Green Screen Top free History Brush Transition templates Green Effects in 4K Resolution.
How to Draw Straight Lines Vertical & Horizontal in MS Word (2003-2016)
His reaction when he sees her FEET for the first time…😳 #Shorts
Build a Slicer Panel in Power BI (2022/2023)
Комментарии
 0:06:15
0:06:15
 0:04:28
0:04:28
 0:00:43
0:00:43
 0:04:11
0:04:11
 0:01:49
0:01:49
 0:05:14
0:05:14
 0:00:24
0:00:24
 0:00:31
0:00:31
 0:01:21
0:01:21
 0:04:20
0:04:20
 0:06:11
0:06:11
 0:03:37
0:03:37
 0:01:40
0:01:40
 0:01:43
0:01:43
 0:11:45
0:11:45
 0:00:35
0:00:35
 0:06:06
0:06:06
 0:10:02
0:10:02
 0:13:51
0:13:51
 0:07:59
0:07:59
 0:01:31
0:01:31
 0:01:56
0:01:56
 0:01:00
0:01:00
 0:10:32
0:10:32