filmov
tv
Algebra 1 Practice - Write an Equation in Slope-Intercept Form (Example 2)
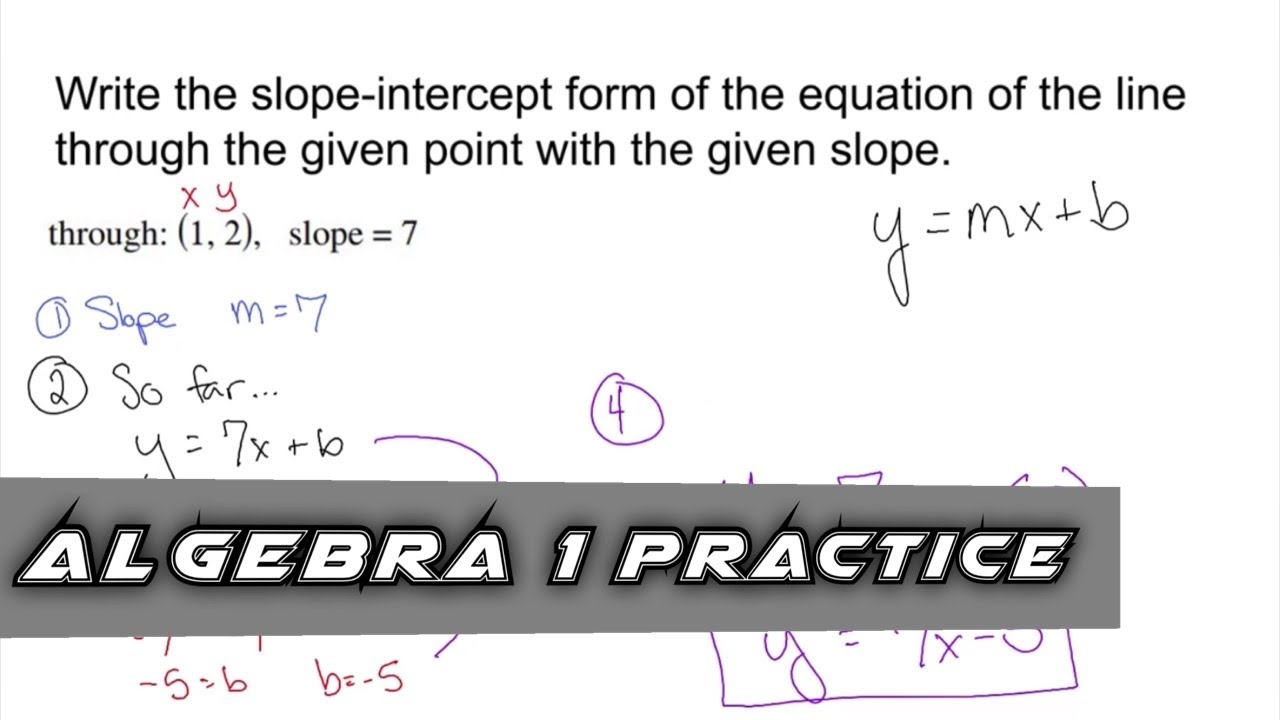
Показать описание
Sure, here's how to write an equation in slope-intercept form directly, given information about the slope, points, or y-intercepts:
### 1. **Using Slope and Y-Intercept**
If you have the slope \( m \) and the y-intercept \( b \), you can directly write the equation in slope-intercept form:
\[ y = mx + b \]
**Example:**
- Slope (\( m \)) = 3
- Y-intercept (\( b \)) = -2
The equation is:
\[ y = 3x - 2 \]
### 2. **Using a Point and the Slope**
If you have the slope \( m \) and a point \((x_1, y_1)\), you can find the y-intercept \( b \) and then write the equation in slope-intercept form.
1. **Calculate the y-intercept \( b \):**
Rearrange the slope-intercept form \( y = mx + b \) to solve for \( b \):
\[ b = y - mx \]
Substitute the coordinates of the point \((x_1, y_1)\) and the given slope \( m \):
\[ b = y_1 - m x_1 \]
2. **Write the equation:**
Substitute the slope \( m \) and y-intercept \( b \) into the slope-intercept form:
\[ y = mx + b \]
**Example:**
- Slope (\( m \)) = 4
- Point = (2, 5)
Calculate \( b \):
\[ b = 5 - 4 \cdot 2 \]
\[ b = 5 - 8 \]
\[ b = -3 \]
The equation is:
\[ y = 4x - 3 \]
### 3. **Using Two Points**
If you have two points \((x_1, y_1)\) and \((x_2, y_2)\), follow these steps:
1. **Calculate the slope \( m \):**
\[ m = \frac{y_2 - y_1}{x_2 - x_1} \]
2. **Find the y-intercept \( b \):**
Substitute one of the points and the slope into the equation \( y = mx + b \) to solve for \( b \):
\[ b = y - mx \]
Use one of the given points \((x_1, y_1)\):
\[ b = y_1 - m x_1 \]
3. **Write the equation:**
Substitute the slope \( m \) and y-intercept \( b \) into the slope-intercept form:
\[ y = mx + b \]
**Example:**
- Points = (1, 3) and (4, 11)
Calculate the slope \( m \):
\[ m = \frac{11 - 3}{4 - 1} = \frac{8}{3} \]
Find the y-intercept \( b \):
\[ b = 3 - \frac{8}{3} \cdot 1 \]
\[ b = 3 - \frac{8}{3} \]
\[ b = \frac{9}{3} - \frac{8}{3} \]
\[ b = \frac{1}{3} \]
The equation is:
\[ y = \frac{8}{3}x + \frac{1}{3} \]
Using these methods, you can determine the slope-intercept form of a linear equation given the necessary information.
I have many informative videos for Pre-Algebra, Algebra 1, Algebra 2, Geometry, Pre-Calculus, and Calculus. Please check it out:
/ nickperich
Nick Perich
Norristown Area High School
Norristown Area School District
Norristown, Pa
#math #algebra #algebra2 #maths
 0:23:05
0:23:05
 0:00:41
0:00:41
 0:05:00
0:05:00
 0:12:07
0:12:07
 0:37:09
0:37:09
 0:00:48
0:00:48
 0:00:18
0:00:18
 0:10:43
0:10:43
 0:46:21
0:46:21
 0:12:52
0:12:52
 0:25:05
0:25:05
 0:32:05
0:32:05
 0:00:33
0:00:33
 0:00:51
0:00:51
 0:00:21
0:00:21
 0:06:54
0:06:54
 0:00:34
0:00:34
 0:00:57
0:00:57
 0:08:39
0:08:39
 0:15:55
0:15:55
 0:55:18
0:55:18
 0:10:29
0:10:29
 0:12:09
0:12:09
 0:09:13
0:09:13