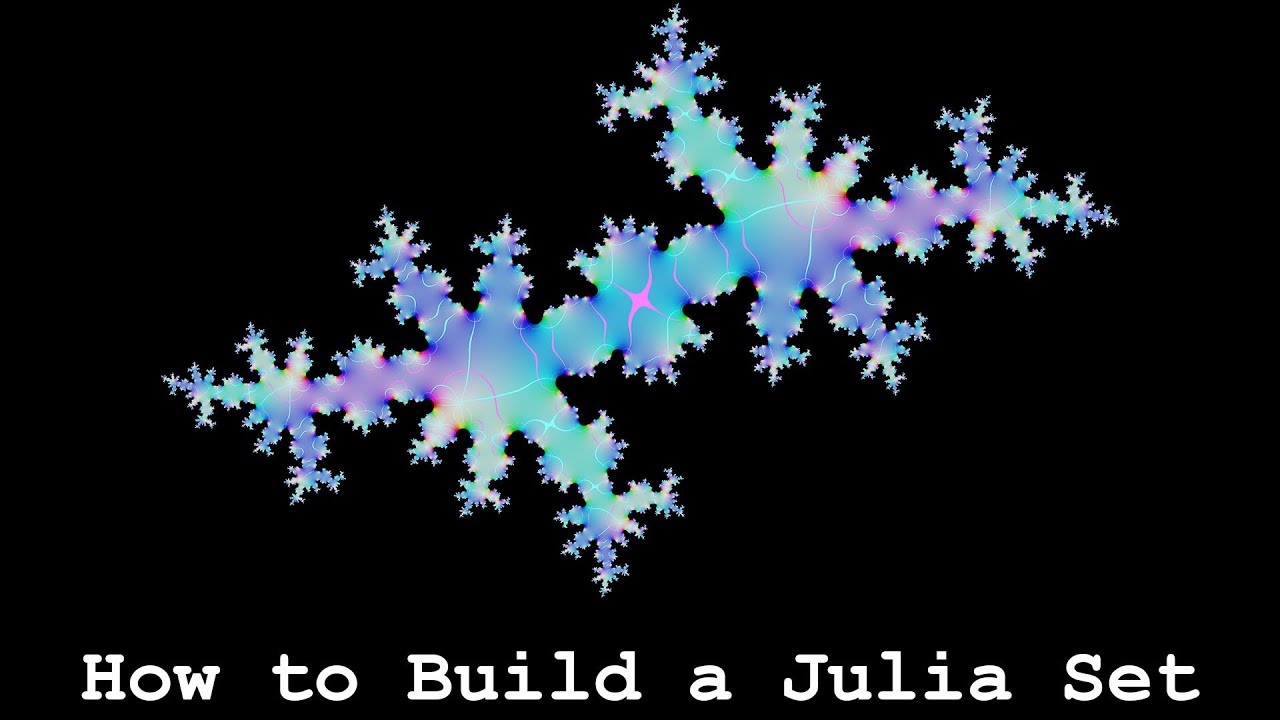
How to Build a Julia Set

In this video we examine how to build a Julia Set by making repeated remapping of a circle on the complex plane. The technique is quite interesting because it allow us to visually understand why Julia Sets have some of their properties, such as rotational symmetry. This video only glosses over the mathematics, and don't worry if you don't exactly understand what is going on. The important part is to look at Julia Set being formed visually. I'm certainly not the first to use this technique, but there isn't much YouTube content, so I've included quite a few visuals, and everything is rendered at a super high 8k resolution. Consider playing the final visuals at slower speeds if you wish to study them. Please subscribe!

In this series:

Extra Visuals (No commentary):

[DrakenFire]

Fascinating. After seeing your videos i understand why mathematicians often say that math is art.

[lagduck]

Sir this is pure gold, I feel lucky to be among 5325 lucky viewers of your art

[lengeschder]

I appreciate the effort you've put into all of these videos. I've always wanted to learn more about fractals, and these presentations do an incredible job of being both informative and just plain fun to watch. Thank you for sharing!

[livemorrow]

An interesting point not stated in the video: For disconnected Julia sets, the point of iteration when building it when the set "breaks up" is the same iteration where the corresponding point (not) in the Mandelbrot set goes out of bounds. For the set at the end, it will take another 50 or so iterations for the set to become disconnected, though it would be hard to tell without zooming in.

[beanos]

best video of julia set explanation on youtube! thanks man, you reallu made my life easier. instant like

[flixgribv]

Thank you for this video, it made it kinda easier to understand how does this actually work!

[ilikepizza]

I was so surprised you only have 900 subscribers, this video is awesome keep it up!

[TheGandorX]

This is very insightful: Mandelbrot is a mere study of the 0+0.i point of julia set "c". And the inverse julia set construction showing how the fractal is formed.
Excellent.

[denelson]

You should have also done a Julia set for a Misiurewicz point, such as c = I.

[Intrebute]

Does the example that ends at 14:16 ever break apart? It feels like it's always closer and closer to exploding into dust

[MaxKili]

Why is the equation z=z^2+c so unique and not z=z^3+c or z^4 etc. and what happens when z is raised to a different power? Does it make a shape and are they usable? Too much chaos?

Is there a correlation between how everything past 2 or -2 goes off towards infinity because z is being squared vs if it was being raised to say the 3rd power would the points start to go toward infinity if they were outside 3 or -3?

Thanks for all the educational and visually impressive videos!

[elizabethojinal]

My favorite Julia set is the last part. And the disconectted Julia is called the Fauto Dust

[cacamikaz]

I find it easier to understand the process when you make the "negative root" part of the image appear stepwise

[ishu]

11:50
Therapist: Snake JUlia doesnt exist, it cant hurt you.
*Snake Julia:*

[THE_ONLY_GOD]

Can confirm if this understanding of difference between Mandelbrot and Julia shader calculations is correct?:
Main difference is seemingly that a Mandelbrot set has a C val that changes every pixel as it basically seems to do a “for loop” style scan across each row of texture coordinates row by row in the entire frame.
So at each point it is calculating the pixel color for, it inputs that texture coordinate under that pixel as C.
In a julia set Z is initially set to the texture coordinate it’s rendering the pixel color for, but C is a constant coordinate val that is shared by every pixel (texture coordinate under the pixel) calculation and that val is from a specified n+i plane coordinate selected. (so in an interactive shader, the coordinate under the touch is C and then Z is every pixel coordinate in a similar “for loop” style row by row scan as the Mandelbrot).
That is seemingly how that functions.

[cacamikaz]

Because you started the reverse iteration from a complete circle of radius 2, and you arrived in a filled julia set,
does that mean that the orbits of the filled julia set will necessary go through any part of the circle ?

[cacamikaz]

Is the result the same if you change the initial shape ?

[Idkgivemeone]

You don’t need 4d things you can use 3d and the third dimension is iterations

[ryanmcginley]

Please let us now know what iterations switched over Julia’s? Let’s see:
10:21
8:09
8:11
8:13
More than a dozen iterations have Julia’s? Let’s see:
10:21
9:17
9:19
9:26
9:28
9:31
9:34
Maybe iterations go, this one will go away (always 20 months)

[ishu]

11:50
Therapist: Snake JUlia doesnt exist, it cant hurt you.
*Snake Julia:*