filmov
tv
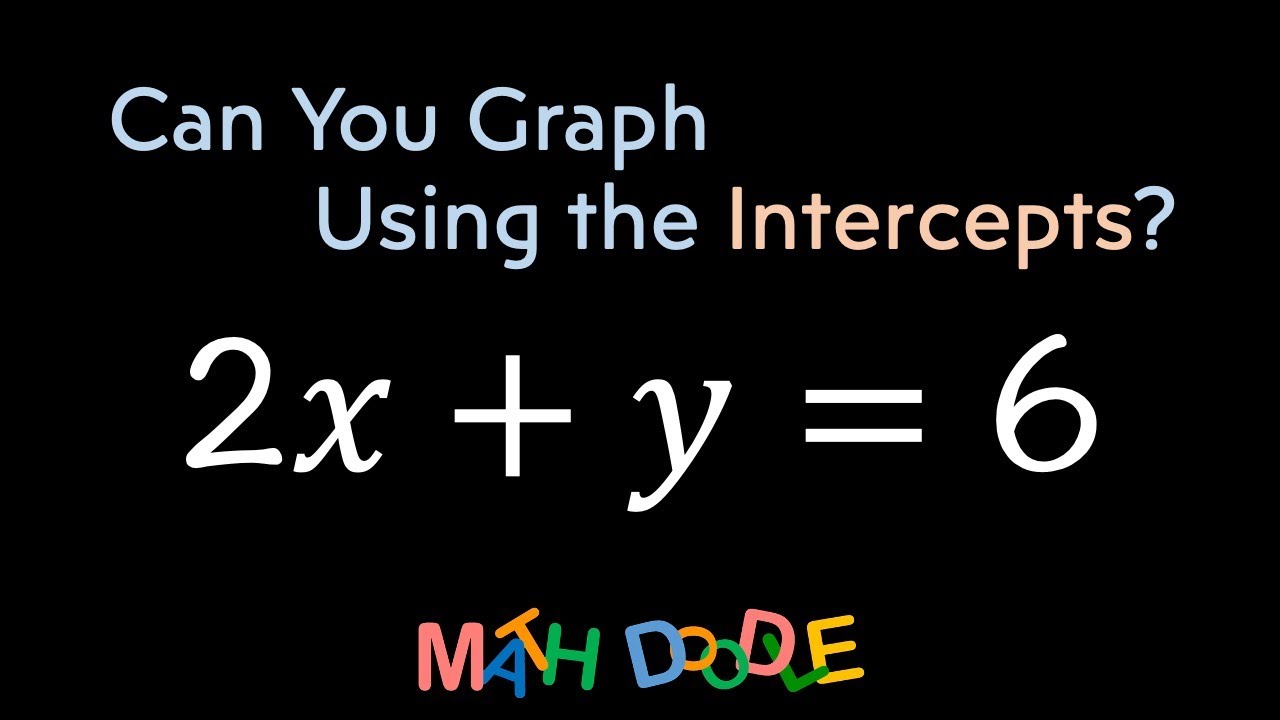
Graphing Linear Equation “2𝑥 + 𝑦 = 6” Using Intercepts | Step-by-Step Algebra - Math Doodle
Показать описание
This video will guide you through [How to Graph Equation “2𝑥 + 𝑦 = 6” Using Intercepts].
📌 How to Graph a Linear Equation Using Intercepts
✓ Step 1. Find the 𝑥- and 𝑦-intercepts of the line.
- To find the 𝑥-intercept: Let 𝑦 = 0 and solve for 𝑥
- To find the 𝑦-intercept: Let 𝑥 = 0 and solve for 𝑦
✓ Step 2. Find a third solution to the equation.
✓ Step 3. Plot the three points and check that they line up.
✓ Step 4. Draw the line.
🔑 Key Things to Remember:
☞ 𝑥-intercept ➜ the point where the line crosses the 𝑥-axis. It has the form (𝑎, 0).
☞ 𝑦-intercept ➜ the point where the line crosses the 𝑦-axis. It has the form (0, 𝑏).
〰️
✅ More BITE-SIZE VIDEOS on "Graphing Linear Equations”
〰️
〰️
〰️
📖 About Math Doodle: This channel is our effort to support students, teachers, and parents by sharing our tutorial and problem-walkthrough contents that cover a wide range of algebra topics!
Hopefully, you have found the math help you were looking for! 🙏 Thank you for checking out our videos! 💯
〰️
🌏 Translated titles and subtitles have been added to this video:
Spanish: Graficar “2x + y = 6” usando intersecciones
French: Représentation graphique de "2x + y = 6" à l'aide d'intersections
German: Grafische Darstellung von „2x + y = 6“ unter Verwendung von Abschnitten
Portuguese: Representação gráfica de “2x + y = 6” usando interceptações
Hindi: इंटरसेप्ट का उपयोग करके "2x + y = 6" रेखांकन करना
Filipino: Pag-graph ng "2x + y = 6" gamit ang Intercepts
Indonesian: Grafik “2x + y = 6” menggunakan Intercepts
Japanese: 切片を使用して「2x + y = 6」をグラフ化する
Russian: График «2x + y = 6» с использованием Intercepts
Vietnamese: Vẽ đồ thị “2x + y = 6” bằng cách sử dụng Intercept
📌 How to Graph a Linear Equation Using Intercepts
✓ Step 1. Find the 𝑥- and 𝑦-intercepts of the line.
- To find the 𝑥-intercept: Let 𝑦 = 0 and solve for 𝑥
- To find the 𝑦-intercept: Let 𝑥 = 0 and solve for 𝑦
✓ Step 2. Find a third solution to the equation.
✓ Step 3. Plot the three points and check that they line up.
✓ Step 4. Draw the line.
🔑 Key Things to Remember:
☞ 𝑥-intercept ➜ the point where the line crosses the 𝑥-axis. It has the form (𝑎, 0).
☞ 𝑦-intercept ➜ the point where the line crosses the 𝑦-axis. It has the form (0, 𝑏).
〰️
✅ More BITE-SIZE VIDEOS on "Graphing Linear Equations”
〰️
〰️
〰️
📖 About Math Doodle: This channel is our effort to support students, teachers, and parents by sharing our tutorial and problem-walkthrough contents that cover a wide range of algebra topics!
Hopefully, you have found the math help you were looking for! 🙏 Thank you for checking out our videos! 💯
〰️
🌏 Translated titles and subtitles have been added to this video:
Spanish: Graficar “2x + y = 6” usando intersecciones
French: Représentation graphique de "2x + y = 6" à l'aide d'intersections
German: Grafische Darstellung von „2x + y = 6“ unter Verwendung von Abschnitten
Portuguese: Representação gráfica de “2x + y = 6” usando interceptações
Hindi: इंटरसेप्ट का उपयोग करके "2x + y = 6" रेखांकन करना
Filipino: Pag-graph ng "2x + y = 6" gamit ang Intercepts
Indonesian: Grafik “2x + y = 6” menggunakan Intercepts
Japanese: 切片を使用して「2x + y = 6」をグラフ化する
Russian: График «2x + y = 6» с использованием Intercepts
Vietnamese: Vẽ đồ thị “2x + y = 6” bằng cách sử dụng Intercept
0:00:46
How to draw graph of the Linear Equation y=2x+3 #math #tutor #mathtrick #learning #shorts #graph
0:00:41
Graphing a Line in Slope-Intercept Form | Graph y=-3/2x+1 | Linear Equations
0:02:30
Graph the line y=2x+1
0:04:05
Graph y=2x-7
0:02:16
Graphing Linear Equation “2𝑥 + 𝑦 = 6” Using Intercepts | Step-by-Step Algebra - Math Doodle...
0:00:49
Graph y=2x + 5 basic algebra
0:02:33
How to Graph y = 2x
0:05:15
Solving Systems of Equations By Graphing
0:46:21
SAT Math on Khan Academy: Algebra (Medium)
0:01:59
How to Graph the Linear Equation 2x + y = 4
0:03:15
Graph the Linear Equation y=2x-3 by Plotting Two Points (and a Third Check Point)
0:01:30
Graph y = -2x + 2 | linear equations
0:32:05
Linear Equations - Algebra
0:02:01
Graph y = 2x + 3
0:02:15
How to Graph the Equation y = 2x - 1
0:01:42
How to Graph 2x+y=4
0:03:24
How to Graph y = 2x + 1
0:08:20
Two-variable linear equations and their graphs | Algebra I | Khan Academy
0:03:05
How to graph y = 2x + 3
0:02:09
Graph a linear equation using a table y = 4 + 2x
0:02:37
How to Graph the Linear Equation 2x - 3y = 6
0:02:26
How to Graph the Equation y = 2x - 6
0:13:10
Graphs of linear equations | Linear equations and functions | 8th grade | Khan Academy
0:03:33
Ex 1: Graph a Linear Equation Using a Table of Values
Комментарии