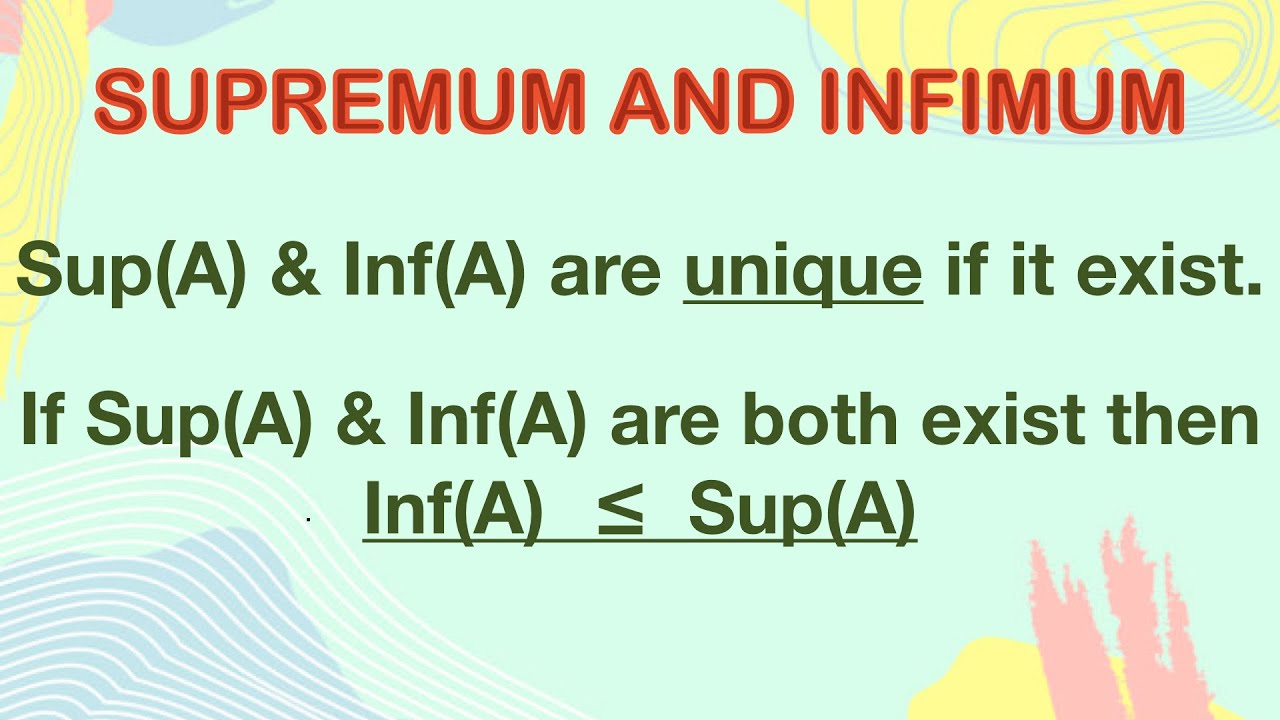
Supremum & Infimum are unique | Property | Real Analysis | glb | lub | upper/lower bound | Msc | Bsc

Supremum & Infimum are unique | Property of Supremum and Infimum | Real Analysis | Math Tutorials.
---------Supremum and Infimum | Definition------------
helpful for Msc | BSC | NET | NBHM | LPU | DU | IIT JAM | TIFR | ISI | BHU | BSc(H), CSIR NET Students.

Topics Covered in playlist Real Analysis:
Definition of Neighbourhood of a point.
Definition of Open set.
infinite intersection of open sets need not to be open
Union of two NBDS is NBD
Intersection of NBDS is NBD
Superset of a NBD is also a NBD
Every Open interval (a,b) is neighbourhood of each of its points.
Closed interval is neighbourhood of each point except end points.
real numbers is NBD of each real number
Rational numbers set is not the neighbourhood of any of its points.
Metric space | Distance Function | Example
Metric space : Definition and Axioms
Real Analysis : Introduction and Intervals
Union of countable sets is countable
Finite,infinite,equivalent,denumerable,countable sets
Infinite subset of countable set is countable
Field,Ordered Field,complete Ordered Field
Set of Integers is Countable
Supremum and infimum
Set is countably infinite iff it can be written in the form distinct elements
Continuum Hypothesis
Cartesian product of two countable sets is Countable
Set of Rational numbers is Countable

--------------------------Thanks for Watching-------------------------------------

Definition of metric Space
Examples of metric space
Open and Closed sets
Topology and convergence
Types of metric spaces
Complete Spaces
Bounded and complete bounded spaces
Compact spaces
Locally compact and proper spaces
connectedness
Separable spaces
Pointed Metric spaces
Types of maps between metric spaces
continuous maps
uniformly continuous maps
Lipschitz-continuous maps and contractions
isometries
Quasi-isometries
notions of metric space equivalence
Topological properties
Distance between points and sets
Hausdorff distance and Gromov metric
Product metric spaces
Continuity of distance
Quotient metric spaces
Generalizations of metric spaces
Metric spaces as enriched categories

Supremum and infimum of sets
sup and inf. value
least upper bound and greatest lower bound.
l.u.b. and g.l.b.
this concept will be helpful for the further study of real analysis.
Real analysis intro and intervals
complete knowledge for bounded and unbounded intervals.
LPU distance MA maths.
LPU msc. maths
Msc maths
Ma maths
Bsc maths

Basic Topology
Topology mathematics
topology maths
Topology optimization
Topology lecture
Topology and types
Topology axioms

Real analysis for BA maths
Real analysis for MA Maths
Real analysis for Msc. Maths
Real analysis study material
Real analysis books
Real Analysis notes

Real Analysis Basic Concept

Real analysis topics
Real analysis course
Real analysis applications
Real analysis lacture notes
Real analysis proofs
Real analysis text book
Real analysis MIT open course
LPU MA maths sylalbus
LPU ma maths study material
LPU MA maths solution
LPU ma maths 1st year
LPU MA maths books
LPU MA maths sample questions
LPU distance education.

LPU MA Math
LPU msc. maths
LPU msc. math

lovely professional univesity MA maths sylalbus
lovely professional univesity ma maths study material
lovely professional univesity MA maths solution
lovely professional univesity ma maths 1st year
lovely professional univesity MA maths books
lovely professional univesity MA maths sample questions
lovely professional univesity distance education.
lovely professional univesity MA Math
lovely professional univesity msc. maths
lovely professional univesity msc. math

Ma maths
real analysis nptel
real analysis mit
real analysis iit jam
real analysis in tamil
real analysis upsc
real analysis mathematics
real analysis
real analysis axioms
real analysis book
real analysis bsc maths part 2
real analysis bsc maths in hindi
real analysis b tech maths
bounded intervals on the real number line
bounded and unbounded intervals
Denumerable union of Denumerable set is Denumerable
Denumerable union of Denumerable sets is Denumerable
union of Denumerable sets is Denumerable
a Denumerable union of Denumerable sets is Denumerable
a countable union of countable sets is countable

discrete math
countable sets examples
countable sets and uncountable sets
countable sets in real analysis
countable sets proof
countable sets problems
countable and uncountable sets discrete mathematics
countable set in real analysis
countable and uncountable sets in discrete mathematics
what is countable and uncountable sets

finite sets and infinite sets
finite sets meaning
finite sets definition
examples of finite sets(mathematics)

[inmathsstar]

Aur monotone sequence ki limit kysy find out krty hn yeh b guide kr den

[shwetaSingh-ftfi]

Mam (5) singleton set hai. Iska infimum any supremum dono 5 hi Hoga na. Please bataya

[nasaworld-zquw]

Proof ko contradiction mh bhe kr skty ha?

[KANCHANKUMARI-euls]

Mam sab aachhe se samag aaya but suprimum, infimum se hamesa bada hoga to less than equal kyo sirf less than kyo nahi.clear kijiye mam🙏🙏🙏

[inmathsstar]

Mam kindly supremum & infrimum of sequence k questions ki video bna dyn

[sakshisahu]

Mam please make a video of a+u<= m where m is upper bound of a+ s

[dikshadhar]

thank youuuu so are a life saver..subscribed to ur channel...

[shamasnaveed]

thank u much ma'am u made this topic so easy thnx God bless you keep it up

[anasumair]

In which conditions infimum of set is equal to supremum of set

[abusufyan]

If t is bounded above sup t exists and belong to t. If t is subset of z

[bhumikaagarwal]

Mam infimum supremum k equal kaise hoga ?
Less than case to samjh as gya but equal k koi example..

[sakshisahu]

Mam please proof the theorem of if supremum of s and t are known then sup(s+ t) =sups +supt