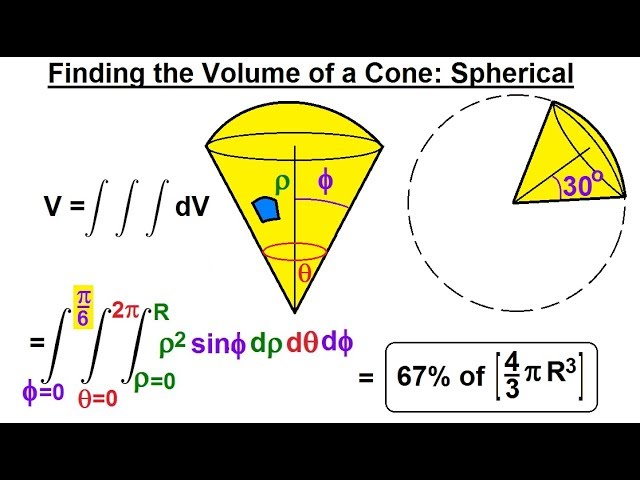
Calculus 3: Triple Integrals (6 of 25) Finding the Volume of a Cone: Part 1: Spherical

In this video I will find volume of a cone using triple integrals in the spherical coordinate system.

Next video in this series can be seen at:

[fadlihafni]

thank youu, your explanation is clear as crystal, wish me luck for upcoming examm

[offxbyxone]

How do you get the same volume for the cone as you would get for just the spherical cap at pi/6?.... he set both of those integrals up the same way....

[harry_page]

I just tried working out the volume of a cone with a flat base, is my working out correct?
I defined the cone's height as h and the angle inside as alpha, so that its base has the Cartesian equation z=h. Since z=rho*cos(phi), we have rho=h/cos(phi) so the limits of the rho integral will be between zero and h/cos(phi).
Then while evaluating the integrals I got 2/3*pi*h^3* integral from 0 to alpha of tan(phi)*sec^2(phi)*d(phi)
The integral evaluates to 1/2*tan^2(phi) if I'm not mistaken, using inspection/reverse chain rule
So we have 1/3*pi*h^3*tan^2(alpha) and since tan(alpha)=r/h where r is the radius of the base of the cone, this simplifies to 1/3*pi*h*r^2. That's interesting, a third of the volume of a cylinder of the same height and base radius

[thomasolson]

I did pi/12 and 2R. For the spherical version of the inscribed angle. It's a little over twice the volume.

Maybe I'm wrong, I don't know. I like how the R and the pi goes poof and we're left with a bunch of symbols.
Thanks for this.

[vineetjain]

Sir I have tried it out but instead of sin phi I am getting cos phi in the volume element and It justifies our reasoning as now when phi=0 we have more width(twisting) while at the lower point we have less twist which is not verified by sin phi in volume element. Also it will be very nice of you If you can make a video on Deriving volume of cone using spherical coordinates. I try hard but eventually cant able to derive via spherical coordinates.

[lordyabo]

Sir, is there a time when the cone is part of a cylinder not sphere? and does the dv of that cone is equal to the dv for cylindrical coordinates?

[dinamustafa]

how do you determine the angle 30 degree for phi ?

[Channel_Math_and_Physics]

Wait shouldn't phi be -π/6 to π/6

[ako]

It is not well-defined if the radial component of the integral dp is going to full radius or only up to the height of the cut-out disk.