filmov
tv
Complex exponentials spin

Показать описание
When we put time up in the exponent of a complex exponential, the complex number it represent rotates in a circle on the complex plane. You can think of it as a spinning number!
Complex exponentials spin
Electrical engineering - Complex exponentials spin
Complex exponentials spin
What is a Complex Exponential?
Eulers formula magnitude
e^(iπ) in 3.14 minutes, using dynamics | DE5
The complex exponential
Complex Exponentials and Trig Identites
Fourier Transforms || Theoretical Interpretations, Complex Exponentials and Window Effect
Complex Analysis Episode 12: The Complex Exponential Function
Complex Exponentiation
Signals: Complex Exponentials
SUM of N COMPLEX exponentials
sum of two complex exponentials
Rant: The Glory of Complex Exponentials
cos θ in terms of complex exponentials (Exam Question 9 of 12)
L7 1 3Review The Complex Exponential
Lecture 8 Module 2 Complex Exponentials Examples
2D Rotations and Complex Exponentials
Complex exponents geometric approach
Visualization of a Continuous-Time Periodic Complex Exponential Signal
Physics123 Complex Exponentials Video
Complex exponential mapping
Math 344 - Complex exponentials
Комментарии
 0:06:40
0:06:40
 0:06:40
0:06:40
 0:06:40
0:06:40
 0:00:53
0:00:53
 0:06:10
0:06:10
 0:04:08
0:04:08
 0:06:46
0:06:46
 0:05:08
0:05:08
 0:19:07
0:19:07
 0:04:30
0:04:30
 0:15:21
0:15:21
 0:03:37
0:03:37
 0:05:48
0:05:48
 0:04:26
0:04:26
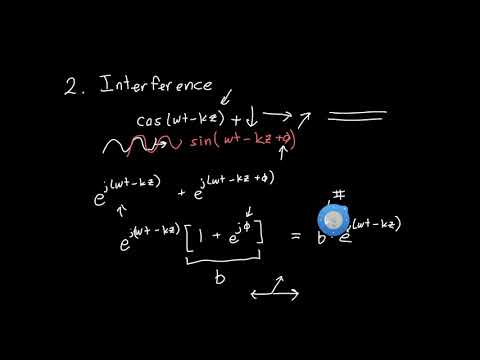 0:07:46
0:07:46
 0:04:08
0:04:08
 0:04:46
0:04:46
 0:17:02
0:17:02
 0:19:19
0:19:19
 0:11:42
0:11:42
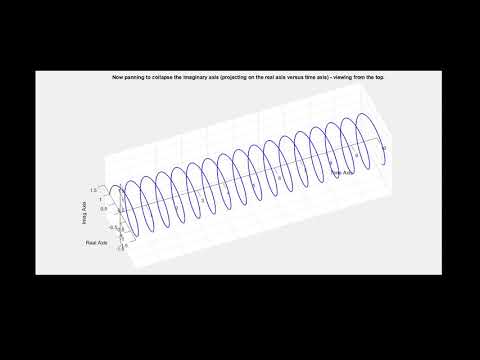 0:01:35
0:01:35
 0:20:36
0:20:36
 0:06:31
0:06:31
 0:24:51
0:24:51