Rouche's Theorem with Example | Complex Analysis #23

Показать описание

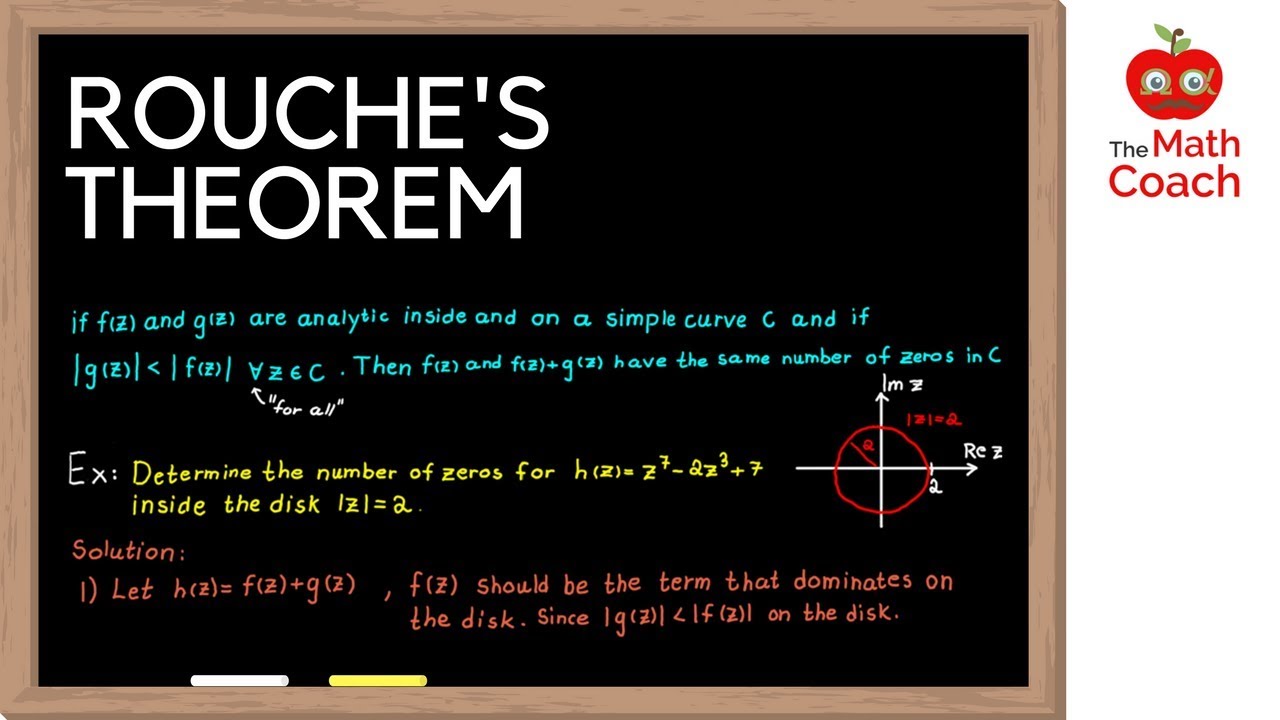
Rouche's theorem states that if two functions f(z) and g(z) are analytic inside and on a simple closed curve C and if |g(z)| smaller than |f(z)| for all z on C, then f(z) and f(z) + g(z) have the same number of zeros inside C (counting multiplicity).

The theorem is really useful when determining the number of zeros for complex functions in a certain region which the video will illustrate with an example.

LINK TO COMPLEX ANALYSIS PLAYLIST

SUPPORT
Consider subscribing and liking if you enjoyed this video or if it helped you understand the subject. It really helps me a lot.

NOTE - DIFFERENT VERSIONS OF THE THEOREM
Rouche's Theorem is actually an consequence of a stronger theorem called "symmetric Rouché's theorem". This symmetric version of the theorem is the reason you can find many different "Rouche's Theorem" online, you might recognise the following forms the theorem has taken:

1) |f(z) - g(z)| smaller than |f(z)|
2) |f(z) + g(z)| smaller than |f(z)|
3) |f(z) - g(z)| smaller than |f(z)| + |g(z)| (Symmetric Rouche's Theorem).

But the one I'm using in the video is the original theorem and is not from the symmetric version of it.

NOTE - HOW TO USE THE THEOREM TO SOLVE PROBLEMS
The most important thing to remember when solving this type of problem is that: Choose f(z) and g(z) so that f(z) + g(z) is equal to the function you want to analysis and that |g(z)| is smaller than |f(z)| on the curve.

In this example, the function f(z) only needed to be one of the terms from the original function, but you should know that sometimes you will need two or even more terms, otherwise, the condition that |f(z)| should be bigger than |g(z)| on the curve might not be fulfilled.

EDIT
Note that I refer to the region as the disk |z| = 2. I would like the watcher to think about the disk as a circle instead since you can't really be "inside and on" a disk. So in short I should have used the word "circle" instead of "disk" when making the video from the start, but I realized this mistake in the later part of the creation process.

Note that then we determine the absolute value of g(z) at 2:40 we are using "Triangle Inequality", which states that the absolute values of a complex number is always smaller or equal to the sum of the absolute value of each term that makes up the number, hence: |z_1 + z_2| smaller or equal to |z_1| + |z_2|. So the right expression at 2:40 should be: |g(z)| smaller or equal to |7|+|-2z^3| = 7+2^4 = 23.

TIMESTAMPS
00:00 Rouche's Theorem
00:32 Example

SOCIAL

HASHTAGS
#TheMathCoach #ComplexAnalysis #Complex Analysis Playlist

Рекомендации по теме

Комментарии

Great video! I did note one error: at minute 2:50, you claim that |g(z)| = |7-2z^3| = |7|+|-2z^3|, when instead it should be |g(z)| = |7-2z^3| <= |7|+|-2z^3| by triangle inequality. Aside from that, excellent video! I'll be watching more!

sethlovelace

You don't know how much you've helped me

matloose

You don't need something as powerful as Rouche's theorem to solve the problem mentioned in the second half of this video. To use that theorem here is an overkill, like killing mosquitoes with cannons.

AA-lels

It would be better if you had added some more examples with theorem though thanks for the hard work!

krisnendubiswas

So when doing rational root tests, we can toss out any number greater then 2. Interesting.

ingiford

Thank you Sir, for crystal clear explanation

devashish

If only I had seen this video a couple of weeks ago... ;) very clear explanation....all the best...

sivatvs

Another phenomenal video! Now I know how to appRouche these types of problems...

PunmasterSTP

You might be mistaken at 3mins. |g (z)| = |7-2z^3| =/= |7| + |-2z^3|... you mightve meant the Triangle Inequality.

davidjohnson-mysr

Sir, please solve more examples, where contour is like, 1<= |z|<2

richasharma

At an exam today I misread my own (allowed) notes and thought |f(z)| had to be bigger on _and_ _inside_ C. Made it SO much harder (...impossible, even) for myself for no goddamn reason lol

metallsnubben

Is it generally difficult/tedious to find how many number of roots in a specific quadrant? I had a few tutorial questions which asked for how many roots in each quadrant.

gnydnnk

Sir please btayeye ki hmesa ek hi term kyu lete hai as f(z)

shivanshirathore

Rouche's Theorem with Example | Complex Analysis #23

Rouche's Theorem with Example | Complex Analysis #23

How many roots? Rouché's Theorem

How to use Rouches Theorem to find the zeros in Complex Analysis

Rouches Theorem Example

Rouche's Theorem and the Fundamental Theorem of Algebra

Rouche's Theorem Problems and solutions 🔥 | Problem 1

Rouche's Theorem

Rouche's theorem proof and examples

GATE Mathematics - 2022 | Complex Analysis; Q - 29 | Rouche's Theorem | #mathtecz

Lecture 12 part (1) \\ Examples Of Rouche,s Theorem;Complex Analysis

Rouche's Theorem with Proof | Complex Analysis #24

Msc complex analysis notes (ROCHES THEOREM) 2nd unit

ROUCHE'S THEOREM PROBLEMS AND SOLUTIONS - 3

Rouche's theorem / Complex analysis / Mathematics/M.Sc-1st sem... #short_video

Rouche's Theorem in Complex Analysis | MSc Math | Rouche's Theorem with Examples in Hindi

Rouche's Theorem

Argument Principle and Rouche`s Theorem with Numerical Example

Lecture 11 \\ Examples of Rouche,s Theorem;Complex Analysis

Identity Theorem,Rouche Theorem,Open Mapping Theorem-2(IMP Note/Rouche's Theorem with Examples)

Polytechnic trb maths / Complex analysis /Rouche theorem & Problem

Rouche's theorem proof with examples

Lecture 12 part(2) \\ Examples of Rouche,s Theorem;Complex Analysis

Rouche's Theorem With Complete Proof in Complex Analysis-II

Lecture 10 \\ Examples Of Rouche,s Theorem;Complex Analysis