filmov
tv
Greg Galloway: Topology & General Relativity 1

Показать описание
Summer School »Between Geometry and Relativity«, Part 10: Greg Galloway: Topology & General Relativity 1
Abstract: An initial data set in spacetime consists of a spacelike hypersurface $V$, together with its its induced (Riemannian) metric h and its second fundamental form $K$. A solution to the Einstein equations influences the curvature of $V$ via the Einstein constraint equations, the geometric origin of which are the Gauss-Codazzi equations. After a brief introduction to Lorentzian manifolds and Lorentzian causality, we will study some topics of recent interest related to the geometry and topology of initial data sets. In particular, we will consider the topology of black holes in higher dimensional gravity, inspired by certain developments in string theory and issues related to black hole uniqueness. We shall also discuss recent work on the geometry and topology of the region of space exterior to all black holes, which is closely connected to the notion of topological censorship. Many of the results to be discussed rely on the recently developed theory of marginally outer trapped surfaces, which are natural spacetime analogues of minimal surfaces in Riemannian geometry.
Greg Galloway is professor at the Department of Mathematics, U of Miami.
1. Lead 00:00:00
2. Lorentzian Causality 00:00:10
3. Achronal Boundaries 00:11:42
4. Causality conditions 00:21:08
5. Global hyperbolicity and Cauchy hypersurfaces 00:26:43
6. Domains of dependence 00:41:44
Abstract: An initial data set in spacetime consists of a spacelike hypersurface $V$, together with its its induced (Riemannian) metric h and its second fundamental form $K$. A solution to the Einstein equations influences the curvature of $V$ via the Einstein constraint equations, the geometric origin of which are the Gauss-Codazzi equations. After a brief introduction to Lorentzian manifolds and Lorentzian causality, we will study some topics of recent interest related to the geometry and topology of initial data sets. In particular, we will consider the topology of black holes in higher dimensional gravity, inspired by certain developments in string theory and issues related to black hole uniqueness. We shall also discuss recent work on the geometry and topology of the region of space exterior to all black holes, which is closely connected to the notion of topological censorship. Many of the results to be discussed rely on the recently developed theory of marginally outer trapped surfaces, which are natural spacetime analogues of minimal surfaces in Riemannian geometry.
Greg Galloway is professor at the Department of Mathematics, U of Miami.
1. Lead 00:00:00
2. Lorentzian Causality 00:00:10
3. Achronal Boundaries 00:11:42
4. Causality conditions 00:21:08
5. Global hyperbolicity and Cauchy hypersurfaces 00:26:43
6. Domains of dependence 00:41:44
Комментарии
 0:48:43
0:48:43
 0:43:54
0:43:54
 0:43:10
0:43:10
 0:54:26
0:54:26
 0:50:50
0:50:50
 1:01:28
1:01:28
 0:00:57
0:00:57
 0:44:04
0:44:04
 0:05:11
0:05:11
 1:31:09
1:31:09
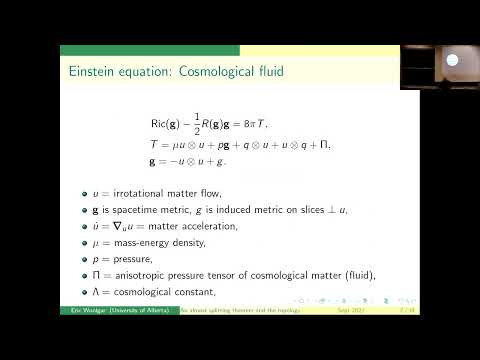 0:59:53
0:59:53
 0:00:07
0:00:07
 0:00:44
0:00:44
 0:02:15
0:02:15
 0:57:48
0:57:48
 1:10:51
1:10:51
 0:10:40
0:10:40
 0:10:39
0:10:39
 0:45:32
0:45:32
 0:32:15
0:32:15
 1:13:00
1:13:00
 0:58:31
0:58:31
 0:00:44
0:00:44
 0:16:27
0:16:27