filmov
tv
Complex number : - ( proof of De moivre's theorem by Euler's formula ) - 48.

Показать описание
Complex number is a number that can be expressed in the form
of a + bi. where a and b are real numbers, and i is a square root
of -- 1. Complex numbers can be called as imaginary numbers.
De Moivre's theorem :
( Cos x + i Sin x ) power n = Cos (nx ) + i Sin (nx)
Proof of De moivre's theorem by Euler's formula.
Please Subscribe our YouTube channel for latest Uploads.
of a + bi. where a and b are real numbers, and i is a square root
of -- 1. Complex numbers can be called as imaginary numbers.
De Moivre's theorem :
( Cos x + i Sin x ) power n = Cos (nx ) + i Sin (nx)
Proof of De moivre's theorem by Euler's formula.
Please Subscribe our YouTube channel for latest Uploads.
Complex Numbers: Operations, Complex Conjugates, and the Linear Factorization Theorem
Introduction to complex numbers | Imaginary and complex numbers | Precalculus | Khan Academy
Necessity of complex numbers
Complex number fundamentals | Ep. 3 Lockdown live math
Imaginary Numbers Are Real [Part 1: Introduction]
Do Complex Numbers Exist?
Complex Numbers 1(Definition, Addition, Subtraction, Multiplication and Division of Complex Numbers)
Complex Numbers - Basics | Don't Memorise
HP TGT Commission Mathematics: Polar form of Complex Number | Most Important Questions
What is a Complex Number
How Imaginary Numbers Were Invented
Complex Number || LS & GS
Plus One Christmas Exam | Maths -Complex Numbers and Quadratic Equations | Conic Sections | Xylem +1
What is 'above' the complex numbers??
Complex Numbers In Polar - De Moivre's Theorem
Simplifying Complex Numbers
What are complex numbers? | Essence of complex analysis #2
007 – ALEVEL PURE MATHEMATICS| COMPLEX NUMBERS (ALGEBRA)| FOR SENIOR 5 & 6
Pre-Calculus - Learn the basic operations of complex numbers
COMPLEX NUMBERS | How to Add, Subtract, Multiply and Divide Complex Numbers
Introduction to Complex Numbers (1 of 2: The Backstory)
Solving Equations With Complex Numbers
Adding and Subtracting Complex Numbers
How to find the principal square root of a complex number
Комментарии
 0:08:35
0:08:35
 0:04:44
0:04:44
 0:07:39
0:07:39
 1:22:11
1:22:11
 0:05:47
0:05:47
 0:11:26
0:11:26
 0:40:02
0:40:02
 0:03:36
0:03:36
 0:58:12
0:58:12
 0:01:00
0:01:00
 0:23:29
0:23:29
 1:00:30
1:00:30
 3:33:16
3:33:16
 0:00:59
0:00:59
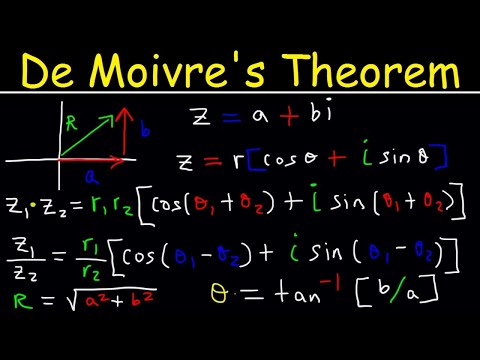 1:04:47
1:04:47
 0:05:57
0:05:57
 0:32:11
0:32:11
 2:57:56
2:57:56
 0:10:46
0:10:46
 0:14:58
0:14:58
 0:09:10
0:09:10
 0:02:07
0:02:07
 0:02:36
0:02:36
 0:00:59
0:00:59