filmov
tv
39. Frenet Frame and Arclength - Part 2 - Proofs of Some Vector Identities
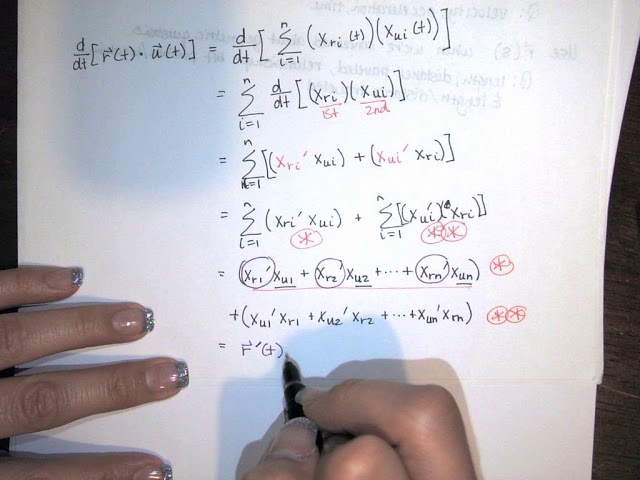
Показать описание
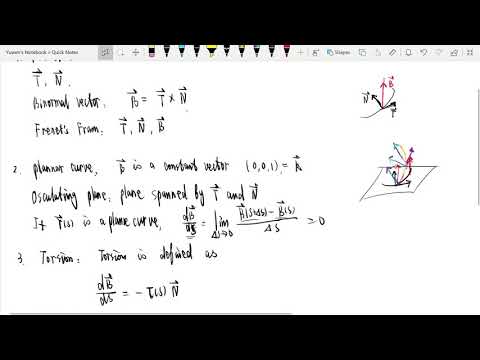
In this video, we prove two vector identities that have implications for the unit tangent, principle unit normal, and binormal vectors. First, we prove a generalization of the product rule for the dot product of two vector functions. Then we use that fact to prove that if the length of a vector is constant, the vector itself is orthogonal to its derivative.
We'll rely on this identity when we define the principal unit normal and binormal vectors, and discuss the tangential and normal components of acceleration.
We'll rely on this identity when we define the principal unit normal and binormal vectors, and discuss the tangential and normal components of acceleration.
 0:16:55
0:16:55
 0:51:59
0:51:59
 0:38:31
0:38:31
 0:06:57
0:06:57
 0:07:48
0:07:48
 0:02:17
0:02:17
 0:07:25
0:07:25
 0:05:23
0:05:23
 0:02:51
0:02:51
 1:17:27
1:17:27
 0:03:00
0:03:00
 0:39:31
0:39:31
 0:05:39
0:05:39
 0:18:13
0:18:13
 0:06:27
0:06:27
 0:49:02
0:49:02
 0:23:39
0:23:39
 0:14:41
0:14:41
 0:09:33
0:09:33
 0:24:39
0:24:39
 0:17:36
0:17:36
 0:00:06
0:00:06
 0:03:33
0:03:33
 0:21:55
0:21:55