Calc 1, Lec 23: Percent Change in the Period of Mass on a Spring, Minimize Cylinder Surface Area

Local linearization can be used to estimate the percent change in a dependent variable for a given percent change in an independent variable. Our first optimization problem is analyzed as well.

(0:00) Lecture plan.
(0:41) Applications to come for the rest of the semester (Families of functions, optimization, differential equations applications, data modeling, related rates, more on the physics of motion, flow rates and accumulated flow (both for fluids and for cash flows, including discounted cash flow, based on present values)).
(4:36) Theorems will be discussed on "as needed" and "natural" way.
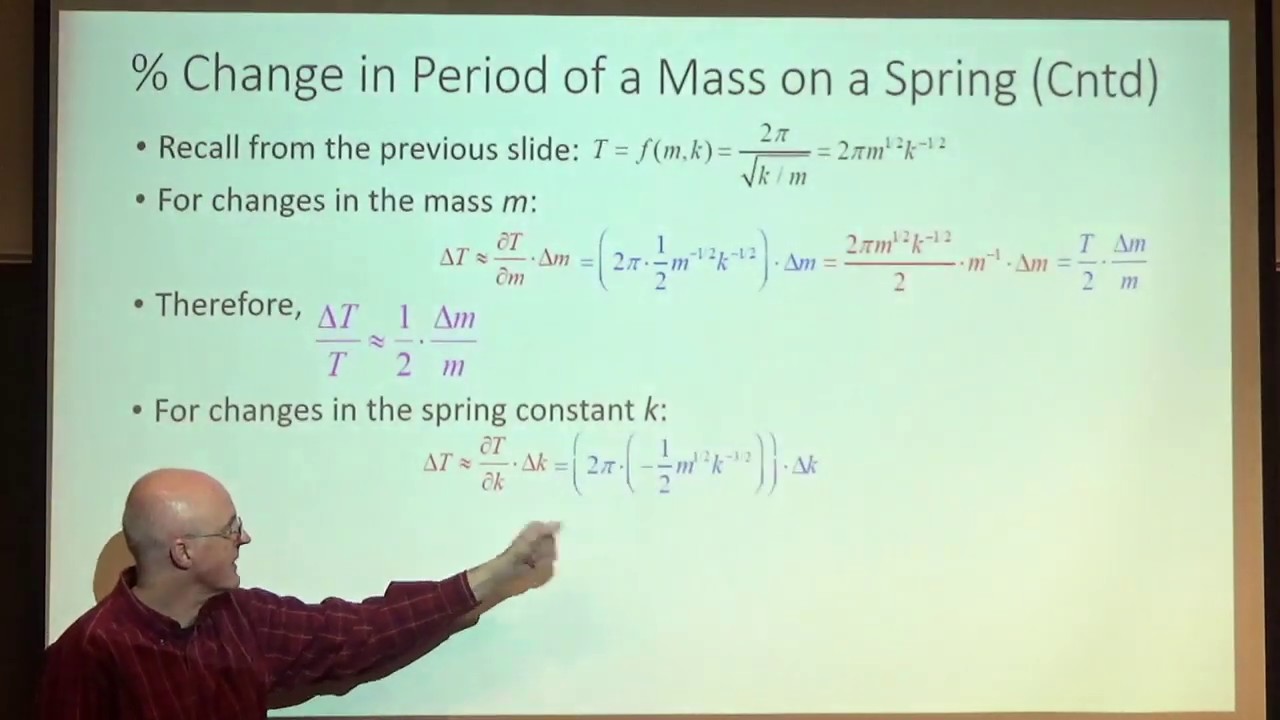
(7:48) Use local linearization to estimate relative (percent) changes of the period of a mass on a spring, based on relative changes in the mass m and the spring constant k.
(18:46) Confirm the approximations on Mathematica.
(26:10) Optimization problem: find the dimensions of a soup can (cylinder) that minimize the surface area when the volume is an arbitrary constant c. Describe the problem as minimizing an objective function subject to a constraint equation. Show the steps to solve it and find the values of the radius and height that give the minimum surface area.
(46:18) Confirm that it is a minimum. First, use the Second Derivative Test by doing a calculation to show the graph is concave up. Then verbally describe how to think about the First Derivative Test (and confirm it mentally without doing calculations).
(53:04) Use Mathematica to find the minimum surface area. Also graph the surface area function when the fixed volume is 500 cubic centimeters, showing the critical point on the plot.