filmov
tv
Complex Analysis: Fancy Branch Cuts

Показать описание
Today, we evaluate the integral of log(x^4+1)/(x^2+1) from -infinity to infinity using contour integration.
Complex Analysis: Fancy Branch Cuts
Complex Workshop - Riemann surfaces and branch cuts (chalkboard test video)
EX: Branch cut of the product of two square roots
Complex Analysis: Logarithms and Branch Cuts
Complex Logarithm, Branch Points, and Branch Cuts
Branch Points and Branch Cuts
Branch Point and Branch Cut |Multi valued Functions in Complex Analysis|Part 1|Theta Classes
Branch cuts Examples
Complex Analysis: Double Keyhole Contour
Branch cut of Log functions || Complex Analysis
(PCb22) Intro. to Branch Cuts and Branch Points
Branch point and Branch cut
logarithmic Integration (Branch cut)
2.5 Evaluating definite integrals. Integrands with branch cuts. Part 1
Branch points and branch cuts
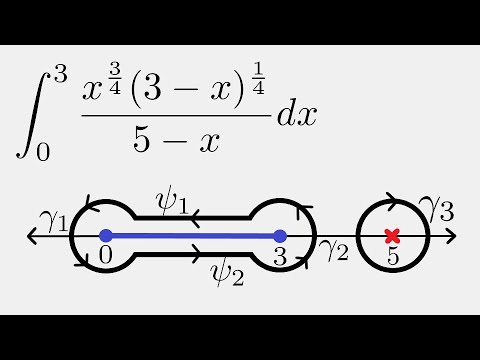
[CA/Week 6] 1. Integrals with power-type integrand and two branch points, Part I.
4.2 Multivaluedness and branches
Complex Analysis: Nasty Integral with Elegant Solution
Complex Analysis: Lecture 3: branch cuts, complex exponential
[CA/Week 4] 3. Extraction of the regular branch of the power type function [example]
Complex Analysis: An Integral from @MichaelPennMath
Complex Analysis: Dogbone Contour Example
Complex Analysis: Integral of log(x^2+1)/x^2
Complex Analysis: Is this solution way too overkill?
Комментарии
 0:48:28
0:48:28
 0:16:13
0:16:13
 0:10:43
0:10:43
 0:34:55
0:34:55
 0:37:50
0:37:50
 0:09:26
0:09:26
 0:45:03
0:45:03
 0:15:58
0:15:58
 0:41:33
0:41:33
 0:15:45
0:15:45
 0:35:54
0:35:54
 0:21:00
0:21:00
 0:11:42
0:11:42
 0:13:01
0:13:01
 0:14:30
0:14:30
![[CA/Week 6] 1.](https://i.ytimg.com/vi/Dqo7Dt8qK5Q/hqdefault.jpg) 0:09:48
0:09:48
 0:50:14
0:50:14
 0:24:48
0:24:48
 0:55:02
0:55:02
![[CA/Week 4] 3.](https://i.ytimg.com/vi/WndfyM7bYck/hqdefault.jpg) 0:11:36
0:11:36
 0:34:00
0:34:00
 0:58:10
0:58:10
 0:34:36
0:34:36
 0:25:14
0:25:14