filmov
tv
Differential Geometry: Lecture 15 part 3: Gaussian and Mean curvature
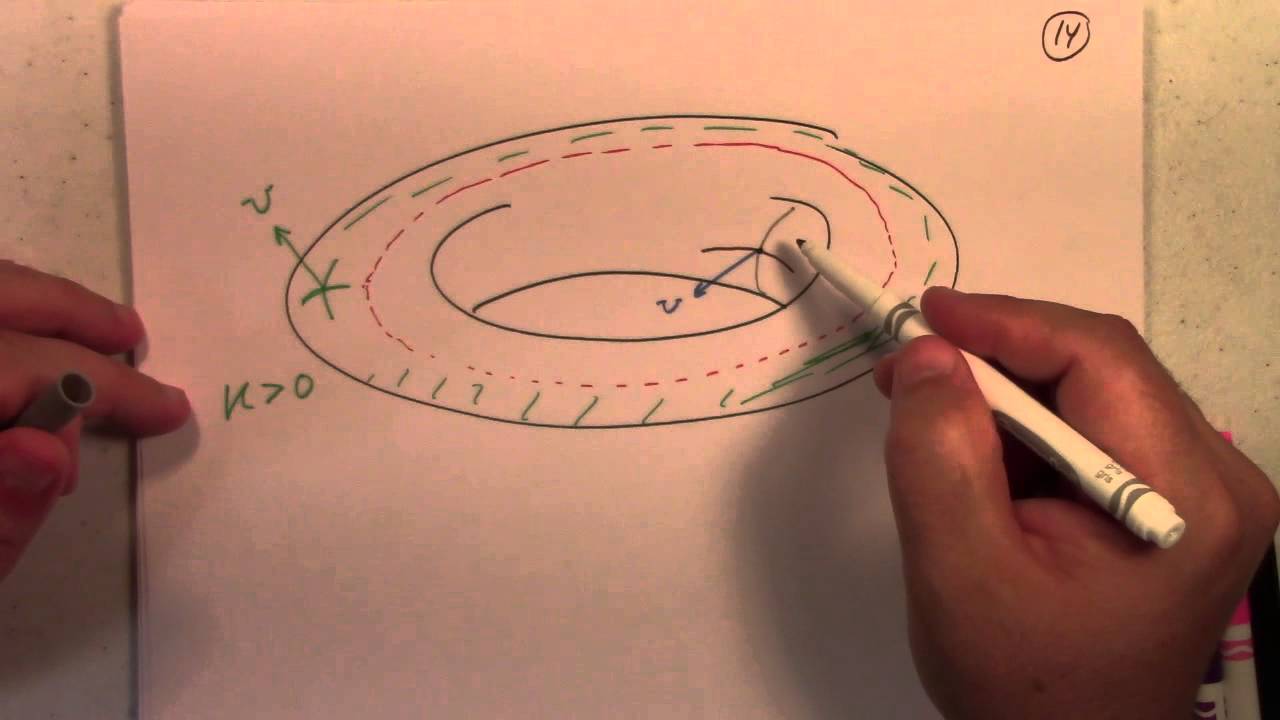
Показать описание
The determinant and trace of the shape operator are used to define the Gaussian and mean curvatures of a surface. We also derive Lagrange's identity and use it to derive a pair of involved formulas which are needed for the alphabet soup of the next section. Finally, the terms "flat" and "minimal" are introduced. However, minimal is not motivated or explained here (see Section 3D of Kuhnel for a really interesting discussion with neat examples)
Differential Geometry: Lecture 15 part 1: Shape Operator Defined
Differential Geometry: Lecture 15 part 2: normal curvature
Differential Geometry: Lecture 15 part 3: Gaussian and Mean curvature
Introduction to differential geometry - Lecture 15 - Prof. Alan Huckleberry
Differential Geometry (MTH-DG) Lecture 15
Differential geometry lecture 15
Differential Geometry in Under 15 Minutes
TORSION || The Theory of Space Curve || Differential Geometry || Lecture -15
Math371-15 - Differential Geometry of Curves and Surfaces
Differential Geometry Lec 15
Differential Geometry 15 : The Lie product - part 2
Lecture 15: Differential Geometry
Differential geometry and topology in physics: Lecture 15
Analysis II Lecture 15 Part 2 flows on manifolds
Intro to General Relativity - 15 - Differential geometry: Curves, functions and vectors
BERTNARD CURVES Differential Geometry Lecture 15 by Dr Sanjeev Rana
When mathematicians get bored (ep1)
How REAL Men Integrate Functions
Lecture: 15 Helix (definition)//curves in space // differential Geometry
Beauty of the Brain😍 IQ - IIT Bombay
Analysis II Lecture 15 Part 3 Triangulations and the Euler characteristic
Symplectic geometry & classical mechanics, Lecture 15
Differential Geometry 15: Lie transport, Exterior algebra
Differential Geometry Lecture 1 (Part 4): Basic notations, tangent spaces, vector fields
Комментарии
 0:41:05
0:41:05
 0:35:05
0:35:05
 0:38:50
0:38:50
 1:17:53
1:17:53
 1:09:29
1:09:29
 0:16:38
0:16:38
 0:13:37
0:13:37
 0:35:33
0:35:33
 0:53:34
0:53:34
 0:33:33
0:33:33
 0:26:13
0:26:13
 0:20:43
0:20:43
 2:34:10
2:34:10
 0:10:31
0:10:31
 0:47:30
0:47:30
 0:18:12
0:18:12
 0:00:37
0:00:37
 0:00:35
0:00:35
 0:03:00
0:03:00
 0:00:19
0:00:19
 0:15:03
0:15:03
 1:19:33
1:19:33
 2:01:41
2:01:41
 0:18:26
0:18:26