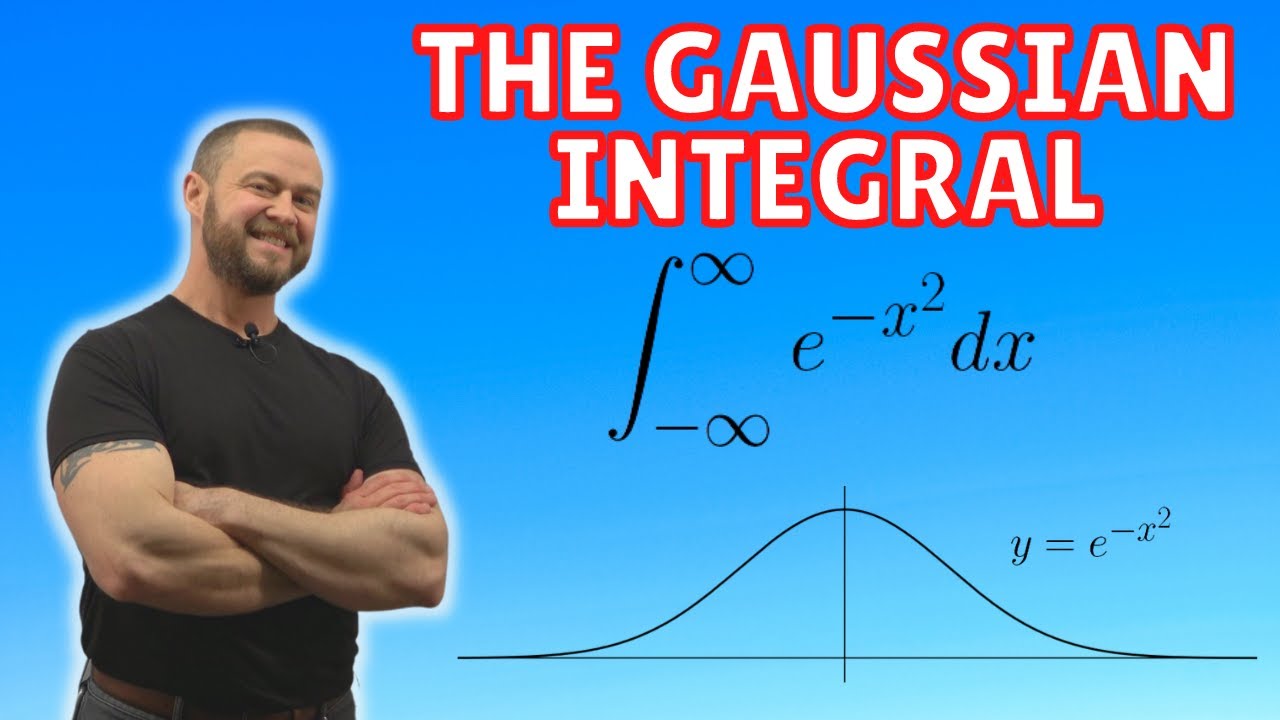
The Gaussian Integral

preview_player
Показать описание
The Gaussian integral is the simplest difficult integral in mathematics. Most difficult integrals require special methods (tricks) and the Gaussian integral is no exception. Here we use the standard trick that every student should know: converting to a double integral and using polar coordinates. While the Gaussian integral is incredibly important we focus in this video on just evaluating it.

Looking for a specific problem or topic? Try checking my website:

► Artist Attribution
Music By: "After The Fall"
Track Name: "Pieces"
Music Published by: Chill Out Records LLC
License: Creative Commons Attribution 4.0 International (CC BY - 4.0)
  1. BlackTshirtMathProfessor
  2. Integral fo e^-x^2 from - infinity to infinity
  3. Gaussian integral
  4. The Gaussian Integral
  5. Calculus
  6. Mathematics
Рекомендации по теме
The Gaussian Integral 0:10:09

The Gaussian Integral

The Gaussian Integral 0:07:52

The Gaussian Integral // Solved Using Polar Coordinates

how Laplace solved 0:15:01

how Laplace solved the Gaussian integral

Why does pi 0:05:45

Why does pi show up here? | The Gaussian Integral, explained

Solving the Gaussian 0:09:39

Solving the Gaussian Integral the cool way

The Gaussian Integral 0:13:31

The Gaussian Integral

Why π is 0:24:46

Why π is in the normal distribution (beyond integral tricks)

Proof of the 0:01:26

Proof of the Gaussian integral in less than 1 minute

Destroying the Gaussian 0:15:43

Destroying the Gaussian Integral using Papa Leibniz and Feynman

The Gaussian Integral 0:24:05

The Gaussian Integral is DESTROYED by Feynman’s Technique

A crazy approach 0:11:04

A crazy approach to the gaussian integral using Feynman's technique

How Gauss solved 0:08:15

How Gauss solved the integral of e^(-x^2) from 0 to infinity

Riemann sums on 0:00:11

Riemann sums on the Gaussian integral

A different approach 0:10:48

A different approach to the Gaussian integral

Gaussian Integral [Int{e^-x^2} 0:14:03

Gaussian Integral [Int{e^-x^2} from -inf to inf]

A simple proof 0:03:29

A simple proof of the Gaussian integral

Solving the Gaussian 0:08:52

Solving the Gaussian Integral... with polar coordinates!

Solving Gaussian Integral 0:00:25

Solving Gaussian Integral in Seconds

Gaussian Integrals 0:08:57

Gaussian Integrals

A pretty reason 0:13:16

A pretty reason why Gaussian + Gaussian = Gaussian

The Super Gaussian 0:07:45

The Super Gaussian Integral (The Art of Integration)

Does the Gaussian 0:15:52

Does the Gaussian integral trick work for other functions?

the answer to 0:00:38

the answer to the Gaussian integral! (integral of e^(-x^2) from -inf to inf)

The Gaussian Integral 0:15:49

The Gaussian Integral meets the Jacobian

Комментарии
Автор

Great video.
Thank you.
Can you please explain why dA=rdrd(theta)

باسلزمريق
Автор

Great video! The Gaussian Integral is always a fun one! Another fun video you could do is a proof on the irrationality of the square root of 2? I know it's a simple topic, but I think it's a good one. : D

georgeklimov
Автор

So what does the value of e have to do with e^-infinity^2

blueschase
Автор

But I am still getting a negative half instead of half

akanshabari