filmov
tv
Find the values where the function has horizontal tangents

Показать описание
👉 Learn how to find the point of the horizontal tangent of a curve. A tangent to a curve is a line that touches a point in the outline of the curve. When given a curve described by the function y = f(x). The value of x for which the derivative of the function y, is zero is the point of horizontal tangent for the curve.
Organized Videos:
✅The Derivative
✅Find the First and Second Derivatives of a Function
✅Find the Differentiability of a Function
✅Find the Derivative of Absolute Value Function
✅Find the Derivative of Exponential and Logarithmic Functions
✅Find the Derivative using Implicit Differentiation
✅Find the Derivative of Inverse Functions
✅Find the Point Where the Tagent Line is Horizontal
✅Write the Equation of the Tangent Line
✅Find the Derivative from a Table
✅Chain Rule Differentiation
✅Product Rule Derivatives
✅Find the Derivative of Trigonometric Functions
✅Find the Derivative using the Power Rule
✅Quotient Rule Derivatives
✅Solve Related Rates Problems
Connect with me:
#derivatives #brianmclogan
Organized Videos:
✅The Derivative
✅Find the First and Second Derivatives of a Function
✅Find the Differentiability of a Function
✅Find the Derivative of Absolute Value Function
✅Find the Derivative of Exponential and Logarithmic Functions
✅Find the Derivative using Implicit Differentiation
✅Find the Derivative of Inverse Functions
✅Find the Point Where the Tagent Line is Horizontal
✅Write the Equation of the Tangent Line
✅Find the Derivative from a Table
✅Chain Rule Differentiation
✅Product Rule Derivatives
✅Find the Derivative of Trigonometric Functions
✅Find the Derivative using the Power Rule
✅Quotient Rule Derivatives
✅Solve Related Rates Problems
Connect with me:
#derivatives #brianmclogan
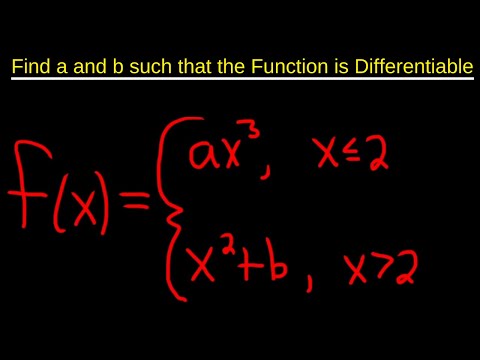
Find the values a and b that make the piecewise function continuous
How To Determine Your Core Values | 13 Questions with Dr John Demartini
How to Find Your Core Values | 3 Easy Steps
Find the values where the function has horizontal tangents
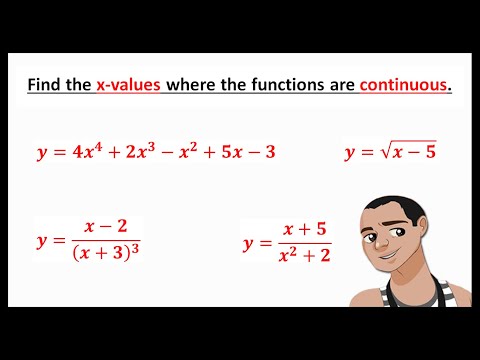
Finding the x-values where f is continous
Determine the values that make the equation true
Finding Local Maximum and Minimum Values of a Function - Relative Extrema
Find the values a and b that make the function differentiable
find the value of gamma 5/2|beta and gamma function imp question #beta_gamma
Find the values of the variables x and y given figures and congruent angles
Calculus: find x values where f is discontinuous
How to find your Core Values 🦋 | summer of self ep 4
Learn to find the values the function is undefined, then write domain
How To Identify Your Core Values: Acceptance and Commitment Therapy (ACT)
Find the Values of a and b Such That the Piecewise Function is Differentiable Everywhere (Calculus)
Learn how to find the values that are not defined for the expression
Finding Absolute Maximum and Minimum Values - Absolute Extrema
Pre-Calculus - Find the values of a and b of a complex number in standard form (3a-1)+(4b+5)i=4-7i
Psychologist's 🧠 Step-by-Step Process to Identify Your Core Values.
How to find Sports Card Values - Baseball, Football, Basketball & Hockey #sportscards #thehobby
FINDING X-VALUES FOR CONTINUOUS FUNCTIONS
How To Determine Your Core Life Values
Key Skill - Complete a table of values for a linear graph.
How to count unique values Excel
Комментарии
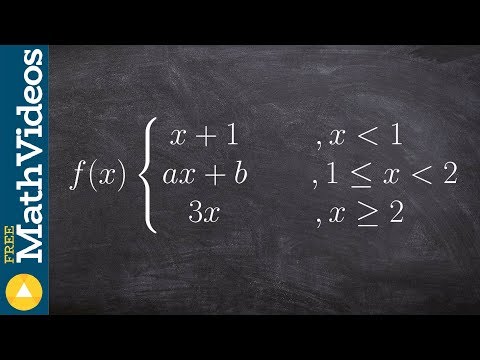 0:03:44
0:03:44
 0:12:46
0:12:46
 0:07:09
0:07:09
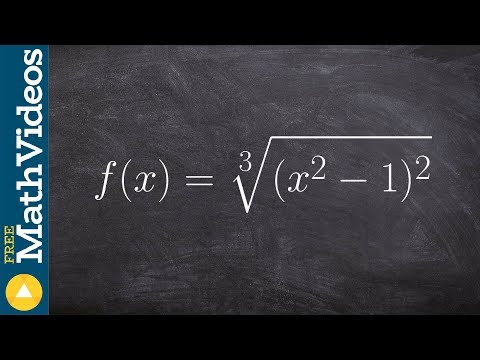 0:03:15
0:03:15
 0:04:48
0:04:48
 0:02:08
0:02:08
 0:14:18
0:14:18
 0:06:05
0:06:05
 0:04:32
0:04:32
 0:08:24
0:08:24
 0:02:36
0:02:36
 0:16:01
0:16:01
 0:01:07
0:01:07
 0:07:31
0:07:31
 0:04:01
0:04:01
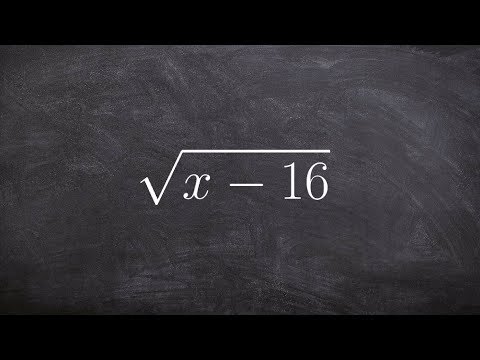 0:02:36
0:02:36
 0:17:17
0:17:17
 0:03:12
0:03:12
 0:09:19
0:09:19
 0:10:26
0:10:26
 0:02:45
0:02:45
 0:38:02
0:38:02
 0:02:34
0:02:34
 0:00:26
0:00:26