filmov
tv
Squeeze Theorem For Sequences
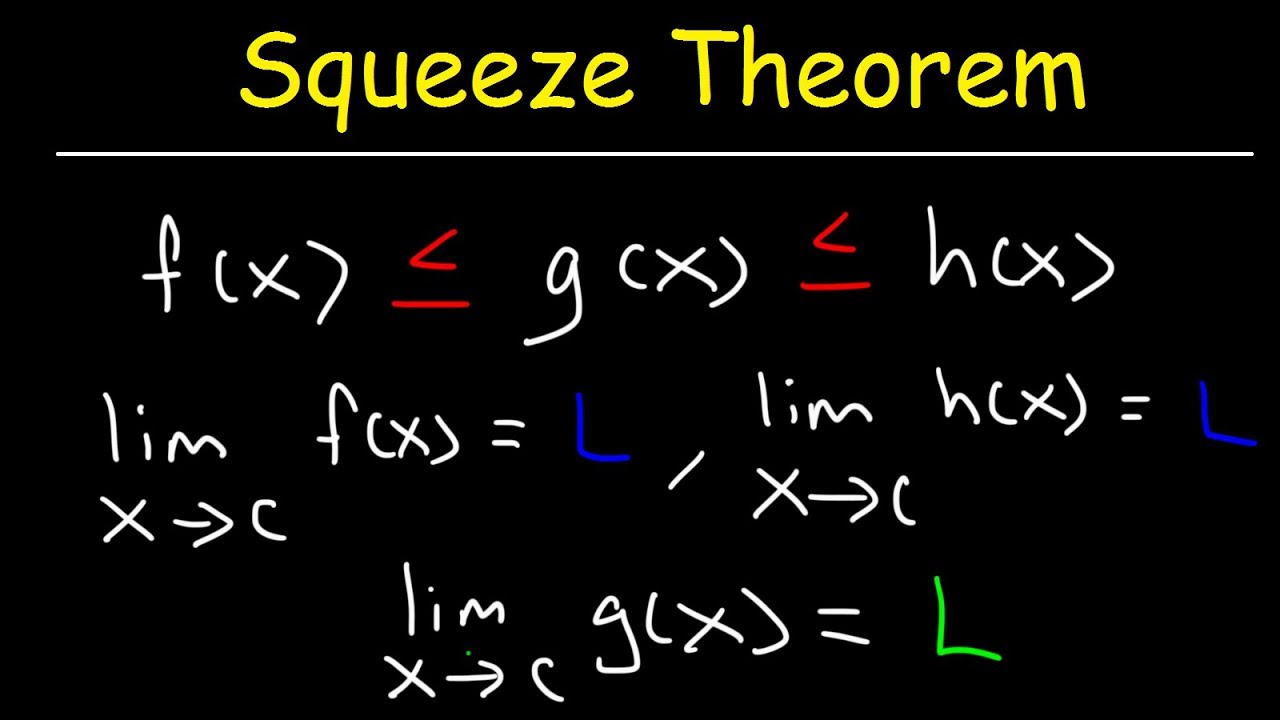
Показать описание
This calculus 2 video tutorial explains how to determine the convergence and divergence of a sequence using the squeeze theorem.
Converging & Diverging Sequences:
Monotonic & Bounded Sequences:
Absolute Value Theorem - Sequences:
Squeeze Theorem - Sequences:
________________________________
Geometric Series & Sequences:
Introduction to Series - Convergence:
Divergence Test For Series:
Harmonic Series:
Telescoping Series:
__________________________________
Integral Test For Divergence:
Remainder Estimate - Integral Test:
P-Series:
Direct Comparison Test:
Limit Comparison Test:
___________________________________
Calculus Final Exam and Video Playlists:
Full-Length Videos and Worksheets:
Converging & Diverging Sequences:
Monotonic & Bounded Sequences:
Absolute Value Theorem - Sequences:
Squeeze Theorem - Sequences:
________________________________
Geometric Series & Sequences:
Introduction to Series - Convergence:
Divergence Test For Series:
Harmonic Series:
Telescoping Series:
__________________________________
Integral Test For Divergence:
Remainder Estimate - Integral Test:
P-Series:
Direct Comparison Test:
Limit Comparison Test:
___________________________________
Calculus Final Exam and Video Playlists:
Full-Length Videos and Worksheets:
0:06:02
Squeeze Theorem For Sequences
0:05:17
Applying the Squeeze Theorem for Sequences
0:08:10
Proof: Sequence Squeeze Theorem | Real Analysis
0:10:49
SQUEEZE THEOREM - The Setup
0:07:50
How to Prove the Squeeze Theorem for Sequences
0:10:43
Squeeze Theorem
0:08:03
Proof of the Squeeze Theorem for Sequences
0:13:19
Squeeze Theorem Examples with Sequences [Real Analysis]
0:07:11
Squeeze theorem or sandwich theorem | Limits | Differential Calculus | Khan Academy
0:10:55
Limit of n!/n^n as n goes to infinity, squeeze theorem, calculus 2 tutorial
0:10:54
Sequences: Using the Squeeze Theorem on (-1)^n
2:27:29
Calculus 2 Lecture 9.1: Convergence and Divergence of Sequences
0:05:21
9_1_d Squeeze Theorem for Sequences
0:05:34
(Squeeze Thrm) Limit n goes to Infinity 7^n /n!
0:10:11
Sequences and the Squeeze Theorem
0:07:58
Convergence and Divergence of a sequence - The squeeze theorem and another short theorem
0:09:16
Limits of a Sequence: The Squeeze Theorem
0:07:10
Sandwich theorem | squeeze theorem | proof | Real analysis | sequence and series | Real sequence
0:10:03
1.2.4 - The Squeeze Theorem For Sequences
0:20:28
Introduction and Proof of Squeeze Theorem for Sequences [Real Analysis]
0:19:41
Squeeze Principle or Sandwich Theorem for Sequences | Complete Proof & Question | Real Analysis
0:31:23
Monotonic Sequences and Bounded Sequences - Calculus 2
0:08:17
Sandwich Theorem (Calculus) for Convergence and Divergence
0:06:15
Quiz 6 Problem 1c Sequence Convergence Using the Squeeze Theorem
Комментарии