filmov
tv
Parametric Equations for a Ladder Sliding Down a Wall (Multivariable Calculus Challenge Problems)
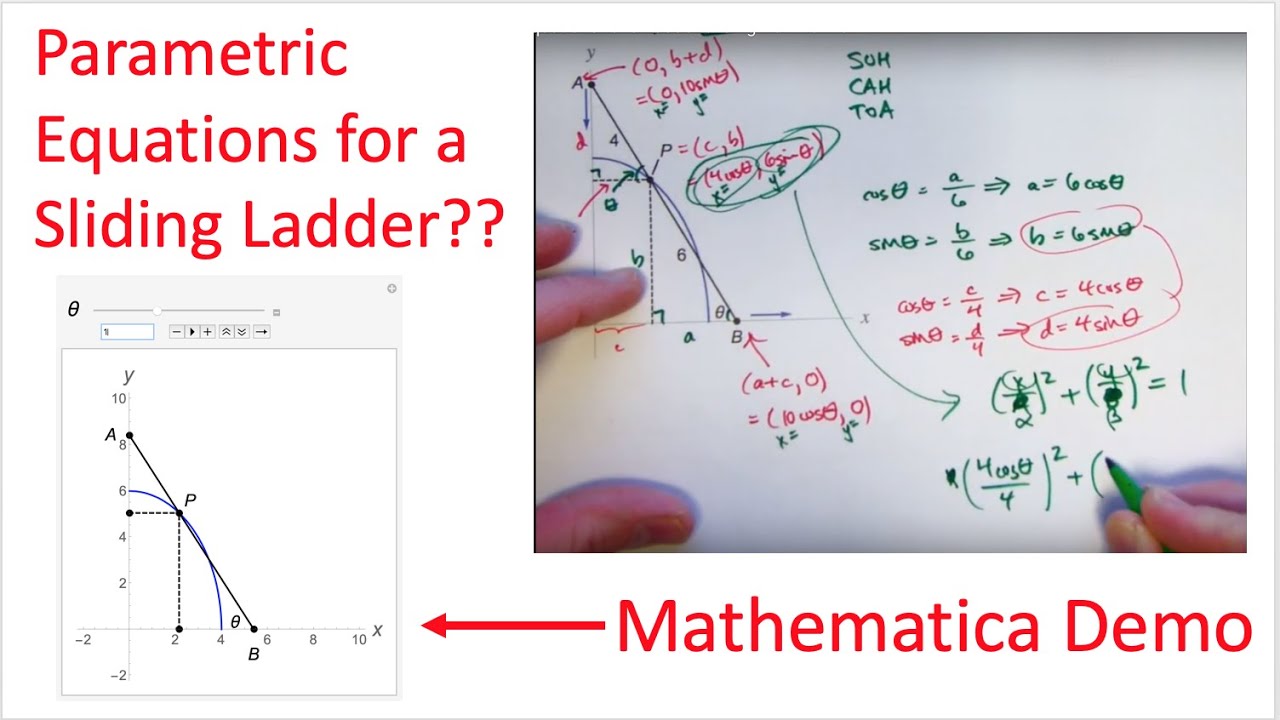
Показать описание
This is similar to Oxford's ladder interview question, which is the topic of a video on the channel Mindyourdecisions related to calculus.
Multivariable Calculus Challenge Problems (with Mathematica Demonstrations), Video #1. Exercise #11.1.66 from the 3rd Edition of "Multivariable Calculus", by Jon Rogawski and Colin Adams.
(0:00) The first of many videos on Challenge Problems from Multivariable Calculus. I will also use Mathematica demonstrations in these videos as well. (2:01) Problem statement. (3:41) Mathematica demonstration (an animation with the Mathematica Manipulate function). (6:22) Solve the challenge problem using the diagram and trigonometry with a right triangle (use SOH CAH TOA for the sine, cosine, and tangent of the angle). (13:04) Show the point P follows the path along an ellipse by using the equation of an ellipse (substitute the parametric equations into it) and the Pythagorean identity (cos^2 + sin^2 = 1 for all input angles). (16:16) Concluding comments.
Problem Statement: Rogawski & Adams, Third Edition, #11.1.66: A 10-ft ladder slides down a wall as its bottom B is pulled away from the wall (see figure below). Using the angle theta as a parameter, find the parametric equations for the path followed by (a) the top of the ladder A, (b) the bottom of the ladder B, and (c) the point P located 4 ft from the top of the ladder. Show that P describes (part of) an ellipse.
AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
Комментарии
 0:17:19
0:17:19
 0:06:14
0:06:14
![[Calculus] Parametric Equations](https://i.ytimg.com/vi/6uKj4Gu-6TU/hqdefault.jpg) 0:12:04
0:12:04
 0:00:54
0:00:54
 0:06:34
0:06:34
 0:11:51
0:11:51
 0:12:57
0:12:57
 0:01:19
0:01:19
 0:05:43
0:05:43
 0:17:10
0:17:10
 0:05:08
0:05:08
 0:00:53
0:00:53
 0:03:09
0:03:09
 0:06:40
0:06:40
 0:06:12
0:06:12
 0:03:23
0:03:23
 0:04:30
0:04:30
 0:02:14
0:02:14
 0:05:45
0:05:45
 0:26:03
0:26:03
 0:29:43
0:29:43
 0:06:49
0:06:49
 0:31:36
0:31:36
 0:06:06
0:06:06