Transpose of a Matrix | Symmetric & Skew-Symmetric Matrices Explained | Engineering Essentials

Explore the concepts of Transpose of a Matrix, Symmetric Matrix, and Skew-Symmetric Matrix in this comprehensive video from Engineering Essentials. Learn the mathematical process behind transposing a matrix and how to identify symmetric and skew-symmetric matrices, with clear examples and step-by-step explanations. This video is perfect for engineering, mathematics, and statistics students studying at universities like MIT, Stanford, IITs, and more. It also caters to Class 11 & 12 students (CBSE/NCERT/AP/IB) and aspirants of entrance exams like IIT JEE and SAT.

A symmetric matrix is a square matrix that remains unchanged when transposed, while a skew-symmetric matrix changes the sign of its elements. Both types are crucial in linear algebra and have applications in areas like quantum mechanics and engineering mathematics.

#TransposeOfMatrix #SymmetricMatrix #SkewSymmetricMatrix #MatrixMultiplication #MultiplyingMatrices #LinearAlgebra #MatrixAddition #Matrices #LinearAlgebra #MathForEngineers #EngineeringMathematics #IITJEE #SATPrep #Class12Math #CBSE #NCERT #UniversityMath #MIT #Stanford #IITs #CBSE #AP
#MatrixIntroduction #TypesOfMatrices #MatrixEquality #EngineeringMathematics #UniversityMath #CBSE #NCERT #MatrixOperations #Determinants #EngineeringMathematics #SAT #Class12 #UniversityMathematics

Transpose of a Matrix | Symmetric matrix & Skew symmetric matrix #maths #eranand
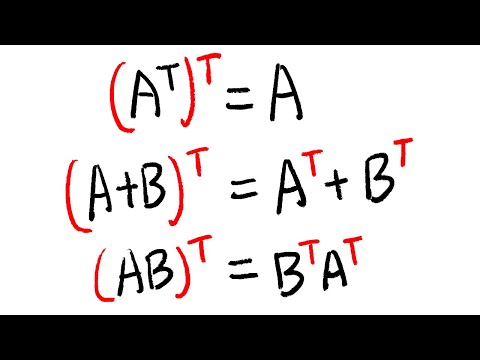
In linear algebra, the transpose of a matrix is an operator which flips a matrix over its diagonal; that is, it switches the row and column indices of the matrix A by producing another matrix, often denoted by AT. The transpose of a matrix was introduced in 1858 by the British mathematician Arthur Cayley.
The main use of matrices is to represent linear maps between finite-dimensional vector spaces. the transpose is an operation on matrices that may be seen as the representation of some operation on linear maps. This leads to a much more general definition of the transpose that works on every linear map, even when linear maps cannot be represented by matrices (such as in the case of infinite dimensional vector spaces). In the finite dimensional case, the matrix representing the transpose of a linear map is the transpose of the matrix representing the linear map, independently of the basis choice.
Symmetric matrix & Skew symmetric matrix

Symmetric Matrices
A symmetric matrix is a square matrix that is equal to its own transpose. In other words, the elements of a symmetric matrix are the same above and below the main diagonal.
Symmetric matrices have many important properties. For example, they are always invertible, and their eigenvalues are all real. Symmetric matrices are also used in a variety of applications, such as linear algebra, differential equations, and physics.
Skew Symmetric Matrices
A skew symmetric matrix is a square matrix whose transpose is equal to its negative. In other words, the elements of a skew symmetric matrix are the opposite of the elements above and below the main diagonal.
Skew symmetric matrices also have many important properties. For example, they are always invertible, and their eigenvalues are all pure imaginary. Skew symmetric matrices are also used in a variety of applications, such as linear algebra, differential equations, and physics.
Join My WhatsApp Channel to get regular updates:
(Your number is not visible to anyone including the Admin)