filmov
tv
📚 Use matrices to balance a chemical equation

Показать описание
Follow us:
Write a balanced chemical equation for the following reaction (propane combustion):
C_3 H_8+O_2→CO_2+H_2 O
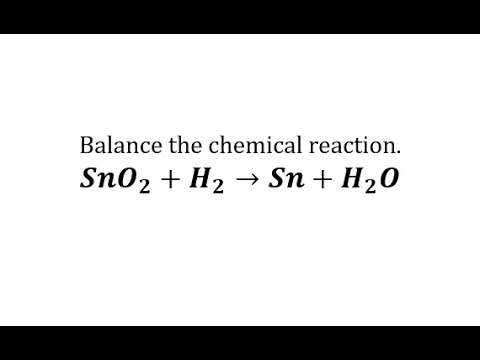
Balancing Chemical Equations: Solving a system of equations using matrices
Example: HClO4 + P4O10 → H3PO4 + Cl2O7
1) We represent each coefficient with a variable (e.g. a-d)
aHClO4 + bP4O10 → cH3PO4 + dCl2O7
2) Write equations to represent the relationship between each element in each species
H: a=3c Cl: a=2d O: 4a + 10b = 4c + 7d P: 4b = c
3) Insert the coefficients from the above equations into an augmented matrix. The vertical line represents an ‘equal sign’. Numbers that are on the wrong side of the ‘equal sign’ must have their sign changed (become negative). Computer programs commonly use matrices to solve equations.
4) Since we are solving for a relationship (the ratio of the coefficients) not specific numbers, we need one less equation than the number of unknowns (4 unknowns, we need 3 equations). The last variable (d) will be set to 1. We can therefore drop the most complex equation.
5) We are attempting to have one 1 in each row and column on the left side, with all the other numbers being 0. The values on the right side correspond to the values of our coefficients.
6) There are 3 valid row operations that we can perform with matrices:
a. Switch two rows
b. Add or subtract two rows from each other
c. Multiply or divide a row by a number
7) We can combine the above operations in some cases (e.g. we can subtract a multiple of a row from another row).
8) Row operations are described above the transition arrows, with the subscript represent the row number (the top row is #1)
9) The value of the coefficients (a, b, and c) can be read from the final matrix:
a. 2 b. 1/6 c. 2/3 d. 1 (implied)
10) We multiply each coefficient by 6 to remove the fractions and sub the numbers into our original equation. Our final, balanced equation is:
12HClO4 + P4O10 → 4H3PO4 + 6Cl2O7
Комментарии
 0:08:07
0:08:07
 0:07:22
0:07:22
 0:07:21
0:07:21
 0:17:40
0:17:40
 0:06:46
0:06:46
 0:09:27
0:09:27
 0:14:57
0:14:57
 0:09:03
0:09:03
 0:10:34
0:10:34
 0:26:07
0:26:07
 0:12:32
0:12:32
 0:12:26
0:12:26
 0:08:26
0:08:26
 0:09:55
0:09:55
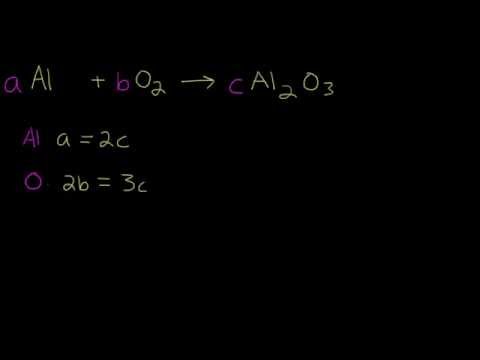 0:03:56
0:03:56
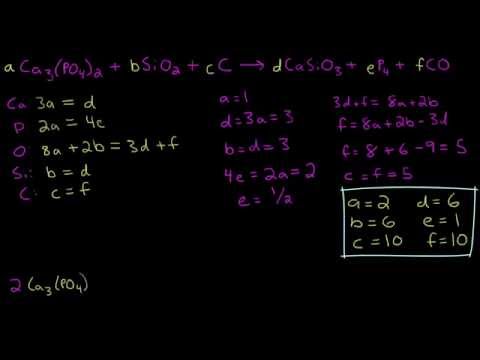 0:08:12
0:08:12
 0:09:23
0:09:23
 0:04:09
0:04:09
 0:06:33
0:06:33
 0:03:25
0:03:25
 0:57:59
0:57:59
 0:00:13
0:00:13
 0:00:37
0:00:37
 0:07:12
0:07:12