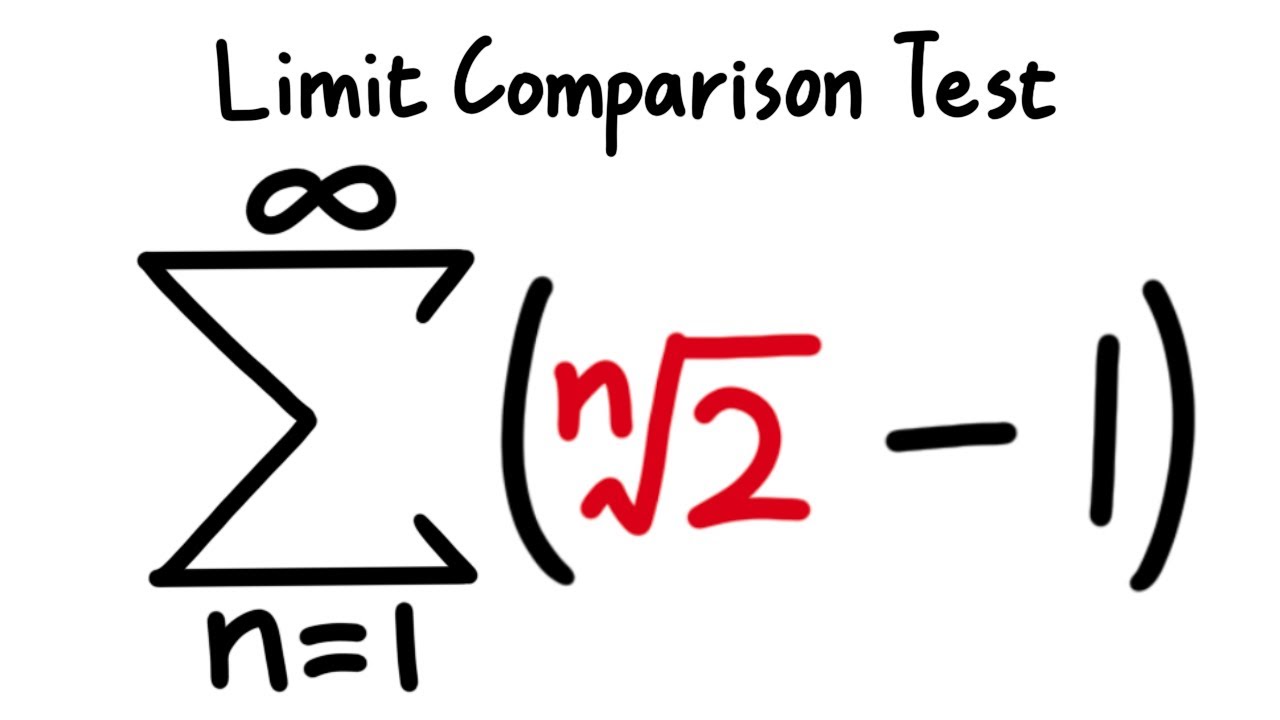
Series of 2^(1/n)-1 with limit comparison test, calculus 2 tutorial

Показать описание

Does this Series Converge or Diverge? Series (2^(1/n)-1)

----------------------------------------
#blackpenredpen #math #calculus #apcalculus

Рекомендации по теме

Комментарии

I was trying to figure out a nice way to explain this problem to my calculus II class, since I don't like the idea of just guessing the right comparison. It turns out that you can gain intuition for why you should compare to the harmonic series by first using the power series expansion for e^x. Using this, we have that 2^(1/n) = e^(1/n ln(2)) ≈ 1 + 1/n ln(2). Therefore 2^(1/n) - 1 ≈ 1/n ln(2) for large n. We could also use the linearization of 2^x at x=1 to get an idea of how 2^(1/n) behaves.

dirkdugan

If the series contains a composite function (e.g. 2^(1/x) as in this video), is one of its inner functions (1/x here) always a valid argument for the comparison test?

josephlauletta

u can just use integral test. f(x)=2^(1/x) - 1 is monotone decreasing for x>1, and integral 1 to infinity of 1 diverges, so the series diverges. lmk if this is flawed but that's the first way to do it that i saw

YorangeJuice

OMG He has a beard and doesn't use pens
I'm shaken ;)

_franci

we do not know lopital yet so we cannot use it

aashsyed

Series of 2^(1/n)-1 with limit comparison test, calculus 2 tutorial

Determine if sequence converges or diverges, if converges find limit (-1)^n/(2sqrt(2))

lim n^1/n = 1

Proof: Sequence (n+1)/n Converges to 1 | Real Analysis

Series of 2^(1/n)-1 with limit comparison test, calculus 2 tutorial

Limit of (-1)^n(n/(n + 1))

Find the Limit of SUM((1/n^3)(i - 1)^2) as n approaches infinity

Series of (1+1/n)^(n^2), root test

Series of sin(1/n) diverges, Limit comparison test, calculus 2 tutorial

Chain Rule Shortcut Method of Differentiation | Derivatives #excellenceacademy #jonahemmanuel

Determine if sequence converges or diverges, if converges find limit {(1+ 2/n)^n}

Determining whether a sequence converges or diverges

❖ Finding the Limit of a Sequence, 3 more examples ❖

Evaluate: Lim(n→∞)[1/(n+1)+1/(n+2)+......+1/2n]

Proof: Sequence (3n+1)/(n+2) Converges to 3 | Real Analysis

Limit Comparison Test, Series of 1/(2n+1), calculus 2 tutorial

Sequences (Real Analysis) | SE#12-13 | Monotone convergence theorem | 1/n+1...+1/n+n & 1/1!+...+...

Converging and Diverging Sequences Using Limits - Practice Problems

Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test

Determine if sequence converges or diverges, if converges find limit ((-1)^(n+1) n)/(n + sqrt(n))

checking for absolute convergence, series of sin(2n)/(1+2^n), calculus 2 tutorial

How to Determine if a Sequence Converges or Diverges: Example with n*sin(1/n)

Telescoping Series

Calculus 2 Lecture 9.1: Convergence and Divergence of Sequences

Proof that the Sequence (-1)^n Diverges using the Definition