filmov
tv
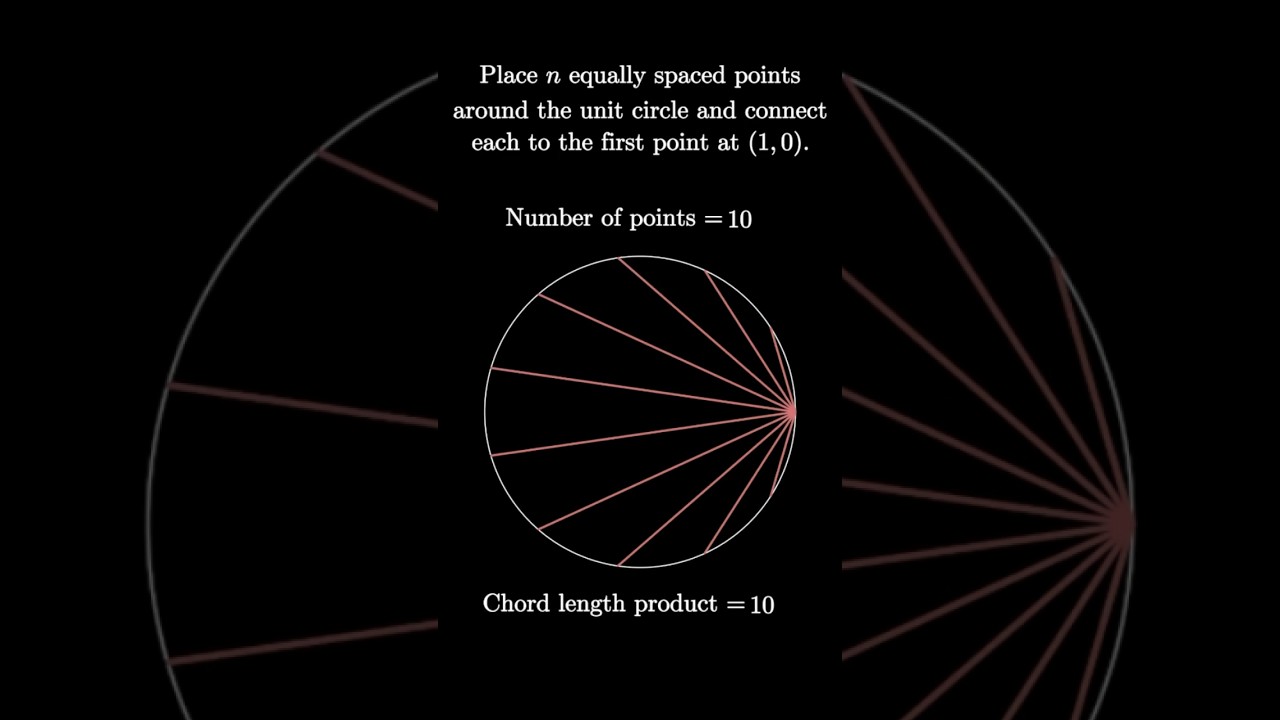
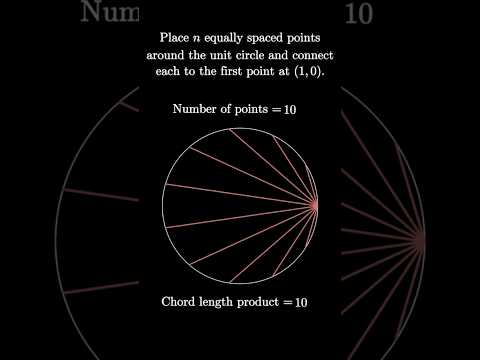
Product of chords?
Показать описание
This short animation shows chords connecting n equally space points to one of those points on a unit circle and computes the product of the chord lengths. Do you have a conjecture based on this? Can you prove it?
#math #mathvideo #manim #circle #chords #visualproof #trigonometry #sine #cosine #tangent #rootsofunity #complexanalysis #complexnumbers #products #geometry
#math #mathvideo #manim #circle #chords #visualproof #trigonometry #sine #cosine #tangent #rootsofunity #complexanalysis #complexnumbers #products #geometry
Products of Chords in a Circle (math visualization)
Product of chords?
Intersecting Chords Theorem (visual proof)
Product of Chords
What is the relationship of the product of two chords that intersect inside of a circle
Circle Theorems: Intersecting Chords - IGCSE Maths - Addvance Maths 😊➕
Algebra 2, Lesson 89.C - Products of Chords and Secants
Circles: Measuring Chords
The Perfect Beginner Guitar? | Fesley FST100
Secret Deep House Chords 🤯
Product of segment lengths of two chords on a circle
How To Play EASY Jazz Chords? | Chord Progressions For Beginners #easypianobeginners #jazzmusic
☝️ Get the chords cheat sheet in my bio ☝️
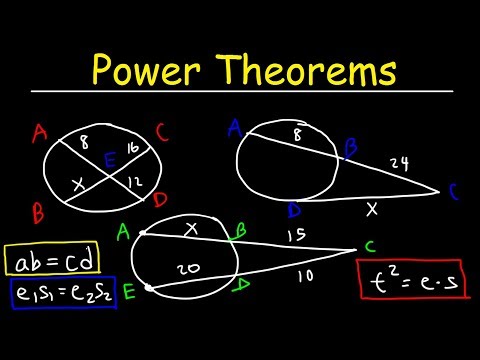
Power Theorems - Chords, Secants & Tangents - Circle Theorems - Geometry
Gospel JAZZ Chords For Beginners | Piano Tutorial
Important Chords For Black Metal #blackmetal #guitar #riff
Proof -- product of segments of two intersecting chords equal
Chord Chord Power Theorem | Intersecting Chords Theorem | Circles | Geometry
Intercept Properties - Circle Geometry (1 of 3: Intersecting chords)
The 3 Secret Guitar Chords (SOUNDS AMAZING!!) #shorts #guitarlesson
intersecting chords of circles (KristaKingMath)
FASTEST way to play ADVANCED chords | The 7 Pro Chords | Advanced Gospel Chords for Beginners
FL Studio HIDDEN GEM For Perfect Chords
Intersecting Chords in a Circle and Their Lengths
Комментарии
 0:06:21
0:06:21
 0:00:29
0:00:29
 0:02:30
0:02:30
 0:21:35
0:21:35
 0:02:05
0:02:05
 0:05:21
0:05:21
 0:07:36
0:07:36
 0:02:56
0:02:56
 0:14:02
0:14:02
 0:00:52
0:00:52
 0:03:32
0:03:32
 0:00:09
0:00:09
 0:00:52
0:00:52
 0:23:01
0:23:01
 0:00:31
0:00:31
 0:00:19
0:00:19
 0:02:19
0:02:19
 0:03:11
0:03:11
 0:05:59
0:05:59
 0:01:00
0:01:00
 0:04:01
0:04:01
 0:00:42
0:00:42
 0:00:33
0:00:33
 0:06:11
0:06:11