filmov
tv
Introduction to Bayesian statistics, part 1: The basic concepts

Показать описание
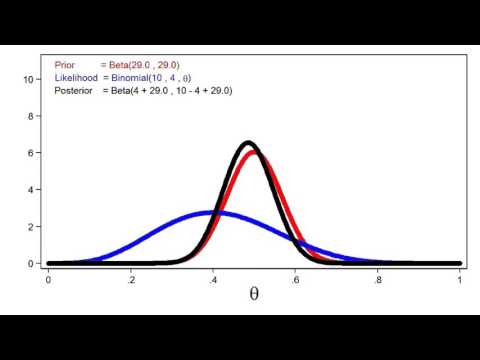
An introduction to the concepts of Bayesian analysis using Stata 14. We use a coin toss experiment to demonstrate the idea of prior probability, likelihood functions, posterior probabilities, posterior means and probabilities and credible intervals.
Copyright 2011-2019 StataCorp LLC. All rights reserved.
Copyright 2011-2019 StataCorp LLC. All rights reserved.
Introduction to Bayesian statistics, part 1: The basic concepts
Introduction to Bayesian statistics, part 2: MCMC and the Metropolis–Hastings algorithm
An introduction to Bayesian statistics
Introduction to Bayesian Statistics - A Beginner's Guide
Introduction to Bayesian data analysis - part 1: What is Bayes?
You Know I'm All About that Bayes: Crash Course Statistics #24
Probability, Part 4: Super Simple Explanation of Bayesian Statistics for Dummies
Bayesian Statistics: An Introduction
Class 5: Introduction to MCMC and Stan (Lecture 3a, Part 1; Bayesian Psychometric Models F2024)
A (brief) introduction to Bayesian statistics
Bayes theorem, the geometry of changing beliefs
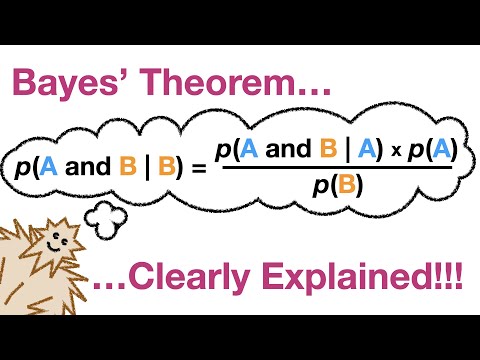
Bayes' Theorem, Clearly Explained!!!!
A Short Introduction to Bayesian Statistics
The better way to do statistics
Are you Bayesian or Frequentist?
Tutorial 10: Bayesian Inference: Part 1
An Introduction to Bayesian Analysis 2016
Introduction to Bayesian Statistical Methods Part 1 8 minutes
Introduction to Bayesian data analysis - part 3: How to do Bayes?
Bayesian Statistics - Introduction to Bayesian inference
R-Ladies Amsterdam: Intro to Bayesian Statistics in R by Angelika Stefan
Introduction to Bayesian data analysis - Part 2: Why use Bayes?
Bayesian Statistics Intro
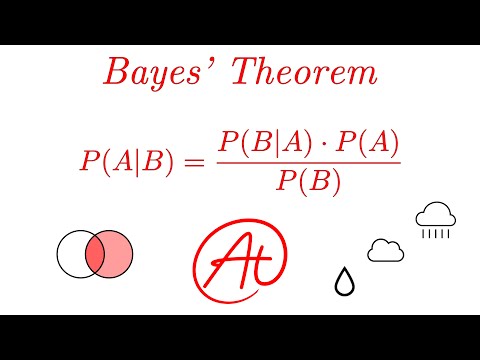
Bayes' Theorem EXPLAINED with Examples
Комментарии
 0:09:12
0:09:12
 0:08:14
0:08:14
 0:09:05
0:09:05
 1:18:47
1:18:47
 0:29:30
0:29:30
 0:12:05
0:12:05
 0:05:08
0:05:08
 0:38:19
0:38:19
 0:59:58
0:59:58
 0:25:46
0:25:46
 0:15:11
0:15:11
 0:14:00
0:14:00
 0:12:34
0:12:34
 0:17:25
0:17:25
 0:07:03
0:07:03
 0:10:52
0:10:52
 1:04:31
1:04:31
 0:09:42
0:09:42
 0:37:52
0:37:52
 0:09:13
0:09:13
 1:48:40
1:48:40
 0:23:00
0:23:00
 0:31:39
0:31:39
 0:08:03
0:08:03