filmov
tv
Calculus In The REAL World!!
Показать описание
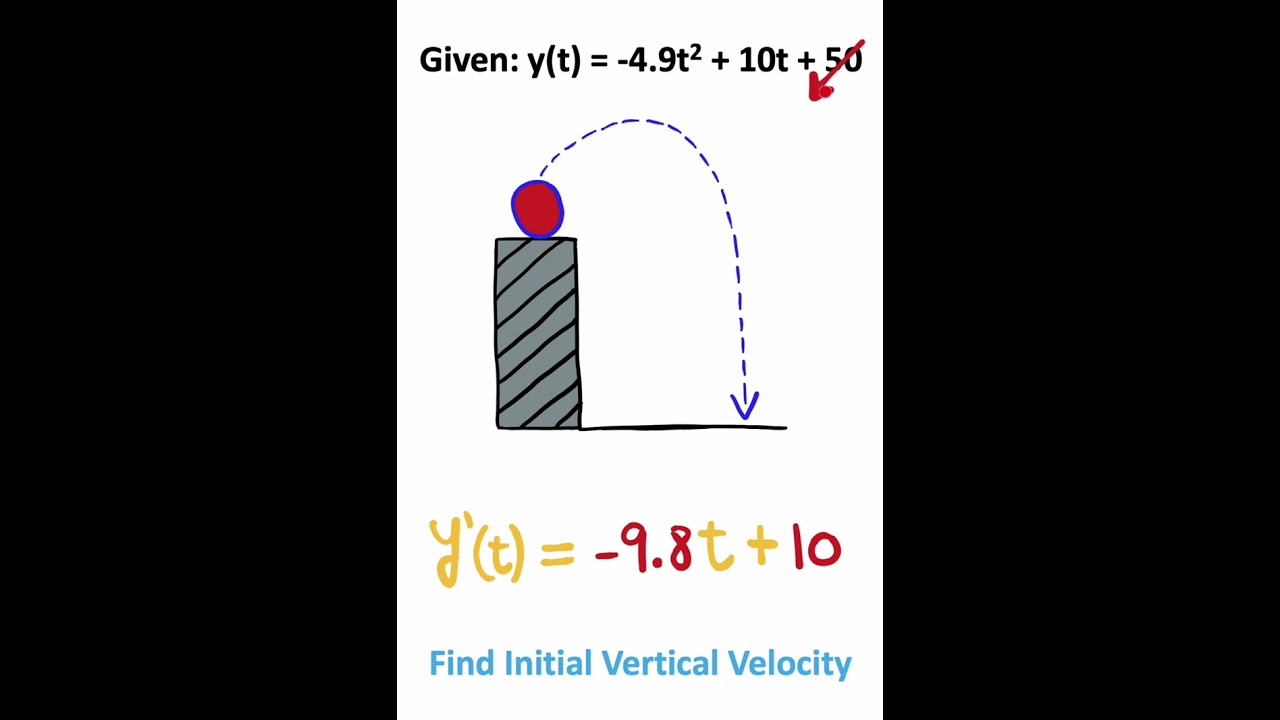
How To Solve Projectile Motion Problems Using Calculus!! #Math #Science #Velocity #College #NicholasGKK #Shorts
What is Calculus used for? | How to use calculus in real life
Unlock the Power of Math with Real-World Calculus and Statistics
Calculus in the Real World
HOW TO USE CALCULUS IN THE REAL WORLD?
How to Make it Through Calculus (Neil deGrasse Tyson)
Calculus in a nutshell
Real World Calculus: How Maths Drives Formula One
REAL LIFE APPLICATIONS OF CALCULUS WITH EXAMPLES | MATHS IN REAL LIFE | MATHS REAL WORLD PROBLEMS
Trinidad and Tobago Math Olympiad - Exponential Equations - intermediate difficulty
What is Integration? 3 Ways to Interpret Integrals
This Is the Calculus They Won't Teach You
Dear all calculus students, This is why you're learning about optimization
What Are Derivatives and How Do They Work? Calculus in Context!
The essence of calculus
Calculus In The REAL World!!
Calculus, what is it good for?
Gilbert Strang: Linear Algebra vs Calculus
This is why you're learning differential equations
Math Integration Timelapse | Real-life Application of Calculus #math #maths #justicethetutor
Mathematics in real life | MrBMaths.com
The Map of Mathematics
Real Life Application of Derivatives
Do we need calculus in real world?
How I Used Calculus to Beat My Kids at Mario Kart
Комментарии
 0:11:39
0:11:39
 0:07:44
0:07:44
 0:08:05
0:08:05
 0:07:07
0:07:07
 0:03:38
0:03:38
 0:03:01
0:03:01
 0:01:51
0:01:51
 0:07:48
0:07:48
 0:03:15
0:03:15
 0:10:55
0:10:55
 0:30:17
0:30:17
 0:16:34
0:16:34
 0:11:24
0:11:24
 0:17:05
0:17:05
 0:01:00
0:01:00
 0:07:43
0:07:43
 0:02:14
0:02:14
 0:18:36
0:18:36
 0:00:09
0:00:09
 0:05:33
0:05:33
 0:11:06
0:11:06
 0:03:51
0:03:51
 0:06:48
0:06:48
 0:08:04
0:08:04