Max Area Enclosed by Rectangular Fence - Optimization Problem #4

🌾 Maximizing A Rectangular Fence Area 🌾

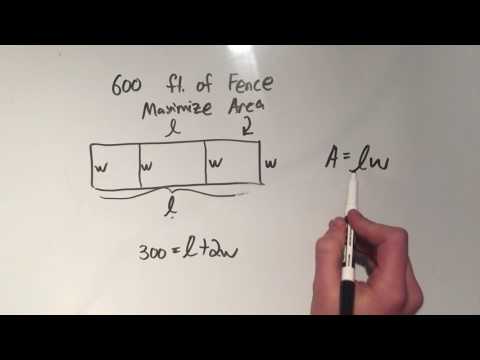
In this video, we tackle Optimization Problem #4, guiding a farmer on how to find the largest area possible for a rectangular pen using 500 feet of fencing material. Although this problem can be approached with algebra, we’ll focus on a calculus-based method to highlight the optimization journey.

What You’ll Discover:

Problem Setup: Learn how to establish the necessary equations to maximize the area effectively.
Calculus Application: Watch as we differentiate the area function, identify critical points, and calculate the maximum area achievable.
Step-by-Step Guidance: Understand the complexities of formulating optimization problems and how to navigate through them using derivatives.
Why Tune In?

Perfect for Students: A great resource for high school and college students diving into calculus and optimization concepts.
Simplified Explanations: Enjoy clear, concise instructions that break down challenging ideas into manageable steps.
Real-World Relevance: See how optimization principles apply in farming and land use scenarios.
📈 Remember to:

LIKE this video if it enhances your understanding!
SHARE with peers eager to excel in optimization problems!
SUBSCRIBE for more insightful math tutorials, problem-solving techniques, and educational resources!

#Optimization #Calculus #MaximizingArea #RectangularFence #Mathematics #MathTutorial #EducationalContent #LearningCalculus #ProblemSolving #HighSchoolMath #CollegeCalculus #RealWorldApplications #MaximizationTechniques

[patrickjmt]

Hi all! Wanna help a Youtube education OG? Please post comments, questions and anything else on your mind in the comment section! so, don’t forget to LIKE, THUMBS UP, and SUBSCRIBE! I’d appreciate it greatly as it helps me :)

[hhharib]

My typical after school schedule: 1st period Chemistry with Tyler Dewitt and 2nd period Calc with patrickJMT

[nidhishgautam]

Thanks dude. I'm going to do that with my cows.

[rosethanitmongkolsak]

that moment when a 10 min video made more sense than a 2 hour class teaching about the same thing...

[RogerKlauser]

Calc final on Thursday. You and your vids just might help preserve my grade. Thanks Patrick!!

[scotlandw]

It's amazing to think people came up with these formulas. Thanks for the video.

[dolphinsatsunset]

I labeled my rectangle differently. The side parallel to the river I labeled x and the other two sides I labeled y. So my equation was x + 2y = 500 and thus y = (500 - x)/2. Then A = x [ (500 - x)/2 ] which gives A = 250x - 1/2 * x^2 and the derivative is dA/dx= 250 - x. When I set that equal to 0, I get x = 250. Then I plug that back in my original formula and I receive 125 for my y value. A = x * y = (125)(250) = 31, 250 square feet. So my computation was different but I still received the same answer!!!

[Minecraft]

My guy, a girl sent me a problem like this and asked for my help. I haven’t done math since like 4th grade and I understood this perfectly. Thank u my guy

[chrisre]

This was a fantastic video. I've been stuck on this kind of problem for 45 minutes now. Your video has really cleared things up for me. Thank you!

[monkeydemon]

When I was in calculus I spent 10 weeks (the length of the quarter) trying to get this down.... and my professor barely helped me at all. You just made it ridiculously clear in under 10 min.

[nathanielrosillo]

I got this exact problem on my online exam but with 800ft lmao good looks bro thanks for the help 😂

[teargun]

Will do man..there's many foreign students here who do not understand a thing the lecturers are babbling about in class, cause everything's in Chinese...your videos are life savers for some of us...cheers....

[bethwilliams]

You're better than my calculus teacher! And my calculus teacher is pretty great...

[amy_muk]

Thank you so much! You really broke down the process and concept of optimization. Everything made so much more sense!

[RhemaBassey]

Dude your videos really helps me, and are crystal clear, thanks a bunch !

[stefanodomeni]

I ended up doing it by constraining the perimeter. I said well if we only have 500ft of material to work with. The the perimeter is 2x+y. So, 500 = 2x+y.

From there I got y=250-x and I plugged that into the area, a=x(250-x).

I was scared I got it wrong when I saw you did it a different way but I also arrived at x=125.

[juliaphillips]

patrick!! u da man UR AWSEOME- love from austria !!!

[isaiaspetros]

Very smart guy. I have never seen such a great Math teacher. May God Bless you. Thank you thank you so much.

[vejayjoshobusan]

in this case, u can just divide the fencing material to 2 then that will be your length. the remaining fencing material will be divided by 2 then that will be your width. now that you have your length and width, multiply it then that'll be the maximum area.

[teargun]

wish you were my math teacher..man I would pay to attend your classes..its a pity I am in China...your videos are so simple to understand..thanks for the free videos man...peace!!!...