filmov
tv
Year 12/AS Pure Chapter 4.3 (Graphs and Transformations)

Показать описание
This video builds on the skills practiced last lesson where we sketched quartics. We now take a look at how we can apply similar ideas to sketch reciprocal functions, as well as finding the points of intersection between functions and coordinate axes.
Introduction: 00:00
Solution to Warm-Up Q1: 0:40
Solution to Warm-Up Q2: 4:07
Solution to Warm-Up Q3a): 6:13
Solution to Warm-Up Q3b): 7:29
Solution to Warm-Up Q4a): 8:44
Solution to Warm-Up Q4b): 9:51
Introduction to reciprocal graphs: 11:15
Student activity: matching reciprocal graphs/equations: 13:55
Points of Intersection: 18:37
Example 1 Method 1: 19:06
Example 1 Method 2: 22:09
Example 2: 24:57
Student Activity 1- sketch 𝑦 + 1 = 0: 29:32
Student Activity 1- sketch 𝑦 = 𝑥² − 𝑥: 31:06
Student Activity 1 - sketch 𝑦 + ¹/𝑥 = 0: 31:56
Student Activity 1 - sketch 𝑦 = ¹/𝑥²: 33:43
Student Activity 1 - sketch 𝑦 = 𝑥(𝑥 − 1)²: 34:17
Student Activity 1 - sketch 𝑦 = 𝑥²(𝑥 − 1)²: 34:17
Student Activity 2 - match functions by no. of solutions: 35:56
Student Activity 3: 51:12
Suggested Exercises: 57:18
Introduction: 00:00
Solution to Warm-Up Q1: 0:40
Solution to Warm-Up Q2: 4:07
Solution to Warm-Up Q3a): 6:13
Solution to Warm-Up Q3b): 7:29
Solution to Warm-Up Q4a): 8:44
Solution to Warm-Up Q4b): 9:51
Introduction to reciprocal graphs: 11:15
Student activity: matching reciprocal graphs/equations: 13:55
Points of Intersection: 18:37
Example 1 Method 1: 19:06
Example 1 Method 2: 22:09
Example 2: 24:57
Student Activity 1- sketch 𝑦 + 1 = 0: 29:32
Student Activity 1- sketch 𝑦 = 𝑥² − 𝑥: 31:06
Student Activity 1 - sketch 𝑦 + ¹/𝑥 = 0: 31:56
Student Activity 1 - sketch 𝑦 = ¹/𝑥²: 33:43
Student Activity 1 - sketch 𝑦 = 𝑥(𝑥 − 1)²: 34:17
Student Activity 1 - sketch 𝑦 = 𝑥²(𝑥 − 1)²: 34:17
Student Activity 2 - match functions by no. of solutions: 35:56
Student Activity 3: 51:12
Suggested Exercises: 57:18
Year 12/AS Pure Chapter 4.3 (Graphs and Transformations)
All of Graphs and Transformations in 30 Minutes! | Chapter 4 | A-Level Pure Maths Revision
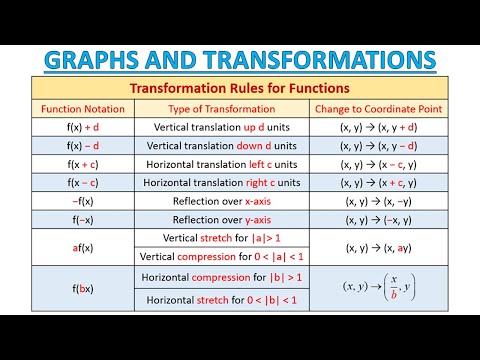
Year 12/AS Pure Chapter 4.1 (Graphs and Transformations)
Year 12/AS Pure Chapter 3.4 (Equations and Inequalities)
Memorization Trick for Graphing Functions Part 1 | Algebra Math Hack #shorts #math #school
How much does a PHYSICS RESEARCHER make?
#pov : my gcse results vs what i predicted #gcse #gcseresults #gcse2022 #results #shortsvideo
Human Calculator Solves World’s Longest Math Problem #shorts
Year 12/AS Pure Chapter 10.4 (Trigonometric Identities and Equations)
pov: you worked harder for gcses than alevels. #shorts #student
A-level Mathematics Pure 3 Chapter 4 Trigonometric Addition Formulae IAL
A-level Mathematics Pure 1 Chapter 4 Graphs and Transformations
👀 Asking GCSE Students (Hamdi) How Much They Physics They Know - Part 1 #Shorts
Invading a first year Maths lecture #shorts #tiktokviral #oxforduniversity
A Level Chemistry is EFFORTLESS Once You Learn This
Year 12/AS Pure Chapter 4.2 (Graphs and Transformations)
Composite Functions
Year 12/AS Pure Chapter 10.3 (Trigonometric Identities and Equations)
Year 12/AS Pure Chapter 8.3 (The Binomial Expansion)
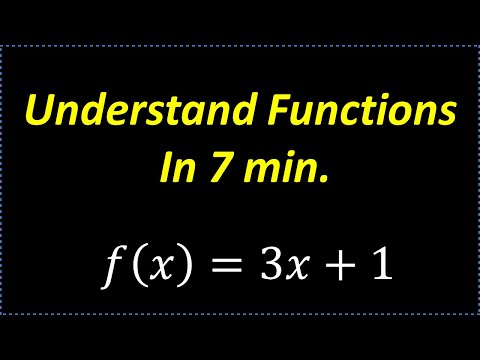
Learn Functions – Understand In 7 Minutes
Year 12/AS Pure Chapter 4.5 (Graphs and Transformations)
Year 12/AS Pure Chapter 9.3 (Trigonometric Ratios)
HOW CHINESE STUDENTS SO FAST IN SOLVING MATH OVER AMERICAN STUDENTS
Google CEO Sundar Pichai Class 12th Marks 😉 | Savage Answer #Shorts
Комментарии
 0:57:46
0:57:46
 0:30:31
0:30:31
 1:11:58
1:11:58
 0:37:55
0:37:55
 0:00:15
0:00:15
 0:00:44
0:00:44
 0:00:16
0:00:16
 0:00:34
0:00:34
 0:30:14
0:30:14
 0:00:10
0:00:10
 2:15:33
2:15:33
 1:29:09
1:29:09
 0:00:37
0:00:37
 0:01:00
0:01:00
 0:05:30
0:05:30
 0:36:04
0:36:04
 0:05:23
0:05:23
 0:36:41
0:36:41
 0:47:06
0:47:06
 0:09:43
0:09:43
 0:54:47
0:54:47
 0:43:50
0:43:50
 0:00:23
0:00:23
 0:01:00
0:01:00