filmov
tv
Olympiad Geometry Problem #46: Circumcenter, Parallel, Orthocenter

Показать описание
Here is a very beautiful problem with a simple starting configuration, that I found on the Art of Problem Solving forum. Please let me know if you find a simpler solution! Link below.
Olympiad Geometry Problem #46: Circumcenter, Parallel, Orthocenter
Olympiad Geometry Problem #39: Circumcenter, Incenter, Double Segment
Olympiad Geometry Problem #83: Tangency, Circumcircle, Bisection
Olympiad Geometry Problem #77: Incenter, Perpendiculars, Parallel
Olympiad Geometry Problem #97: Incircle, Tangency Points, Concurrence
Olympiad Geometry Problem #33: Incenter, Angle Bisector, Midpoint
Olympiad Geometry Problem #22: Perpendiculars, Parallel, Cyclic
Olympiad Geometry Problem #48: Gauss Line
L-11 Properties of Different Centres of Circles | Geometry | IOQM 2025 | Prashant Jain #ioqm
Olympiad Geometry Problem #47: Heron's Formula
Orthocenter| Angle Chasing| The Ultimate Geo Course
Olympiad Geometry Problem #20: Orthocenter, Angle Bisector, Tangent
Olympiad Geometry Problem #17: Blanchett's Theorem
How to find the area of blue circle geometry problem for olympiad @Kamaldheeriya Maths easy
Triangle Incenter, Orthocenter, & Circumcenter Phenomenon 👀
Olympiad Geometry Problem #32: IMO 2019 #6
Finding Angles with Orthocenter and Problem Solving Strategies
Albert Einstein doing physics | very rare video footage #shorts
Olympiad Geometry Problem #96: Spiral Similarities
Steiner Lehmus
Olympiad Geometry Problem #31: Two Angle Bisectors, Equal Angles
Indian National Math Olympiad 2021 Problem 5 - A simple and beautiful geometry problem from the INMO
Most💯 Important Step Before any Procedure 🔥
Prove 3 Points Are Collinear: A Geometry Problem from 2021 China's Math Olympiad
Комментарии
 0:09:22
0:09:22
 0:10:13
0:10:13
 0:12:32
0:12:32
 0:07:58
0:07:58
 0:09:32
0:09:32
 0:09:04
0:09:04
 0:12:37
0:12:37
 0:16:00
0:16:00
 0:46:26
0:46:26
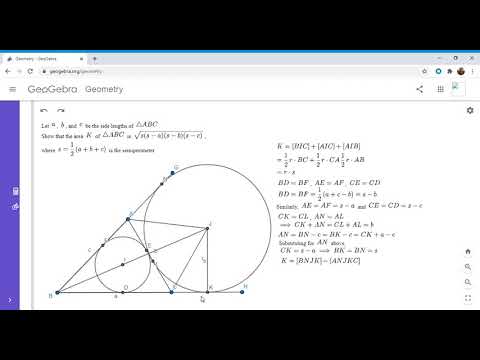 0:16:12
0:16:12
 0:09:33
0:09:33
 0:13:09
0:13:09
 0:11:39
0:11:39
 0:00:46
0:00:46
 0:00:46
0:00:46
 0:20:24
0:20:24
 0:12:02
0:12:02
 0:00:13
0:00:13
 0:12:31
0:12:31
 0:13:46
0:13:46
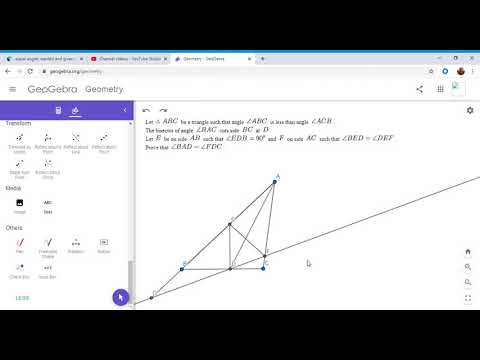 0:08:38
0:08:38
 0:26:31
0:26:31
 0:00:16
0:00:16
 0:14:52
0:14:52