Alexander Efimov - Nuclear modules over proper DG algebras (Part 1)

Alexander Efimov (Steklov Institute for Mathematics); February 18, 2021

I will explain a certain general natural construction of a dualizable presentable DG category Nuc(A) associated with a proper DG algebra (or a proper DG category) A over a commutative ring k. As a special case, it gives (an "unbounded" version of) the category of nuclear modules on a formal scheme, which was defined recently by Clausen and Scholze.
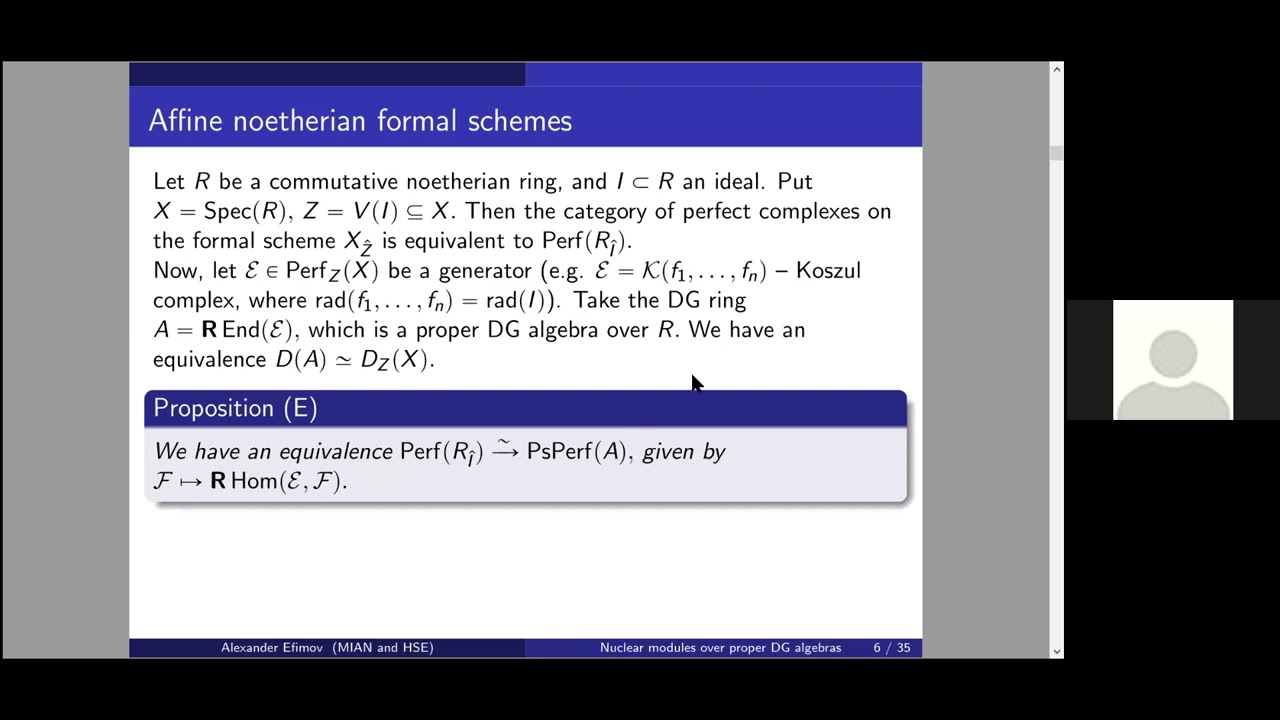
The compact objects of Nuc(A) are given by (the usual) pseudo-perfect A-modules PsPerf(A) (i.e. those A-modules which are perfect over k). However, unlike PsPerf(A), the category Nuc(A) has very nice properties: it satisfies Zariski descent over Spec(k), and so does its continuous K-theory. Moreover, its continuous K-theory and Hochschild homology are expected to have a very concrete description in terms of A.
I will also explain that Nuc(A) is a special case of an even more general notion/construction, which (surprisingly) was not considered before: internal Hom in the symmetric monoidal category, whose objects are dualizable presentable DG categories, and the morphisms are given by strongly continuous functors (i.e. the functors whose right adjoint commutes with infinite direct sums).